TRIANGLE CONGRUENCE POSTULATES AND THEOREMS
Two triangles are said to be congruent, if they have the same shape and same size.
Following are the triangle congruence postulates and theorems :
1. Side - Side - Side (SSS) Congruence Postulate
2. Side - Angle - Side (SAS) Congruence Postulate
3. Angle - Side - Angle (ASA) Congruence Postulate
4. Angle - Angle - Side (AAS) Congruence Postulate
5. Hypotenuse - Leg (HL) Theorem
6. Leg - Acute (LA) Angle Theorem
7. Hypotenuse - Acute (HA) Angle Theorem
8. Leg - Leg (LL) Theorem
Caution :
SSA and AAA can not be used to test congruent triangles.
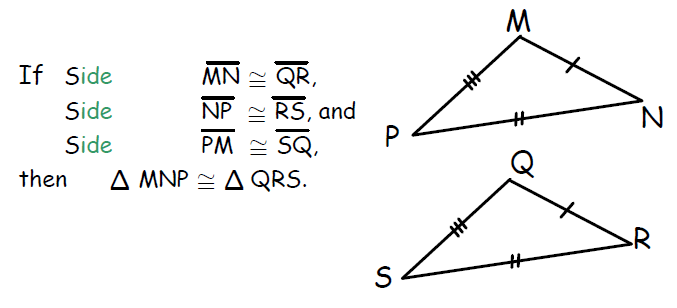
SSS Congruence Postulate
Side-Side-Side (SSS) Congruence Postulate
Explanation :
If three sides of one triangle are congruent to three sides of another triangle, then the two triangles are congruent.
SAS Congruence Postulate
Side-Angle-Side (SAS) Congruence Postulate
Explanation :
If two sides and the included angle of one triangle are equal to two sides and the included angle of another triangle, then the two triangles are congruent.
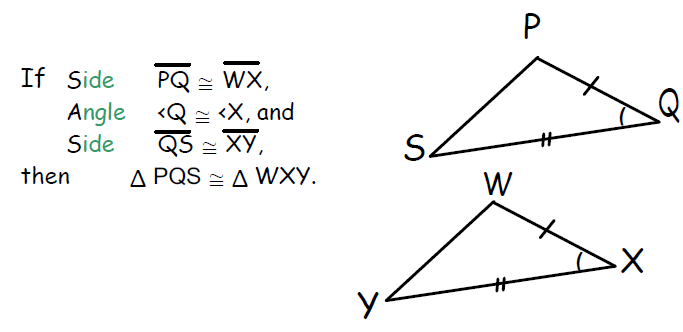
ASA Congruence Postulate
Angle-Side-Angle (ASA) Congruence Postulate
Explanation :
If two angles and the included side of one triangle are equal to two angles and the included side of another triangle, then the two triangles are congruent.
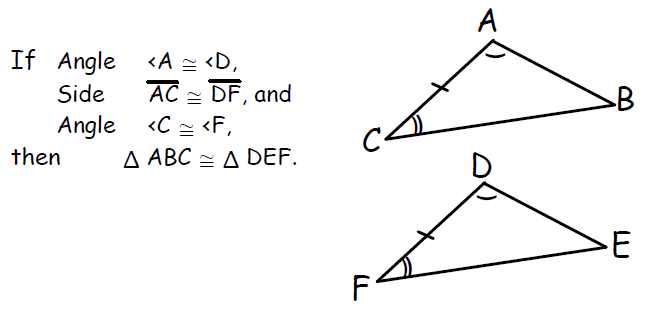
AAS Congruence Postulate
Angle-Angle-Side (AAS) Congruence Postulate
Explanation :
If two angles and non-included side of one triangle are equal to two angles and the corresponding non-included side of another triangle, then the two triangles are congruent.
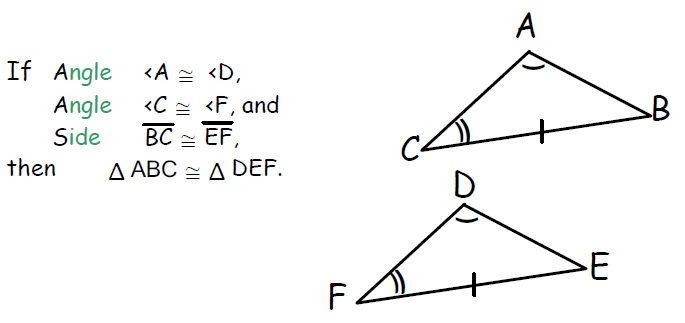
HL Theorem
Hypotenuse-Leg (HL) Theorem
Explanation :
If the hypotenuse and one leg of a right triangle are equal to the hypotenuse and one leg of another right triangle, then the two right triangles are congruent.
This principle is known as Hypotenuse-Leg theorem.
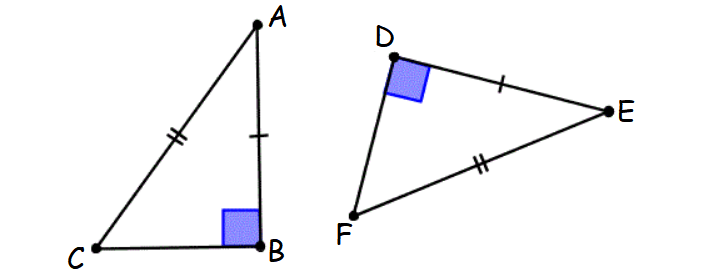
LA Angle Theorem
Leg-Acute (LA) Angle Theorem
Explanation :
If a leg and an acute angle of one right triangle are congruent to the corresponding parts of another right triangle, then the two right triangles are congruent.
This principle is known as Leg-Acute Angle theorem.
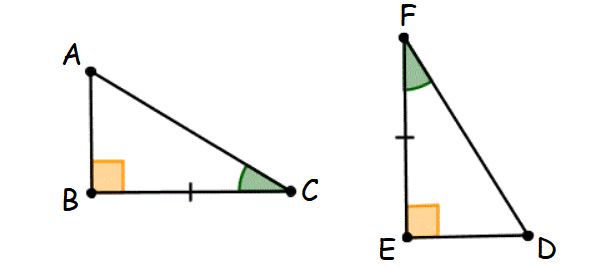
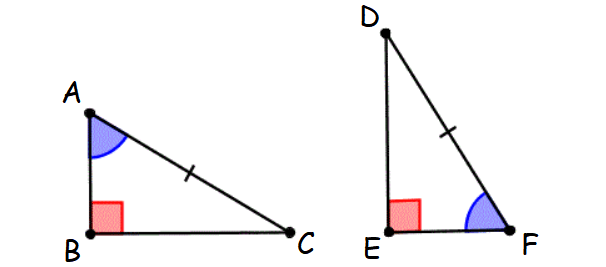
HA Angle Theorem
Hypotenuse-Acute (HA) Angle Theorem
Explanation :
If the hypotenuse and an acute angle of a right triangle are congruent to the hypotenuse and an acute angle of another right triangle, then the two triangles are congruent.
This principle is known as Hypotenuse-Acute Angle theorem.
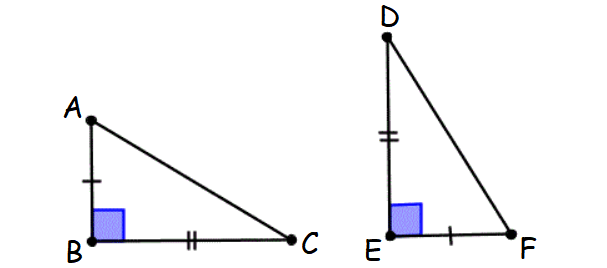
LL Theorem
Leg-Leg (LL) Theorem
Explanation :
If the legs of one right triangle are congruent to the legs of another right triangle, then the two right triangles are congruent.
This principle is known as Leg-Leg theorem.
Solved Problems
Problem 1 :
In the diagram given below, prove that ΔPQW ≅ ΔTSW.
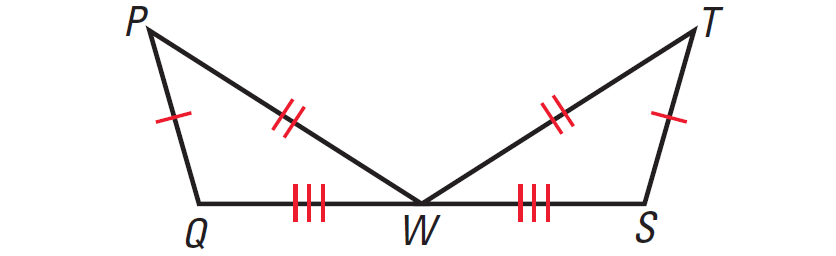
Solution :
|
Statements PQ ≅ ST PW ≅ TW QW ≅ SW ΔPQW ≅ ΔTSW |
Reasons Given Given Given SSS Congruence Postulate |
Problem 2 :
In the diagram given below, prove that ΔABC ≅ ΔFGH.
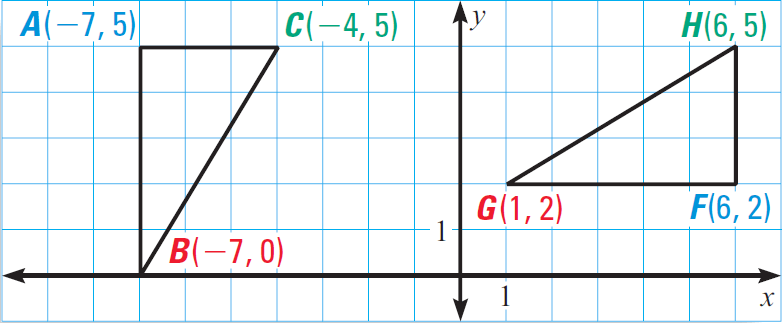
Solution :
Because AB = 5 in triangle ABC and FG = 5 in triangle FGH,
AB ≅ FG
Because AC = 3 in triangle ABC and FH = 3 in triangle FGH,
AC ≅ FH
Use the distance formula to find the lengths of BC and GH.
Length of BC :
BC = √[(x2 - x1)2 + (y2 - y1)2]
Substitute (x1, y1) = B(-7, 0) and (x2, y2) = C(-4, 5).
BC = √[(-4 + 7)2 + (5 - 0)2]
= √[32 + 52]
= √[9 + 25]
= √34
Length of GH :
GH = √[(x2 - x1)2 + (y2 - y1)2]
Substitute (x1, y1) = B(1, 2) and (x2, y2) = C(6, 5).
GH = √[(6 - 1)2 + (5 - 2)2]
= √[52 + 32]
= √[25 + 9]
= √34
Conclusion :
Because BC = √34 and GH = √34,
BC ≅ GH
All the three pairs of corresponding sides are congruent. By SSS congruence postulate,
ΔABC ≅ ΔFGH
Problem 3 :
In the diagram given below, prove that ΔAEB ≅ ΔDEC.
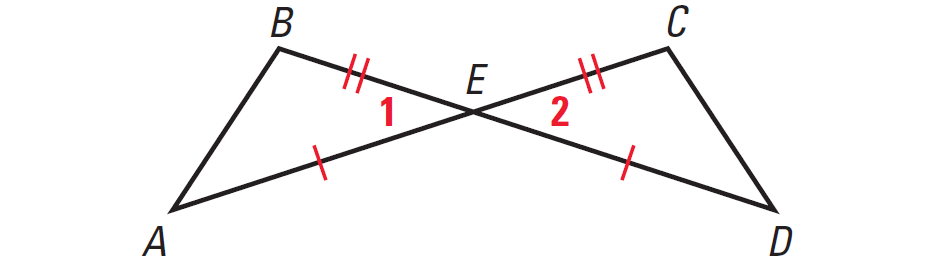
Solution :
|
Statements AE ≅ DE, BE ≅ CE ∠1 ≅ ∠2 ΔAEB ≅ ΔDEC |
Reasons Given Vertical Angles Theorem SAS Congruence Postulate |
Problem 4 :
In the diagram given below, prove that ΔABD ≅ ΔEBC.
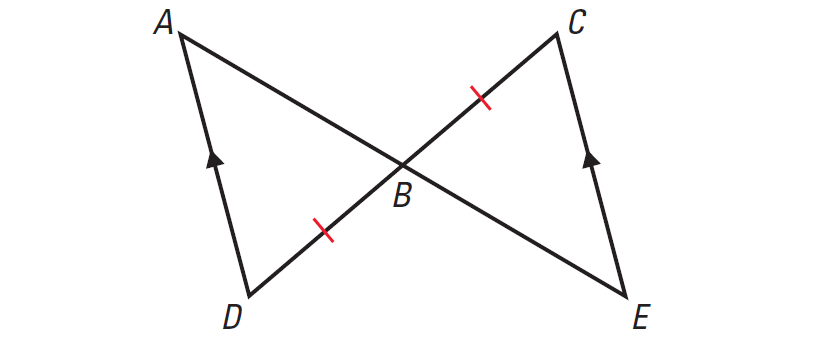
|
Statements BD ≅ BC AD || EC ∠D ≅ ∠C ∠ABD ≅ ∠EBC ΔABD ≅ ΔEBC |
Reasons Given Given Alternate Interior Angles Theorem Vertical Angles Theorem ASA Congruence Postulate |
Problem 5 :
In the diagram given below, prove that ΔEFG ≅ ΔJHG.
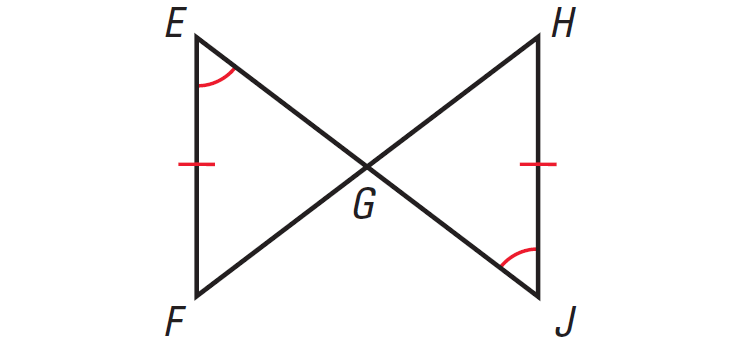
Solution :
|
Statements FE ≅ JH ∠E ≅ ∠J ∠EGF ≅ ∠JGH ΔEFG ≅ ΔJHG |
Reasons Given Given Vertical Angles Theorem AAS Congruence Postulate |
Kindly mail your feedback to v4formath@gmail.com
We always appreciate your feedback.
©All rights reserved. onlinemath4all.com
Recent Articles
-
SAT Math Questions and Answers
Oct 13, 25 01:09 PM
SAT Math Questions and Answers -
10 Hard SAT Math Questions (Part - 27)
Oct 13, 25 11:55 AM
10 Hard SAT Math Questions (Part - 27) -
10 Hard SAT Math Questions (Part - 26)
Oct 10, 25 08:14 PM
10 Hard SAT Math Questions (Part - 26)
