TRIANGLE WORKSHEET SOLUTION 5

(5) If the three points (h, 0) (a, b) and (0, k) lie on a straight line, then using the area of the triangle formula show that (a/h) + (b/k) = 1,where h, k ≠ 0

Solution :

Let A(h, 0) B (a, b) and C (0, k) are the three points

Since the three points A (h, 0) B (a, b) and C (0, k) lie on a straight line we can say that the three points are collinear

So , area of triangle ABC = 0

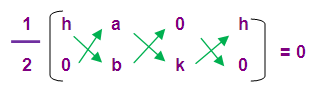
(1/2) [ (h b + a k + 0) – (0 + 0 + kh) ] = 0

[ h b + a k  – k h ] = 0 x 2

h b + a k - k h = 0

h b + a k  = k h

Divided (k h) on both sides,

(h b)/(k h) + (a k)/(k h)  = (k h)/(k h)

(b/k) + (a/h) = 1

(a/h) + (b/k) = 1

(6) Find the area of the triangle formed by joining the midpoints of the sides of a triangle whose vertices are (0, -1) (2, 1) and (0, 3). Find the ratio of this area to the area of the given triangle.

Solution :

Let A (0,-1) B (2,1) and C (0,3) are the vertices of the triangle. D,E and F are the midpoints of the side AB,BC and CA respectively.

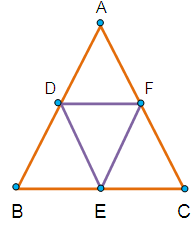
Midpoint of AB  =  (x₁ + x₂)/2 , (y₁ + y₂)/2

  =  (0 + 2)/2 , (-1 + 1)/2

  =  2/2 , 0/2

  =  D (1,0)

Midpoint of BC  =  (x₁ + x₂)/2 , (y₁ + y₂)/2

  =  (2 + 0)/2 , (1 + 3)/2

  =  2/2 , 4/2

  =  E (1,2)    

Midpoint of CA  =  (x1+x2)/2 , (y1+y2)/2

  =  (0 + 0)/2 , (3 + (-1))/2

  =  0/2 , 2/2

  =  F (0,1)    

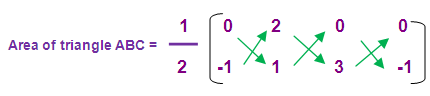
  =  (1/2) [(0 + 6 + 0) – (-2 + 0 + 0)]

  =  (1/2) [6+2]

  =  (1/2) [8]

  =  4 square units

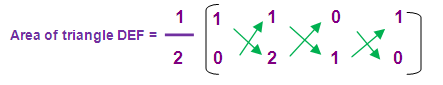
  =  (1/2) [(2 + 1 + 0) – (0 + 0 + 1)]

  =  (1/2) [3-1]

  =  (1/2) [2]

  =  1 square units

Area of triangle ABC: Area of triangle DEF

4 : 1

Apart from the stuff given above, if you need any other stuff in math, please use our google custom search here.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.