TRIGONOMETRIC RATIOS OF 180 DEGREE MINUS THETA

Trigonometric ratios of 180 degree minus theta is one of the branches of ASTC formula in trigonometry. 

Trigonometric-ratios of 180 degree minus theta are given below.

sin (180° - θ)  =  sin θ

cos (180° - θ)  =  - cos θ

tan (180° - θ)  =  - tan θ

csc (180° - θ)  =  csc θ

sec (180° - θ)  =  - sec θ

cot (180° - θ)  =  - cot θ

Let us see, how the trigonometric ratios of 180 degree minus theta are determined. 

To know that, first we have to understand ASTC formula. 

The ASTC formula can be remembered easily using the following phrases.

"All Sliver Tea Cups" 

or

"All Students Take Calculus"

ASTC formula has been explained clearly in the figure given below.

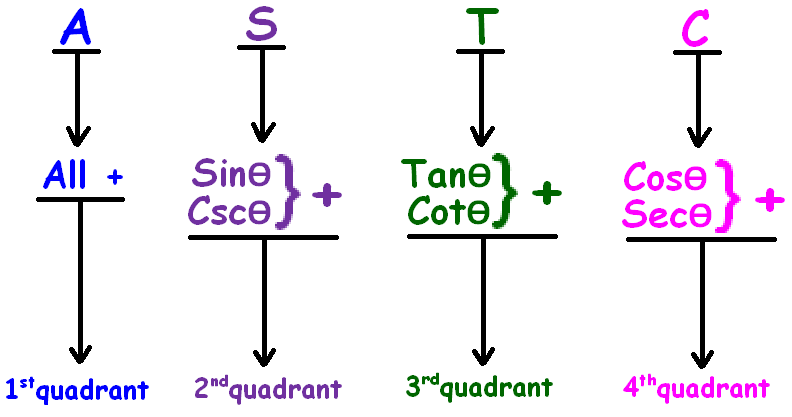
More clearly 

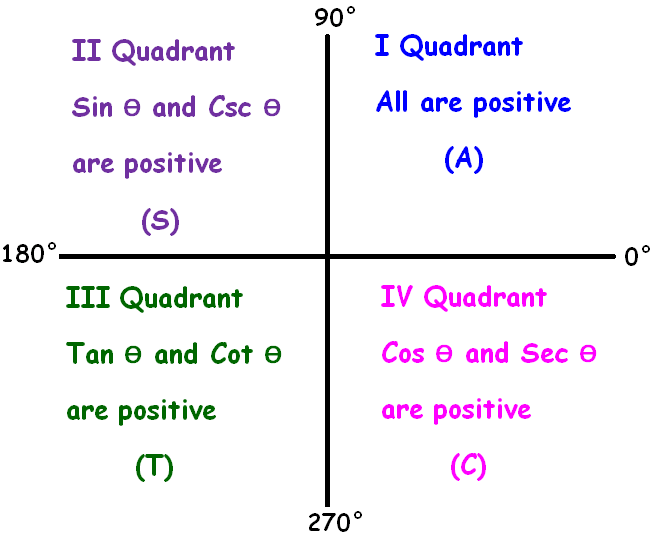
From the above picture, it is very clear that 

(180° - θ) falls in the second quadrant 

In the third quadrant (180° + θ), tan and cot are positive and other trigonometric ratios are negative.

Important Conversions

When we have the angles 90° and 270° in the trigonometric ratios in the form of

(90° + θ)

(90° - θ)

(270° + θ)

(270° - θ)

We have to do the following conversions, 

sin θ <------> cos θ

tan θ <------> cot θ

csc θ <------> sec θ

For example,

sin (270° + θ)  =  - cos θ

cos (90° - θ)  =  sin θ

For the angles 0° or 360° and  180°, we should not make the above conversions. 

Evaluation of Trigonometric Ratios of 180 Degree Minus Theta

Problem 1 :

Evaluate :

sin (180° - θ)

Solution :

To evaluate sin (180° - θ), we have to consider the following important points. 

(i)  (180° - θ) will fall in the II^nd quadrant. 

(ii)  When we have 180°, "sin" will not be changed as "cos"

(iii)  In the II^nd quadrant, the sign of "sin" is positive. 

Considering the above points, we have 

sin (180° - θ)  =  sin θ

Problem 2 :

Evaluate :

cos (180° - θ)

Solution :

To evaluate cos (180° - θ), we have to consider the following important points. 

(i)  (180° - θ) will fall in the II^nd quadrant. 

(ii)  When we have 180°, "cos" will not be changed as "sin"

(iii)  In the II^nd quadrant, the sign of "cos" is negative. 

Considering the above points, we have 

cos (180° - θ)  =  - cos θ

Problem 3 :

Evaluate :

tan (180° - θ)

Solution :

To evaluate tan (180° - θ), we have to consider the following important points. 

(i)  (180° - θ) will fall in the II^nd quadrant. 

(ii)  When we have 180°, "tan" will not be changed as "cot"

(iii)  In the II^nd quadrant, the sign of "tan" is negative. 

Considering the above points, we have 

tan (180° - θ)  =  - tan θ

Problem 4 :

Evaluate :

csc (180° - θ)

Solution :

To evaluate csc (180° - θ), we have to consider the following important points. 

(i)  (180° - θ) will fall in the II^nd quadrant. 

(ii)  When we have 180°, "csc" will not be changed as "sec"

(iii)  In the II^nd quadrant, the sign of "csc" is positive. 

Considering the above points, we have 

csc (180° - θ)  =  csc θ

Problem 6 :

Evaluate :

cot (180° - θ)

Solution :

To evaluate cot (180° - θ), we have to consider the following important points. 

(i)  (180° - θ) will fall in the II^nd quadrant. 

(ii)  When we have 180°, "cot" will not be changed as "tan"

(iii)  In the II^nd quadrant, the sign of "cot" is negative. 

Considering the above points, we have 

cot (180° - θ)  =  - cot θ

Summary (180 Degree Minus Theta)

sin (180° - θ)  =  sin θ

cos (180° - θ)  =  - cos θ

tan (180° - θ)  =  - tan θ

csc (180° - θ)  =  csc θ

sec (180° - θ)  =  - sec θ

cot (180° - θ)  =  - cot θ

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.