TRIGONOMETRY PROBLEMS WITH SOLUTIONS FOR CLASS 11

Problem 1 :

Show that

cot(7½°) =   √2 + √3 + √4 + √6

Solution :

cot(7½°) :

=  cos(7½°) / sin(7½°)

Multiply both numerator and denominator by 2sin(7½°).

=  [cos(7½°) ⋅ 2sin(7½°)] / [sin(7½°) ⋅ sin(7½°)]

=  [2sin(7½°)cos(7½°)] / sin²(7½°)

=  sin(2 ⋅ 7½°) / [1 - cos(2 ⋅ 7½°)]

=  sin15° / (1 - cos15°) -----(1)

sin15°  =  sin(45° - 30°)  

=  sin45°cos30° - cos45°sin30°

=  (1/√2) (√3/2) - (1/√2) (1/2)

=  (√3/2√2) - (1/2√2)

=  (√3 - 1)/2√2 -----(2)

cos15°  =  cos(45° - 30°)  

=  cos45°cos30° + sin45°sin30°

=  (1/√2) (√3/2) + (1/√2) (1/2)

=  (√3/2√2) + (1/2√2)

=  (√3 + 1)/2√2

1 - cos15° :

=  1 - [(√3 + 1)/2√2]

=  1 - (√3 + 1)/2√2]

=  [2√2 - (√3 + 1)] / 2√2 -----(2)

(1)----->

=  (2)/(3)

  =  (√3 - 1)/2√2 / {[2√2 - (√3 + 1)]/2√2}

  =  (√3 - 1) / [2√2 - (√3 + 1)]

Multiply both numerator and denominator by [2√2+(√3+1)].

=  (√3 - 1)[2√2 + (√3 + 1)] / [4(2) - (√3 + 1)²]

=  (√3 - 1)[2√2 + √3 + 1] / [4(2) - (√3 + 1)²]

=  (2√6 + 3 + √3 - 2√2 - √3 - 1)/ [8 - (3 + 1 + 2√3)]

=  (2√6 + 2 - 2√2) / [4 - 2√3]

=  (√6 + 1 -  √2) / [2 - √3]

Multiply both numerator and denominator by (2 + √3).

=  (√6 + 1 -  √2) (2 + √3) / (2 - √3)(2 + √3)

=  (2√6 + 2 - 2√2 + 3√2 + √3 - √6)/(4 - 3)

=  √6 + √2 + 2 + √3 / 1

=  √6 + √2 + 2 + √3

=  √2 + √3 + √4 + √6

Hence proved. 

Question 2 :

Prove that

(1 + sec2θ)(1 + sec4θ)..........(1 + sec2ⁿθ)  =  tan2ⁿθcotθ 

Solution :

L.H.S

(1 + sec2θ)(1 + sec4θ).............(1 + sec2ⁿθ)

=  (1 + cos2θ)(1 + cos4θ).............(1 + cos2ⁿθ)/(cos2θ cos4θ ......cos2ⁿθ)

=  (2cos²θ)(2cos²2θ)(2cos²4θ) ...........(2cos²2^n-1θ) / (cos2θ cos4θ ......cos2ⁿθ)

=  (2cos²θ) (2cos2θ)(2cos4θ) ...........(2cos²2^n-1θ)/(cos2ⁿθ)

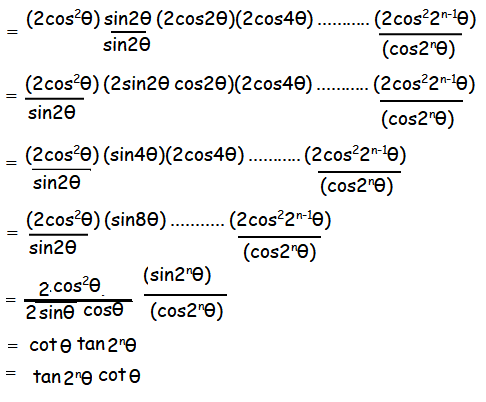
Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.