UNIQUE SOLUTION NO SOLUTION INFINITE SOLUTIONS

Consider the following system of linear equations given in slope-intercept form.

y = m₁x + b₁

(slope = m₁ and y-intercept = b₁)

y = m₂x + b₂

(slope = m₂ and y-intercept = b₂)

Unique Solution

y = m₁x + b₁

y = m₂x + b₂

If the above system of linear equations has unique solution or only one solution, then it has to satisfy the following condition.

m₁ ≠ m₂

That is, the slopes must not be equal.

We already know that the graph of any linear equation will be a line.

If two lines have different slopes (m₁ ≠ m₂), then the lines intersect in only one point and the system has unique solution or only one solution.

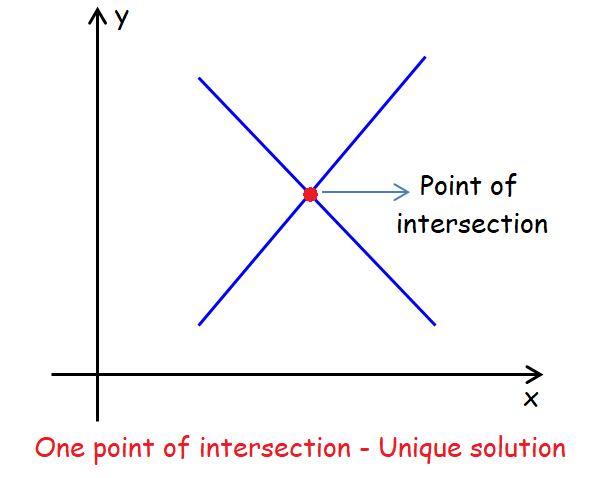
In a system of equations with two variables, let the slopes be not equal (m₁ ≠ m₂).

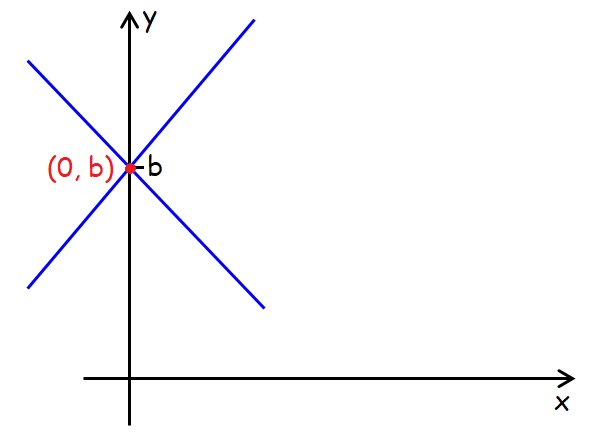
What if there is same y-intercept, say 'b'?

In a system of two linear equations, if the slopes are not equal (m₁ ≠ m₂), then the system has unique solution or only one solution. It does not matter whether there is same y-intercept or different y-intercepts.

If there is same y-intercept, say 'b', the unique solution is

(0, b)

No Solution

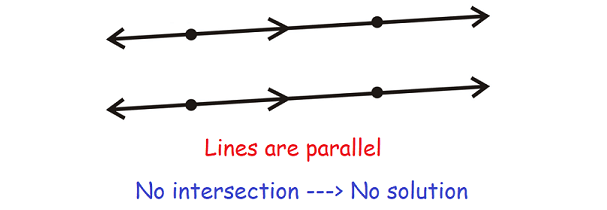
If the above system of linear equations does not have solution, then it has to satisfy the following conditions.

m₁ = m₂

b₁ ≠ b₂

If two lines have the same slope (m₁ = m₂) and different y-intercepts (b₁ ≠ b₂), then the two lines are parallel and they will never intersect. 

So, no solution for the system of linear equations with the same slope and different y-intercept.

Infinite Solutions

Two linear equations in a system are exactly the same, then the system has infinitely many solution.

Consider the following system of equations.

3x - y + 5 = 0 ----(1)

15x - 5y + 25 = 0 ----(2)

Divide both sides of the equation (2) by 5.

(2) ÷ 5 ----> 15x/5 - 5y/5 + 25/5 = 0/5

3x - y + 5 = 0

When you divide the equation (2) by 5, you get the equation (1).

So, the two equations given in the above system are exactly same. Hence the system has infinitely many solutions.

The other way to check whether a system of linear equations in two variables has infinitely many solutions is writing the equations in slope intercept form.

Consider the following system of equations given in slope-intercept form.

y = m₁x + b₁

(slope = m₁ and y-intercept = b₁)

y = m₂x + b₂

(slope = m₂ and y-intercept = b₂)

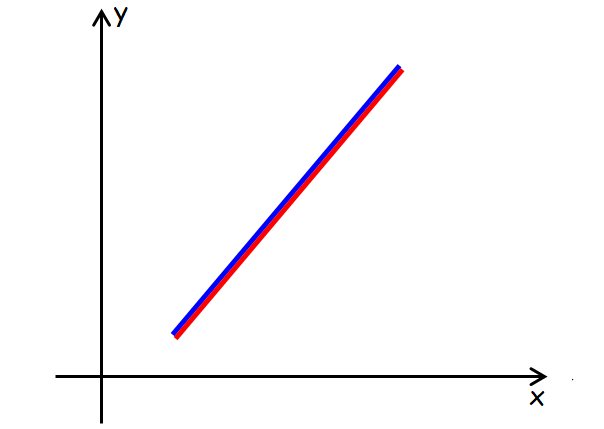
If the above system of equations has infinitely many solutions, then it has to satisfy the following two conditions.

m₁ = m₂

b₁ = b₂

The lines are same and hence they coincide.

Since the lines coincide, they touch each other in all the points on both the lines and the system has infinitely many solutions.

Examples 1-2 : Determine whether the following systems of linear equations have unique solution.

Example 1 :

y = 2x + 5

y = 3x - 2

Solution :

y = 2x + 5 ----> slope  m = 2

y = 3x - 2 ----> slope m = 3

In the above two linear equations, the slopes are different.

So, the lines intersect in only one point.

Hence, the system has unique solution.

Example 2 :

y = -2x + 5

y = -2x + 3

Solution :

y = -2x + 5 ----> slope m = -2

y = -2x + 1 ----> slope m = -2

In the above two linear equations, slopes are equal.

Hence, the system has no unique solution.

Examples 3-4 : Determine whether the following systems of linear equations have no solution.

Example 3 :

y = 3x + 5

y = 3x - 2

Solution :

y = 3x + 5 ----> m = 3 and b = 5

y = 3x - 2 ----> m = 3 and b = -2

In the above two linear equations, the slope is same and y-intercepts are different.

So, the lines are parallel and they never intersect.

Hence, the system has no solution.

Example 4 :

4x + 2y - 1 = 0

2x + y + 5 = 0

Solution :

The equations are not in slope-intercept form.

Write them in slope-intercept form.

y = -2x + 1/2 ----> m = -2 and b = 1/2

y = -2x - 5 ----> m = -2 and b = -5

In the given two linear equations, the slope is same and y-intercepts are different.

So, the lines are parallel and they never intersect.

Hence, the system has no solution.

Examples 5-7 : Determine whether the following systems of linear equations have infinitely many solutions.

Example 5 :

y = 3x + 5

y = 3x + 5

Solution :

y = 3x + 5 ----> m = 3 and b = 5

y = 3x + 5 ----> m = 3 and b = 5

In the above two linear equations, both the slopes and y-intercepts are same.

So, the lines coincide and they  touch each other in all the points on the line.

Hence, the system has infinitely many solution.

Example 6 :

4x + 2y - 1 = 0

2x + y - 0.5 = 0

Solution :

The equations are not in slope-intercept form.

Write them in slope-intercept form.

y = -2x + 1/2 ----> m = -2 and b = 1/2

y = -2x + 1/2 ----> m = -2 and b = 1/2

In the given two linear equations, both the slopes and y-intercepts are same.

So, the lines coincide and they  touch each other in all the points on the line.

Hence, the system has infinitely many solution.

Example 7 :

2x - y = 1

4x + y = 5

Solution :

The equations are not in slope-intercept form.

Write them in slope-intercept form.

In the given two linear equations, the slope are different.

So, the lines do not coincide.

Hence, the system does not have infinitely many solutions.

Example 8 :

In the following system of linear equations, k is a constant and x and y are variables. For what value of k will the system of equations have unique solution?

kx - y = 4

10x - 5y = 7

Solution :

The equations are not in slope-intercept form.

Write them in slope-intercept form.

y = kx - 4/3 ----> slope m = k

y = 2x - 7/5 ----> slope m = 2

If the system has unique solution, then the slopes must not be equal.

k ≠ 2

When k ≠ 2, the system has unique solution.

Example 9 :

In the following system of linear equations, k is a constant and x and y are variables. For what value of k will the system of equations have no solution?

kx - 3y = 4

4x - 5y = 7

Solution :

The equations are not in slope-intercept form.

Write them in slope-intercept form.

y = (k/3)x - 4/3 ----> m = k/3 and b = -4/3

y = (4/5)x - 7/5 ----> m = 4/5 and b = -7/5

In the given two linear equations, y-intercepts are different.

If slopes are equal, then the lines will be parallel and they will never intersect. And also, the system will not have solution.

It is given that the system has no solution.

So, the slopes must be equal.

k/3 = 4/5

Multiply both sides by 3.

k = 12/5

When k = 12/5, the system will have no solution.

Example 10 :

In the following system of linear equations, k is a constant and x and y are variables. For what value of k will the system of equations have infinitely many solution?

kx - 3y = 12

4x - 5y = 20

Solution :

The equations are not in slope-intercept form.

Write them in slope-intercept form.

y = (k/3)x - 4 ----> m = k/3 and b = -4

y = (4/5)x - 4 ----> m = 4/5 and b = -4

In the given two linear equations, y-intercepts are equal.

If slopes also are equal, then the lines will coincide and the system will have infinitely many solutions.

It is given that the system has infinitely many solutions.

So, the slopes must be equal.

k/3 = 4/5

Multiply both sides by 3.

k = 12/5

When k = 12/5, the system will have infinitely many solutions.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.