USE MODELS TO FIND AREAS OF COMPOSITE SHAPES

Example 1 : 

Find the area of the shaded region.

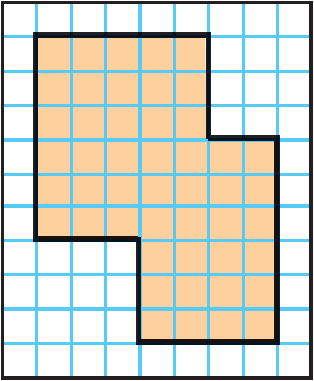
Solution :

One way : 

Count the square units. The shape shows that there are 48 square units.

Another Way :

Step 1 :

Decompose the shape into rectangles and squares.

The shape can be broken into two rectangles and one square as given below.

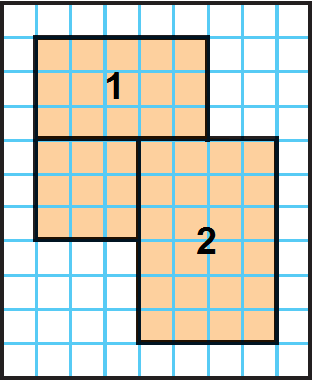
Step 2 :

Find the area of the parts. Then add them together.

Area of rectangle 1 :  3 × 5 = 15

Area of square  :  3 × 3 = 9

Area of rectangle 2 :  4 × 6 = 24

Area of the given composite shape is

=  15 + 24 + 9 

=  48 square units

Example 2 : 

Find the area of the shaded region.

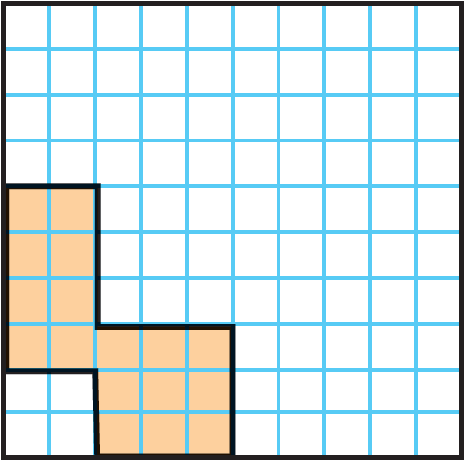
Solution :

Step 1 :

Decompose the shape into parts.

The shape can be broken into one rectangle and one square as given below. 

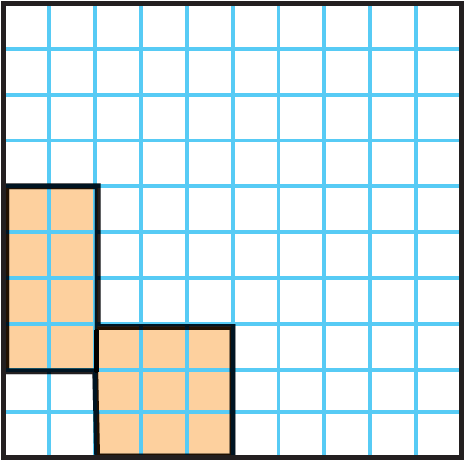
Step 2 :

Find the area of the parts. Then add them together.

Area of rectangle  :  2 × 4 = 8

Area of square  :  3 × 3 = 9

Area of the given composite shape is

=  8 + 9 

=  17 square units

Example 3 : 

Find the area of the shaded region.

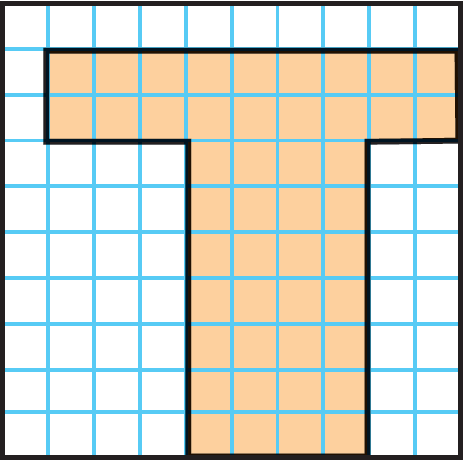
Solution :

Step 1 :

Decompose the shape into parts.

The shape can be broken into two rectangles as given below. 

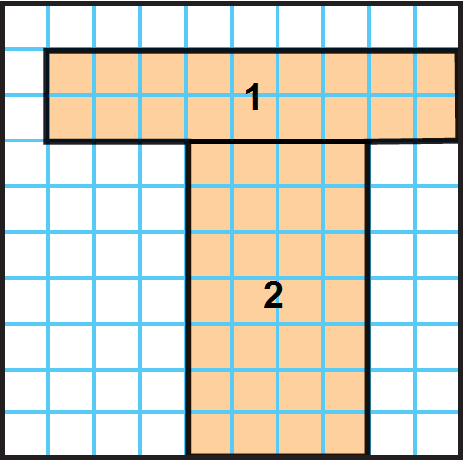
Step 2 :

Find the area of the parts. Then add them together.

Area of rectangle 1  :  9 × 2 = 18 

Area of rectangle 2  :  4 × 7 = 28

Area of the given composite shape is

=  18 + 28 

=  46 square units

Example 4 : 

Find the area of the shaded region.

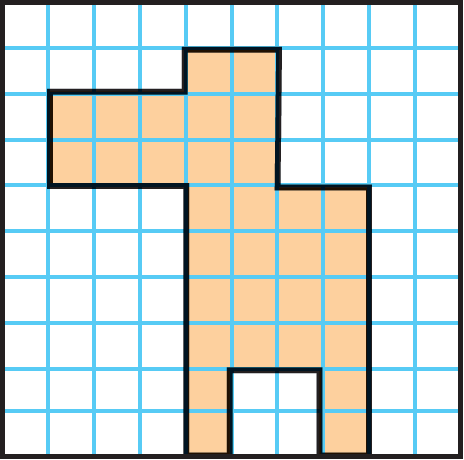
Solution :

Step 1 :

Decompose the shape into parts.

The shape can be broken into four rectangles and one square as given below. 

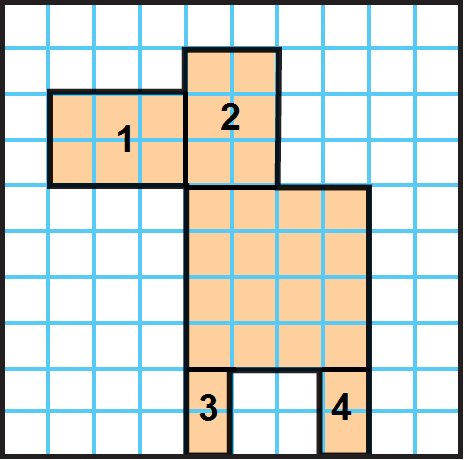
Step 2 :

Find the area of the parts. Then add them together.

Area of rectangle 1  :  3 × 2 = 6 

Area of rectangle 2  :  2 × 3 = 6

Area of square  :  4 × 4 = 16

Area of rectangle 3  :  1 × 2 = 2

Area of rectangle 4  :  1 × 2 = 2

Area of the given composite shape is

=  6 + 6 + 16 + 2 + 2 

=  32 square units

Example 5 : 

Find the area of the shaded region.

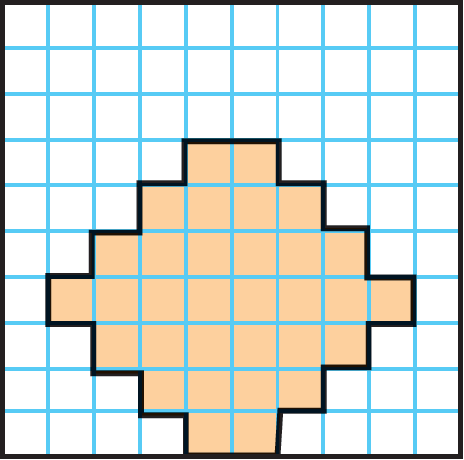
Solution :

Count the square units inside the shaded region. The shape shows that there are 32 square units.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.