USE THE CONVERSE OF THE PYTHAGOREAN THEOREM WORKSHEET

Problem 1 :

Tanya is buying edging for a triangular flower garden she plans to build in her backyard. If the lengths of the three pieces of edging that she purchases are 13 feet, 10 feet, and 7 feet, will the flower garden be in the shape of a right triangle ?

Problem 2 :

A blueprint for a new triangular playground shows that the sides measure 480 ft, 140 ft, and 500 ft. Is the playground in the shape of a right triangle ? Explain.

Problem 3 :

A triangular piece of glass has sides that measure 18 in., 19 in., and 25 in. Is the piece of glass in the shape of a right triangle ? Explain.

Problem 4 :

A corner of a fenced yard forms a right angle. Can we place a 12 ft long board across the corner to form a right triangle for which the leg lengths are whole numbers ? Explain.

Answers

1. Answer :

Step 1 :

Let a = 10, b = 7, and c = 13.

(Always assume the longest side as 'c')

Step 2 :

Find the value of (a² + b²). 

a² + b²  =  10² + 7²

a² + b²  =  100 + 49

a² + b²  =  149 -----(1)

Step 3 :

Find the value of c². 

c²  =  13²

c²  =  169 ------(2)

Step 4 :

From (1) and (2), we get

a² + b²  ≠  c²

By the converse of Pythagorean theorem, the triangle with the side lengths 13 feet, 10 feet, and 7 feet is not a right triangle. 

Hence, the garden will not be in the shape of a right triangle.

2. Answer :

Step 1 :

Let a = 480, b = 140, and c = 500.

(Always assume the longest side as 'c')

Step 2 :

Find the value of (a² + b²). 

a² + b²  =  480² + 140²

a² + b²  =  230,400 + 19,600

a² + b²  =  250,000 -----(1)

Step 3 :

Find the value of c². 

c²  =  500²

c²  =  250,000 -----(2)

Step 4 :

From (1) and (2), we get

a² + b²  =  c²

By the converse of Pythagorean theorem, the triangle with the side lengths 480 ft, 140 ft, and 500 ft is a right triangle. 

Hence, the the playground is in the shape of a right triangle.

3. Answer :

Step 1 :

Let a = 18, b = 19, and c = 25.

(Always assume the longest side as 'c')

Step 2 :

Find the value of (a² + b²). 

a² + b²  =  18² + 19²

a² + b²  =  324 + 361

a² + b²  =  685 -----(1)

Step 3 :

Find the value of c². 

c²  =  25²

c²  =  625 -----(2)

Step 4 :

From (1) and (2), we get

a² + b²  ≠  c²

By the converse of Pythagorean theorem, the triangle with the side lengths 18 in., 19 in., and 25 in. is not a right triangle. 

Hence, the piece of glass is not in the shape of a right triangle.

4. Answer :

Step 1 :

Let a and b be the legs of the triangle.   

Step 2 :

Draw an appropriate diagram for the given information. 

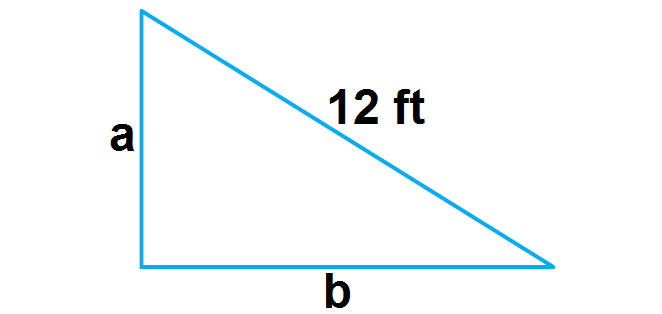
Step 3 :

To form a right triangle, the legs a and b and the length of the board 12 ft must satisfy the converse of the Pythagorean theorem. That is

a² + b²  =  12²

a² + b²  =  144

But, there are no pairs of whole numbers whose squares add up to  12²  =  144.

Hence, we can not place a 12 foot long board across the corner to form a right triangle for which the leg lengths are whole numbers.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.