USING TRANSFORMATIONS TO GRAPH FUNCTIONS OF THE FORM y = af[k(x-d)] + c

In mathematics, we will have situation to graph a function from the parent function using transformation.

Let us see, how to graph the functions which are in the form 

y  =  af[k(x-d)] + c 

using transformation with an example.

Example : 

Sketch the graph of the function given below.

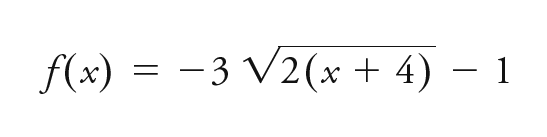
Solution :

The function is a transformed square root function.

So, the parent function is  y  =  √x.

Let us look at each part of the function and write down all the transformations which we need to apply.

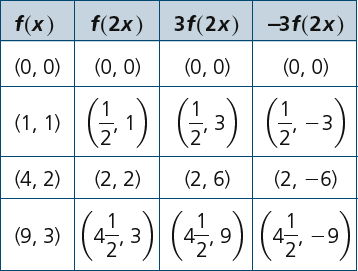
First, let us divide the x- coordinates of points on  y = √x by 2 to compress the graph horizontally by the factor 1/2. 

When we do as said above, we will get the following table of values and graph. 

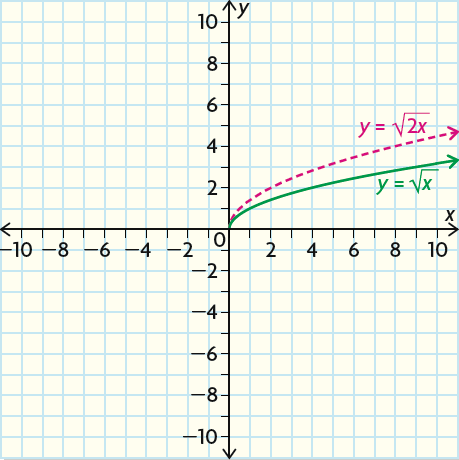
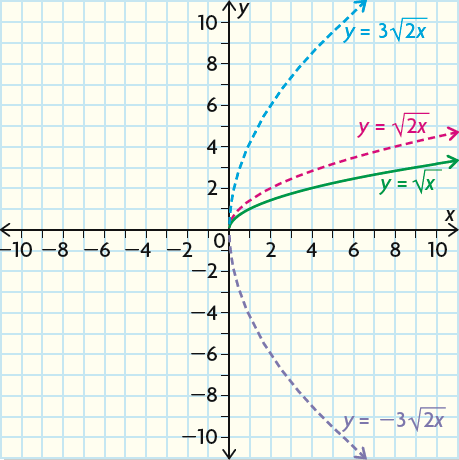
Let us multiply the y-coordinates of y = √(2x) by 3 to stretch the graph vertically by the factor 3.

When we do as said above, we will get the following table of values and graph. 

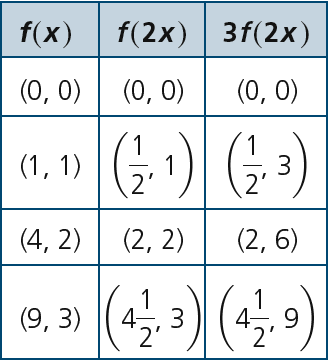
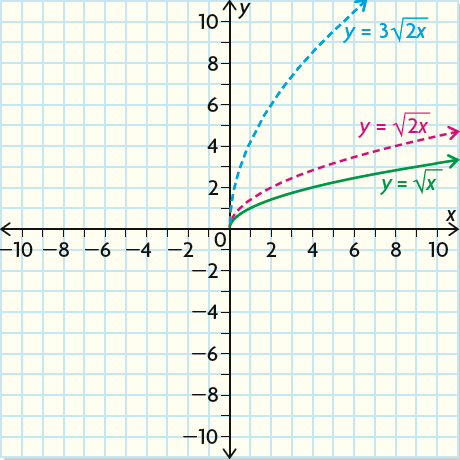
Let us flip the graph of y = 3√(2x) over the x-axis.

(Because, we have negative sign in  front of 3 in the given function)

When we do as said above, we will get the following table of values and graph. 

I did both shifts together. I subtracted 4 from each of the x-coordinates and subtracted 1 from each of the y-coordinates of the graph of y = -3√(2x).

When we do as said above, we will get the following table of values and graph. 

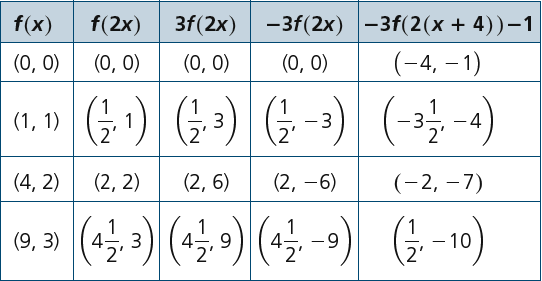
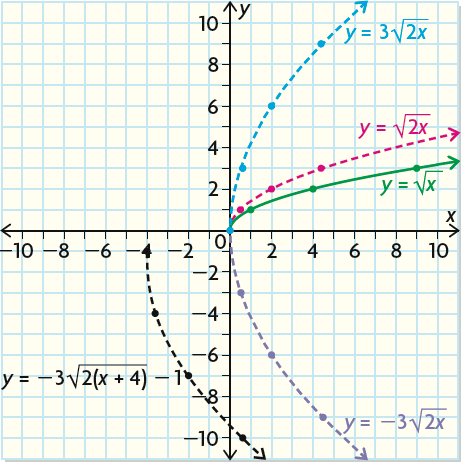
Translate the graph of y = -3√(2x), 4 units left and 1 unit down in order to get the graph of the given function 

y = -3[√(2x+4)] - 1

The graph of the given function y = -3[√(2x+4)] - 1 is given in black color.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.