VERTEX FORM OF A QUADRATIC EQUATION

Learning Objectives :

* Vertex form of a quadratic equation.

* If a quadratic equation is given in standard form, how to write it in vertex form.

* How to sketch the graph of a quadratic equation that is in vertex form.

Vertex form of a quadratic equation :

y = a(x - h)² + k

where (h, k) is the vertex of the parabola. 

The h represents the horizontal shift and k represents the vertical shift. 

Horizontal Shift :

Shifting the graph of the parent function y = x² to the left or right from x = 0. 

Vertical Shift :

Shifting the graph of the parent function y = x² up or down from x = 0. 

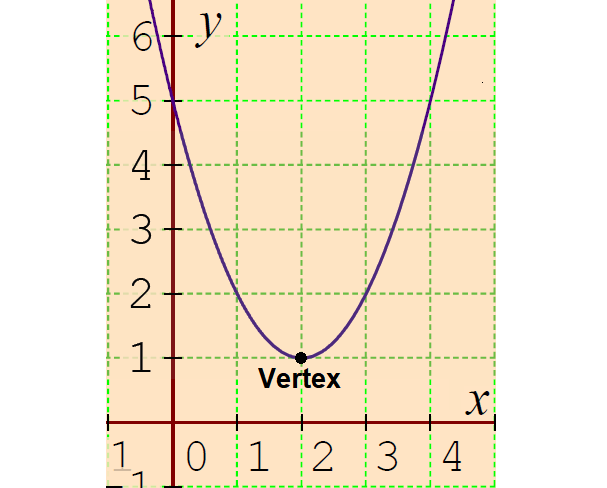
In the above picture, the graph (Parabola) of the parent function y = x² is shifted 2 units to the right from x = 0 and 1 unit up from y = 0. 

So, the vertex is

(Horizontal shift, Vertical shift) = (2, 1)

Example 1 :

Write the quadratic equation in vertex form and write its vertex : 

y = -x² - 2x - 2

Solution :

Vertex form of the quadratic equation :

y = -x² - 2x - 2

y = -(x² + 2x) - 2

y = -[x² + 2(x)(1) + 1² - 1²] - 2

y = -[(x + 1)² - 1²] - 2

y = -[(x + 1)² - 1] - 2

y = -(x + 1)² + 1 - 2

y = -(x + 1)² - 1

Vertex :

Comparing y = a(x - h)² + k and y = -(x + 1)² - 1,

h = -1 and k = -1

So, the vertex is (h, k) = (-1, -1).

Example 2 :

Write the quadratic equation in vertex form and sketch the graph. 

y = -x² + 2x + 3

Solution :

Vertex form of the quadratic equation :

y = -x² + 2x + 3

y = -(x² - 2x) + 3

y = -[x² - 2(x)(1) + 1² - 1²] + 3

y = -[(x - 1)² - 1²] + 3

y = -[(x - 1)² - 1] + 3

y = -(x - 1)² + 1 + 3

y = -(x - 1)² + 4

Vertex :

Comparing y = a(x - h)² + k and y = -(x - 1)² + 4,

h = 1 and k = 4

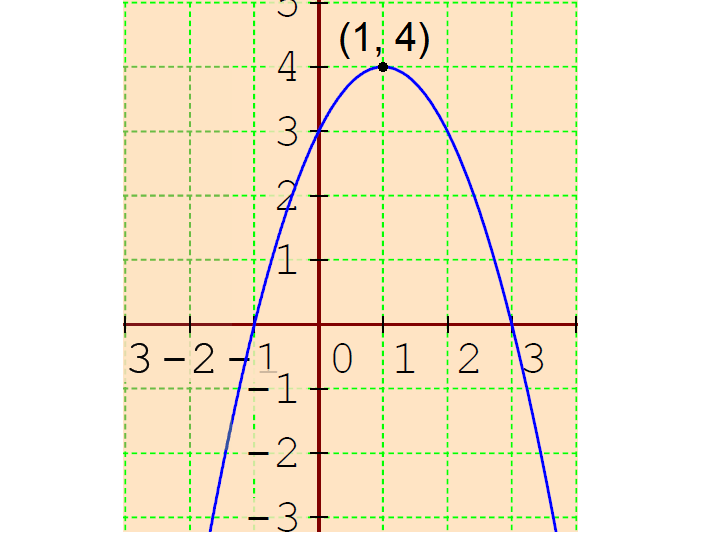
So, the vertex is (h, k) = (1, 4).

To graph the above quadratic equation, we need to find x-intercept and y-intercept, if any.

x-intercept :

To find the x-intercept, substitute y = 0. 

0 = -(x - 1)² + 4

(x - 1)² = 4

Take square root on both sides.

x - 1 = ±√4

x - 1 = ±2

x - 1 = -2  or  x - 1 = 2

x = -1  or  x = 3

So, the x-intercepts are (-1, 0) and (3, 0) .

y-intercept :

To find the y-intercept, substitute x = 0.

y = -(0 - 1)² + 4

y = -(-1)² + 4

y = -1 + 4

y = 3

So, the y-intercept is (0, 3).

Comparing y = a(x - h)² + k and y = -(x - 1)² + 4,

a = -1

So, the parabola opens down with vertex (1, 4), x-intercepts (-1, 0) and (3, 0) and y-intercept (0, 3).

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.