VERTICAL EXPANSIONS AND COMPRESSIONS

How to Do Vertical Expansion

Let f(x) be a function.

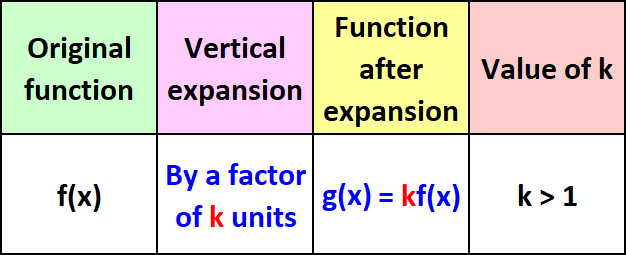
In the above function, if we want to do vertical expansion by a factor of k, at every where of the function, y co-ordinate has to be multiplied by k.

Then, we get the new function

g(x) = kf(x)

The graph of g(x) = kf(x) can be obtained by expanding the graph of f(x) vertically by the factor k.

It can be done by using the rule given below.

Note :

Point on the original curve : (x, y)

Point on the curve after it is vertically expanded (stretched) or compressed by the factor of k :

(x, ky)

How to Do Vertical Compression

Let f(x) be a function.

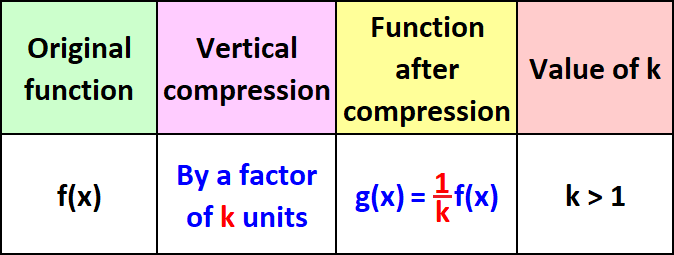
In the above function, if we want to do vertical compression by a factor of k, at every where of the function, y co-ordinate has to be multiplied by 1/k.

Then, we get the new function

g(x) = (1/k)f(x)

The graph of g(x) = kf(x) can be obtained by compressing the graph of f(x) vertically by the factor k.

It can be done by using the rule given below.

Note :

Point on the original curve : (x, y)

Point on the curve after it is vertically compressed by the factor of k :

(x, (1/k)ky)

Example 1 :

Perform a vertical expansion by a factor 2 to the function

f(x) = x²

And also write the formula that gives the requested transformation and draw the graph of both the given function and the transformed function

Answer :

Step 1 :

Let g(x) be a function which represents f(x) after the vertical expansion by a factor of 2.

Since we do vertical expansion by the factor 2, we have to replace x² by 2x² in f(x) to get g(x).

Step 2 :

So, the formula that gives the requested transformation is

g(x) = 2x²

Step 3 :

The graph g(x) = 2x² can be obtained by expanding the graph of the function f(x) = x² vertically by the factor 2.

(x, y) -----> (x, 2y)

Step 4 :

Table of values :

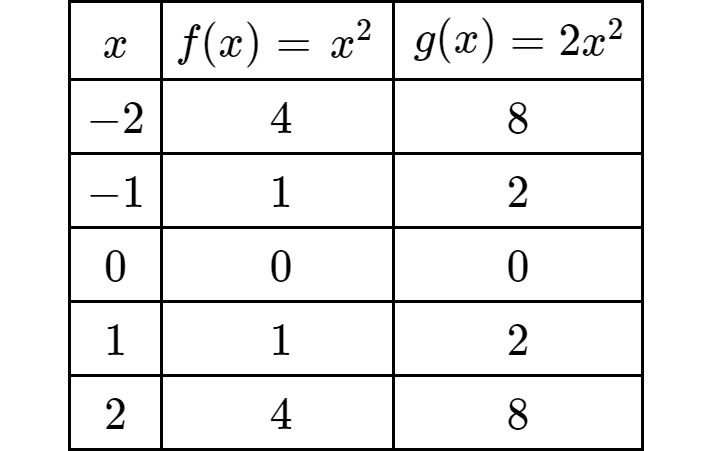
Step 5 :

Graphs of f(x) and g(x) :

Example 2 :

Perform a vertical compression by a factor 2 to the function

f(x) = x²

And also write the formula that gives the requested transformation and draw the graph of both the given function and the transformed function

Answer :

Step 1 :

Let g(x) be a function which represents f(x) after the vertical compression by a factor of 2.

Since we do vertical compression by the factor 2, we have to replace x² by (1/2)x² in f(x) to get g(x).

Step 2 :

So, the formula that gives the requested transformation is

g(x) = (1/2)x²

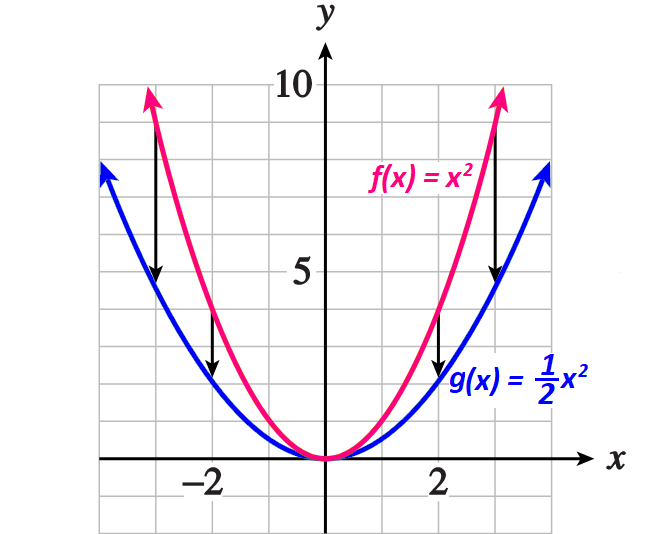
Step 3 :

The graph g(x) = (1/2)x² can be obtained by compressing the graph of the function f(x) = x² vertically by the factor 2.

(x, y) -----> (x, (1/2)y)

Step 4 :

Table of values :

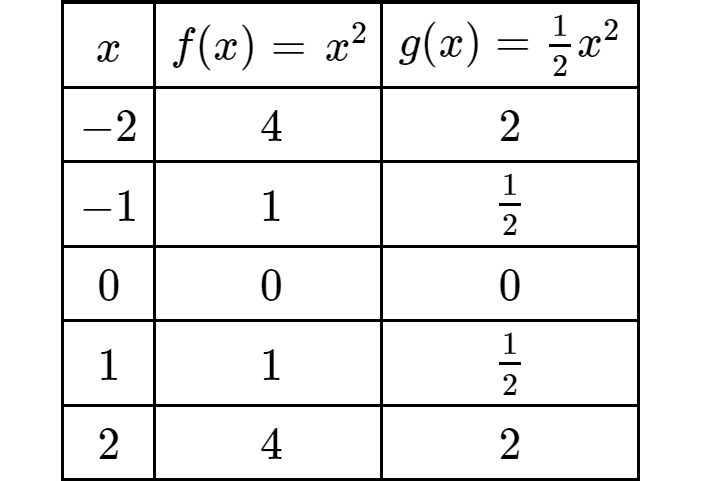
Step 5 :

Graphs of f(x) and g(x) :

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.