VOLUME OF CONE QUESTIONS AND ANSWERS

Question 1 :

Radius and slant height of a cone are 20 cm and 29 cm respectively. Find its volume.

Solution :

Radius of the cone (r)  =  20 cm

Slant height of the cone (l)  =  29 cm

l²  =  r²+h²

29²  =  20² + h²

841 = 400 + h²

h²  =  841-400

 h²  =  441

 h  =  √(21 21

 h  =  21 cm

Volume of the cone  =  (1/3) Π r² h

=   (1/3) ⋅ (22/7) ⋅ (20)² ⋅ 21

=  8800 cm³ 

Volume of the cone = 8800 cm³

Question 2 :

The circumference of the base of a 12 m high wooden solid cone is 44 m. Find the volume.

Solution :

Circumference of cone  =  44 m

Height of the cone (h)  =  12 m

2Πr  =  44

2 ⋅ (22/7) ⋅ r  =  44

r  =  44 ⋅ (1/2) ⋅ (7/22)

r  =  7 cm

Volume of the cone  =  (1/3) Π r² h

=  (1/3) ⋅ (22/7) ⋅ 7² ⋅ 12

=  (1/3) ⋅ (22/7) ⋅ 7 ⋅ 7 ⋅ 12

=  616 cm³ 

Volume of the cone  =  616 cm³ 

Question 3 :

A vessel is in the form of frustum of a cone. Its radius at one end and the height are 8 cm and 14 cm respectively. If its volume is 5676/3 cm³, then find the radius at the other end.

Solution :

Volume of the frustum cone  =  (5676/3) cm³

Let r be the required radius

Radius (R)  =  8 cm

height (h)  =  14 cm

(1/3) Π h (R²+r²+R r) = (5676/3)

(1/3) ⋅ (22/7) ⋅ (14) (8²+ r²+8r) = 5676/3

r²+8r+64  =  129

r²+ 8r+64-29  =  0

r²+8r-65  =  0

(r+13) (r-5)  =  0

r  =  -13, r  =  5 cm

So, the required radius = 5 cm

Question 4 :

The perimeter of the ends of a frustum of a cone are 44 cm and 8.4 Π cm. If the depth is 14 cm, then find its volume.

Solution :

Perimeter of upper end  =  44 cm

Perimeter of lower end  =  8.4 Π cm

Height of frustum cone  =  14 cm

Now we have to find the volume of frustum cone

Volume of the frustum cone  =  (1/3) Π h (R²+r²+R r)

2ΠR  =  44 

2 ⋅ (22/7) ⋅ R  =  44      

R  =  44 ⋅ (1/2) ⋅ (7/22)

R  =  2 ⋅ (1/2) ⋅ 7

R  =  7

2Πr  =  8.4 Π

r  =  8.4 Π ⋅ (1/2Π)

r  =  4.2 

Volume of the frustum cone

=  (1/3) ⋅ (22/7) ⋅ 14 (7²+4.2²+7(4.2))

=  (44/3) (49+29.4+17.64)

=  (44/3) (96.04)

=  (44) (32.013)

=  1408.57 cm³

Volume of the frustum cone =  1408.57 cm³

Question 5 :

You must answer a trivia question before the sand in the timer falls to the bottom. The sand falls at a rate of 50 cubic millimeters per second. How much time do you have to answer the question?

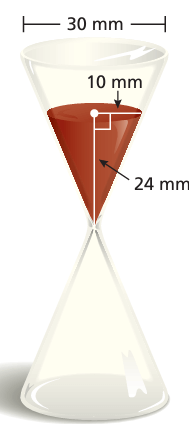
Solution :

Radius = 10 mm, height = 24 mm

Volume of sand in the conical timer = (1/3) Π r²h 

= (1/3) x 3.14 x 10² x 24

= 2512 mm³

The volume of the sand is about 2512 cubic millimeters. To fi nd the amount of time you have to answer the question, multiply the volume by the rate at which the sand falls.

2512 mm³ × (1 sec/50 mm³)

= 50.24 sec

You have about 50 seconds to answer the question.

Question 6 :

The volume of a cone is 20π cubic meters. What is the volume of a cylinder having the same base and same height?

Solution :

Volume of cone = 20π cubic meters

(1/3) Π r²h = 20π cubic meters

Multiplying by 3 on both sides, we get

Π r²h = 20π x 3

= 60π

Volume of cylinder = 60π cubic units.

Question 7 :

Water leaks from a crack in a vase at a rate of 0.5 cubic inch per minute. How long does it take for 20% of the water to leak from a full vase?

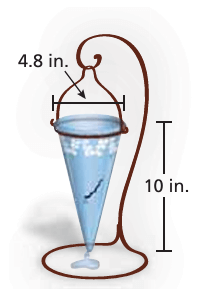
Solution :

Quantity of water in the vase = (1/3) Π r²h

diameter = 4.8 inches

radius = 2.4 inches and height = 10 inches

= (1/3) Π (2.4)²(10)

= (1/3) x 3.14 x (2.4)²(10)

= 60.28 cubic inches.

20% of quantity of water = 0.20(60.28)

= 12.056

Time taken = 12.056/0.5

= 24.11 minutes

So, the time taken is 24 minutes

Question 8 :

You have 10 gallons of lemonade to sell. (1 gal ≈ 3785 cm³)

a. Each customer uses one paper cup. How many paper cups will you need?

b. The cups are sold in packages of 50. How many packages should you buy?

c. How many cups will be left over if you sell 80% of the lemonade?

Solution :

a)  Radius = 4 cm and height = 11 cm

Volume of conical vessel = (1/3) Π r²h

= (1/3) Π (4)²(11)

= (1/3) x 3.14 x 16 x 11

Quantity of drink in 1 cup = 184.21 cm³

1 gal ≈ 3785 cm³

10 gallons = 37850 cm³

Number of cups = 37850/184.21

≈ 205 cups

Therefore, number of packs of cups required is 205.

b) Number of cups required = 205

Number of cups in each packs = 50

number of package = 205/50

= 4.1

So, 5 packages are needed.

c) 80% of lemonade = 0.80 (37850)

= 30280

Number of cups needed = 30280/184.21

= 164

Since we are purchasing 5 package (250 cups), the remaining number of cups = 250 - 164

= 86 cups will be remaining.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.