VOLUME OF CUBOID WORD PROBLEMS

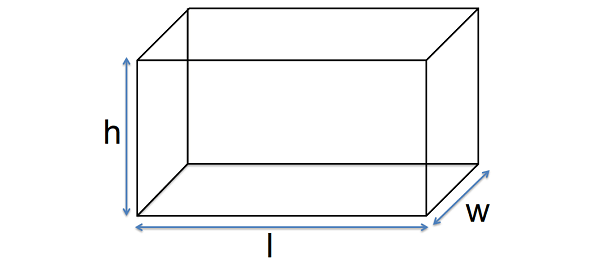
If the length, width and height of a cuboid are l, w and h respectively, then

volume  of the cuboid = l x w x h cubic units

Problem 1 :

The length, breadth and depth of a pond are 20.5 m, 16 m and 8 m respectively. Find the capacity of the pond in liters.

Solution :

l = 20.5 m, w = 16 m, h = 8 m

Capacity of pond :

= l x w x h

= 20.5 (16) (8)

= 2624 m³

1 m³ = 1000 liters

= 2624(1000) liters

= 2624000 liters

Problem 2 :

The dimensions of a brick are 24 cm x 12 cm x 8 cm. How many such bricks will be required to build a wall of 20 m length, 48 cm breadth and 6 m height?

Solution :

Volume of 1 brick :

= l x w x h

= 24(12)(8)

  = 2304 cm³

Dimensions of the wall :

l = 20 m = 2000 cm

w = 48 cm

h = 6 m = 6000 cm

Volume of wall :

= l x w x h

= 2000(48)(600)

= 57600000 cm³

Number of bricks required :

= 57600000/2304

= 25000

Problem 3 :

The volume of a container is 1440 m³. The length and width of the container are 15 m and 8 m respectively. Find its height

Solution :

Volume of container = 1440 m³

length x width x height = 1440

15 x 8 x h = 1440

height = 1440/120

height = 12 m

Problem 4 :

The side of a metallic cube is 12 in. It is melted and formed into a cuboid whose length and width are 18 in and 16 cm respectively. Find the height of the cuboid.

Solution :

volume of cuboid = volume of cube

l x w x h = a³

18 x 16 x h = 12³

288h = 1728

Divide both sides by 288.

h = 6 in

Problem 5 :

The length, width and height of a cuboid are in the ratio 7 : 5 : 2. Its volume is 35840 cm3. Find its dimensions.

Solution :

From the ratio 7 : 5 : 2, the dimensions of the cuboid are

length = 7x, width = 5x, height = 2x

Volume = 35840 cm³

(7x)(5x)(2x) = 35840

70x³ = 35840

Divide each side by 70.

x³ = 512

x³ = 8³

x = 8

Length = 7(8) = 56 cm

Width = 5(8) = 40 cm

Height = 2(8) = 16 cm

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.