WHY ROLLES THEOREM IS NOT APPLICABLE FOR THE GIVEN FUNCTION

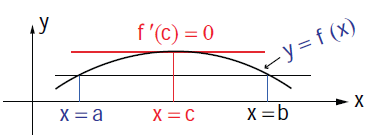
Let f (x) be continuous on a closed interval [a, b] and differentiable on the open interval (a, b)

If f(a)  =  f(b)

then there is at least one point c ∈ (a,b) where f '(c) = 0.

Geometrically this means that if the tangent is moving along the curve starting at x = a towards x = b then there exists a c ∈ (a, b) at which the tangent is parallel to the x -axis.

Explain why Rolle’s theorem is not applicable to the following functions in the respective intervals.

Problem 1 :

f(x)  =  |(1/x)|, x ∊ [-1, 1]

Solution :

The function is not defined at the interval [-1, 1], because when we apply x  =  0, we get

f(0)  =  |1/0|

f(0)  =  ∞

So, rolle's theorem is not applicable for the given function.

Problem 2 :

f (x) = tan x, x ∊ [0, π]

Solution :

The function is not defined at the interval [0, π], because when we apply x  =  π/2, we get

f(π/2)  =  tan (π/2)

f(π/2)  =  ∞

So, rolle's theorem is not applicable for the given function.

Problem 3 :

f(x)  =  x - 2 log x, x ∊ [2, 7]

Solution :

f(x)  =  x - 2 log x

f(x) is defined and continuous on the interval [2, 7] and differentiable on (2, 7).

f(x)  =  x - 2 log x

f(2)  ≠ f(7)

(1)  ≠ (2)

So, rolle's theorem is not applicable for the given function.

For each problem, determine if the Rolle's Theorem can be applied. If it can, find all values of c that satisfy the theorem. If it cannot, explain why not

Problem 4 :

y = (-x² + 1)/3x   [1, 6]

Solution :

i)  The given function y is defined and continuous on the closed interval [1, 6].

ii) Let f(x) = y = (-x² + 1)/3x

u = -x² + 1 and v = 3x

u' = -2x + 0 and v' = 3(1)

u' = -2x and v' = 3

d(u/v) = (vu' - uv')/v²

= [3x(-2x) - (-x² + 1)3] / (3x)²

= [-6x² + 3x² - 3] / 9x²

= (-3x² - 3)/ 9x²

= 3(-x² - 1) / 9x²

= (-x² + 1) / 3x²

It is differentiable in the open interval (1, 6)

iii) f(x) = (-x² + 1)/3x 

f(1) = (-x² + 1)/3x

 = (-1² + 1)/3(1) 

= 0 ----(1)

f(6) = (-6² + 1)/3(6)

 = (-36 + 1)/18

= -35/8 ----(2)

(1) and (2) are not equal.

Since the condition is not satisfied, rolle's theorem does not exists.

Problem 5 :

y = (x² - x - 12)/(x + 4)   [-3, 4]

Solution :

i)  The given function y is defined and continuous on the closed interval [-3, 4].

ii) Let f(x) = y = (x² - x - 12)/(x + 4)

u = x² - x - 12 and v = x + 4

u' = 2x - 1 - 0 and v' = 1 + 0

u' = 2x - 1 and v' = 1

d(u/v) = (vu' - uv')/v²

= [(x + 4)(2x - 1) - (x² - x - 12)1] / (x + 4)²

= [(2x² - x + 8x - 4) - (x² - x - 12)] / (x + 4)²

= [2x² + 7x - 4 - x² + x + 12] / (x + 4)²

= (x² + 8x + 8) / (x + 4)²

It is differentiable in the open interval (-3, 4)

iii) f(x) = (x² - x - 12)/(x + 4)

f(-3) = ((-3)² - (-3) - 12)/(-3 + 4)

= (9 + 3 - 12)/1

= 0/1

= 0 -----(1)

f(4) = (4² - 4 - 12)/(4 + 4)

= (16 - 4 - 12)/8

= (16 - 16)/8

= 0 -----(2)

Rolle's theorem exists.

To find the value of c, we do f'(c) = 0

f'(x) = (x² + 8x + 8) / (x + 4)²

f'(c) = (c² + 8c + 8) / (c + 4)²

 (c² + 8c + 8) / (c + 4)² = 0

c² + 8c + 8 = 0

Solving the above quadratic equation using the formula.

a = 1, b = 8 and c = 8

= (-b ± √b² - 4ac)/2a

= [8 ± √8² - 4(1)(8)]/2(1)

= [-8 ± √(64 - 32)]/2

= [-8 ± √32]/2

= [-8 ± 4√2]/2

= [-4 ± 2√2]

So, the values of c are -4 + 2√2 and -4 - 2√2.

Problem 6 :

y = (-x² - 2x + 8)/(-x + 3);   [-4, 2]

Solution :

i)  The given function y is defined and continuous on the closed interval [-4, 2].

ii) Let f(x) = y = (-x² - 2x + 8)/(-x + 3)

u = -x² - 2x + 8 and v = -x + 3

u' = -2x - 2(1) + 0 and v' = -1 + 0

u' = -2x - 2 and v' = -1

d(u/v) = (vu' - uv')/v²

= [(-x + 3)(-2x - 2) - (-x² - 2x + 8)(-1)] / (-x + 3)²

= [(2x² + 2x - 6x - 6) - (-x² - 2x + 8)(-1)] / (-x + 3)²

= [(2x² - 4x - 6) + (-x² - 2x + 8)] / (-x + 3)²

= [2x² - 4x - 6 - x² - 2x + 8] / (-x + 3)²

= (x² - 6x + 2) / (-x + 3)²

It is differentiable in the open interval (-4, 2)

iii) f(x) = (-x² - 2x + 8)/(-x + 3)

f(-4) = (-(-4)² - 2(-4) + 8)/(-(-4) + 3)

= (-16 + 8 + 8)/(4 + 3)

= 0/7

= 0 -----(1)

f(2) = (-2² - 2(2) + 8)/(-2 + 3)

= (-4 - 4 + 8)/1

= 0/1

= 0 -----(2)

Rolle's theorem exists.

To find the value of c, we do f'(c) = 0

f'(x) = (x² - 6x + 2) / (-x + 3)²

f'(c) = (c² - 6c + 2) / (-c + 3)²

(c² - 6c + 2) / (-c + 3)²= 0

c² - 6c + 2 = 0

Solving the above quadratic equation using the formula.

a = 1, b = -6 and c = 2

= (-b ± √b² - 4ac)/2a

= [6 ± √(-6)² - 4(1)(2)]/2(1)

= [6 ± √(36 - 8)]/2

= [6 ± √28]/2

= [6 ± 2√7]/2

= 3 ± √7

So, the values of c are 3 + √7 and 3 - √7.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.