WORKSHEET ON SIMILAR TRIANGLES

(1)  Check whether the which triangles are similar and find the value of x.

(i)  

Solution

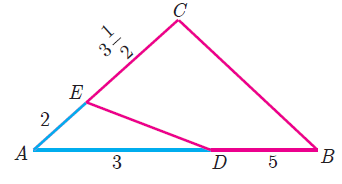
(ii)

Solution

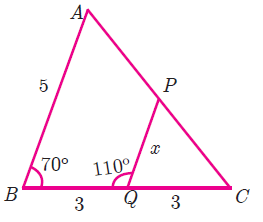
(2)  A girl looks the reflection of the top of the lamp post on the mirror which is 6.6 m away from the foot of the lamppost. The girl whose height is 1.25 m is standing 2.5 m away from the mirror. Assuming the mirror is placed on the ground facing the sky and the girl, mirror and the lamppost are in a same line, find the height of the lamp post.         Solution

(3)  A vertical stick of length 6 m casts a shadow 400 cm long on the ground and at the same time a tower casts a shadow 28 m long. Using similarity, find the height of the tower.         Solution

(4)  Two triangles QPR and QSR, right angled at P and S respectively are drawn on the same base QR and on the same side of QR. If PR and SQ intersect at T, prove that PT × TR = ST × TQ.         Solution 

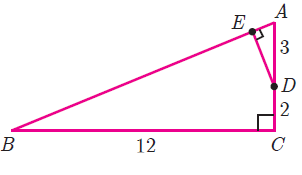
(5)  In the adjacent figure, triangle ABC is right angled at C and DE ⊥ AB . Prove that ΔABC ∼ ΔADE and hence find the lengths of AE and DE.

Solution

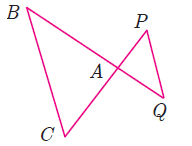
(6)  In the adjacent figure, ΔACB  ∼ ΔAPQ . If BC = 8 cm, PQ = 4 cm, BA = 6.5 cm and AP = 2.8 cm, find CA and AQ.

Solution

(7)  If figure OPRQ is a square and <MLN = 90° . Prove that (i) ΔLOP ∼ ΔQMO (ii) ΔLOP ∼ ΔRPN (iii) ΔQMO ∼ ΔRPN (iv) QR² = MQ × RN

Solution

(8)  If ΔABC  ∼ ΔDEF such that area of ΔABC is 9cm² and the area of ΔDEF is 16cm² and BC = 2.1 cm. Find the length of EF.          Solution

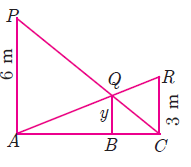
(9)  Two vertical poles of heights 6 m and 3 m are erected above a horizontal ground AC. Find the value of y.

Solution

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.