WORKSHEET ON VOLUME OF CONE
(1) Radius and slant height of a cone are 20 cm and 29 cm respectively. Find its volume.
(2) The circumference of the base of a 12 m high wooden solid cone is 44 m. Find the volume.
(3) A vessel is in the form of frustum of a cone. Its radius at one end and the height are 8 cm and 14 cm respectively. If its volume is 5676/3 cm3, then find the radius at the other end
(4) The perimeter of the ends of a frustum of a cone are 44 cm and 8.4 Π cm. If the depth is 14 cm, then find its volume.
(5) A right angled triangle ABC with sides 5 cm, 12 cm and 13 cm is revolved about the fixed side of 12 cm. Find the volume of the solid generated.
(6) The radius and height of a right circular cone are in the ratio 2:3. Find the slant height if its volume is 100.48 cu.cm (take Π = 3.14)
(7) The volume of a cone with circular base is 216 Π cu.cm. If the base radius is 9 cm, then find the height of the cone.
8) The volume of cone shown is 150 cm3
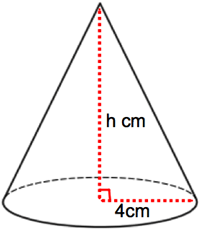
Calculate the height of the cone.
(9) A cone below has base radius 10cm and height h cm. A smaller cone radius 4cm and height 6cm is cut from the top. The frustum is shown below.
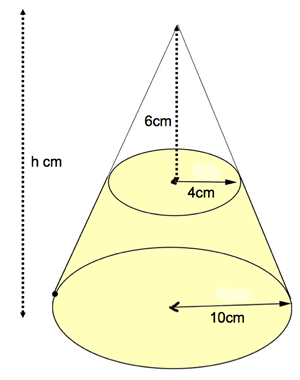
Calculate the volume of frustum cone.

Detailed Answer Key
(1) Solution :
Radius of the cone (r) = 20 cm
Slant height of the cone (l) = 29 cm
l2 = r2+h2
292 = 202 + h2
841 = 400 + h2
h2 = 841-400
h2 = 441
h = √(21 21
h = 21 cm
Volume of the cone = (1/3) Π r2 h
= (1/3) ⋅ (22/7) ⋅ (20)2 ⋅ 21
= 8800 cm3
Volume of the cone = 8800 cm3
(2) Solution :
Circumference of cone = 44 m
Height of the cone (h) = 12 m
2Πr = 44
2 ⋅ (22/7) ⋅ r = 44
r = 44 ⋅ (1/2) ⋅ (7/22)
r = 7 cm
Volume of the cone = (1/3) Π r² h
= (1/3) ⋅ (22/7) ⋅ 72 ⋅ 12
= (1/3) ⋅ (22/7) ⋅ 7 ⋅ 7 ⋅ 12
= 616 cm3
Volume of the cone = 616 cm3
(3) Solution :
Volume of the frustum cone = (5676/3) cm3
Let r be the required radius
Radius (R) = 8 cm
height (h) = 14 cm
(1/3) Π h (R2+r2+R r) = (5676/3)
(1/3) ⋅ (22/7) ⋅ (14) (82+ r2+8r) = 5676/3
r2+8r+64 = 129
r2+ 8r+64-29 = 0
r2+8r-65 = 0
(r+13) (r-5) = 0
r = -13, r = 5 cm
So, the required radius = 5 cm
(4) Solution :
Perimeter of upper end = 44 cm
Perimeter of lower end = 8.4 Π cm
Height of frustum cone = 14 cm
Now we have to find the volume of frustum cone
Volume of the frustum cone = (1/3) Π h (R2+r2+R r)
2ΠR = 44
2 ⋅ (22/7) ⋅ R = 44
R = 44 ⋅ (1/2) ⋅ (7/22)
R = 2 ⋅ (1/2) ⋅ 7
R = 7
2Πr = 8.4 Π
r = 8.4 Π ⋅ (1/2Π)
r = 4.2
Volume of the frustum cone
= (1/3) ⋅ (22/7) ⋅ 14 (72+4.22+7(4.2))
= (44/3) (49+29.4+17.64)
= (44/3) (96.04)
= (44) (32.013)
= 1408.57 cm3
Volume of the frustum cone = 1408.57 cm3
(5) Solution :
In any right triangle the longer side must be hypotenuse side. The longer side of the given sides is 13 cm.
So it must be hypotenuse side of the triangle.
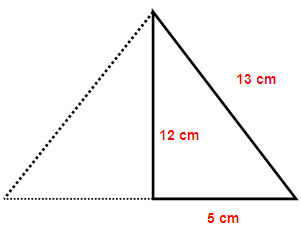
From the diagram we know that slant height is 13 cm, radius is 5 cm and height is 12 cm.
l = 13 cm, r = 5 cm and h = 12 cm
Volume of cone = (1/3)Πr2h
= (1/3) ⋅ (22/7) ⋅ 52⋅12
= (22/7) ⋅ 5 ⋅ 5 ⋅ 4
= 314.29 cm3
Volume of cone = 314.29 cm3
(6) Solution :
Radius and height of right circular cone are in the ratio
2 : 3
r : h = 2 : 3
r/h = 2/3
r = 2h/3
Volume of cone = 100.48 cu.cm
(1/3)Πr2 h = 100.48
(1/3) (3.14) (2h/3)2 h = 100.48
(1/3) (3.14) (4h2/9) h = 100.48
h3 = 100.48 ⋅ (3/1) ⋅ (1/3.14) ⋅ (9/4)
h3 = 8 ⋅ 27
h = 6 cm
r = 4 cm
l2 = r2+h2
l2 = 42+62
l = √52
l = 2√13 cm
So, slant height of the cone is 2√13 cm.
(7) Solution :
Volume of the cone = 216Π cu.cm
Radius of the cone = 9 cm
(1/3) Π r2 h = 216Π
92 h = 216 ⋅ 3
h = 216 ⋅ 3 ⋅ (1/9) (1/9)
h = 24/3
h = 8 cm
Required height of cone is 8 cm.
(8) Solution :
Volume of the cone = 150 cm3
(1/3) Π r2 h = 150
(1/3) x 3.14 x 42 h = 150
h = 150 x (3/1) x (1/3.14) x (1/16)
h = 8.95
Approximately is 9 cm
So, the height of the cone is 9 cm.
(9) Solution :

Volume of frustum cone = (1/3) Π h(R2 + r2 + Rr)
R = 10 cm, r = 4 cm
6/4 = h/10
h = 60/4
h = 15 cm
= (1/3) Π 15 (102 + 42 + 10(4))
= 3.14 (5) (100 + 16 + 40)
= 3.14 x 5 x 156
= 2449.2 cm3
So, volume of the frustum cone is 2449.2 cm3.
Kindly mail your feedback to v4formath@gmail.com
We always appreciate your feedback.
©All rights reserved. onlinemath4all.com
Recent Articles
-
Time and Work Problems
Oct 20, 25 07:13 AM
Time and Work Problems - Concept - Solved Problems -
10 Hard SAT Math Questions (Part - 30)
Oct 17, 25 07:27 PM
10 Hard SAT Math Questions (Part - 30) -
10 Hard SAT Math Questions (Part - 29)
Oct 16, 25 06:04 AM
10 Hard SAT Math Questions (Part - 29)
