WRITING ABSOLUTE VALUE EQUATIONS FROM GRAPHS

Write the absolute value equation of the following graph.

Example 1 :

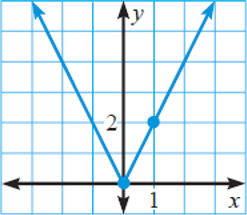
Solution :

Vertex of the absolute value function :

(h, k)  ==>  (0, 0)

From (0, 0) to (1, 2)

Rise is 2 units and run is 1 unit and it is open upward.

Slope (a)  =  Rise/Run

Slope (a)  =  2/1  ==>  2

Applying the the values discussed above, we get

y = a|x - h| + k

y = 2|x - 0| + 0

y = 2|x|

Example 2 :

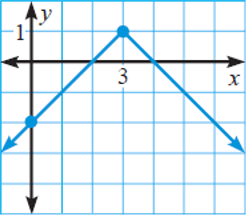
Solution :

Vertex of the absolute value function :

(h, k)  ==>  (3, 1)

From (3, 1) to (4, 0)

Rise is 1 unit and run is 1 unit and it is open downward.

Slope (a)  =  Rise/Run

Slope (a)  =  1/1  ==>  -1

Applying the the values discussed above, we get

y  =  a |x-h| + k

y = -1|x-3|+1

So, the required absolute equation for the given graph is

y = -1|x-3|+1

Example 3 :

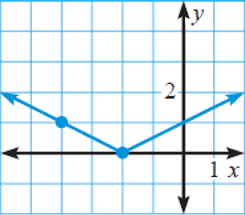
Solution :

Vertex of the absolute value function :

(h, k)  ==>  (-2, 0)

From (-2, 0) to (-5, 1)

Rise is 1 unit and run is 2 units and it is open upward.

Slope (a)  =  Rise/Run

Slope (a)  =  1/2  ==>  1/2

Applying the the values discussed above, we get

y  =  a |x-h| + k

y  =  (1/2)|x+2|+0

y  =  (1/2)|x+2|

So, the required absolute equation for the given graph is

y  =  (1/2)|x+2|

Example 4 :

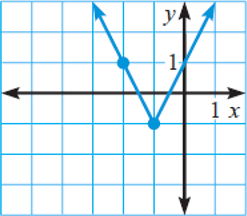
Solution :

Vertex of the absolute value function :

(h, k)  ==>  (-1, -1)

From (-1, -1) to (-2, 1)

Rise is 2 units and run is 1 unit and it is open upward.

Slope (a)  =  Rise/Run

Slope (a)  =  2/1  ==>  2

Applying the the values discussed above, we get

y  =  a |x-h| + k

y  =  2|x+1|+(-1)

y  =  2|x+1|-1

So, the required absolute equation for the given graph is

y  =  2|x+1|-1

Example 5 :

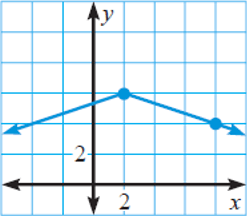
Solution :

Vertex of the absolute value function :

(h, k)  ==>  (2, 6)

From (2, 6) to (8, 4)

Rise is 1 unit and run is 3 units and it is open down

Slope (a)  =  Rise/Run

Slope (a)  =  -1/3

Applying the the values discussed above, we get

y  =  a |x-h| + k

y  =  (-1/3)|x-2|+6

y  =  (1/3)|x-2|+6

So, the required absolute equation for the given graph is

y  =  (1/3)|x-2|+6

Example 6 :

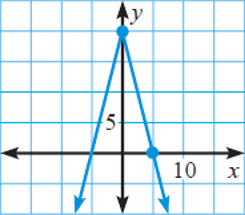
Solution :

Vertex of the absolute value function :

(h, k)  ==>  (0, 20)

From (0, 20) to (5, 0)

Rise is 4 units and run is 1 unit and it is opens down.

Slope (a)  =  Rise/Run

Slope (a)  =  -4/1  ==>  -4

Applying the the values discussed above, we get

y  =  a|x - h| + k

y  =  -4|x -0| + 20

y  =  -4|x| + 20

So, the required absolute equation for the given graph is

y  =  -4|x| + 20

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.