X AND Y INTERCEPTS

The x-intercept is the x-coordinate of the point where the graph intersects the x-axis. The y-coordinate of this point is always 0.

The y-intercept is the y-coordinate of the point where the graph intersects the y-axis. The x-coordinate of this point is always 0.

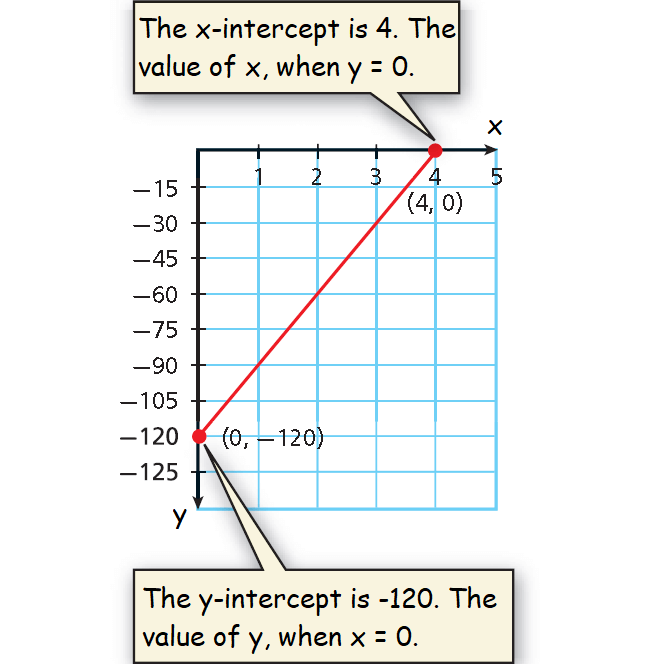
Finding Intercepts

Find the x- and y-intercepts. 

Example 1 :

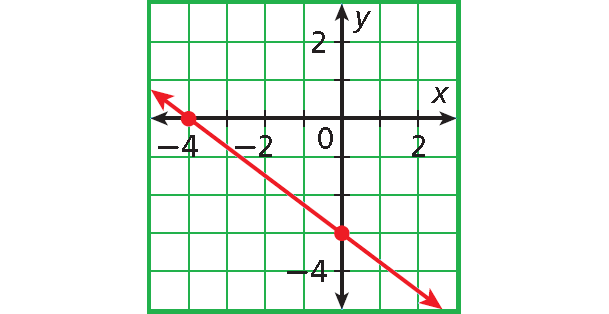
Solution :

The graph intersects the x-axis at (-4, 0). 

The x-intercept is -4.

The graph intersects the y-axis at (0, -3).

The y-intercept is -3.

Example 2 :

3x - 2y  =  12

Solution :

Travel Application

Example 3 :

A tramway travels a distance of about 4500 meters to the top of the peak. Its rate is 300 meters per minute. The function f(x) = 4500 - 350x gives the tram’s distance in meters from the top of the peak after x minutes. Graph this function and find the intercepts. What does each intercept represent?

Solution :

Neither time nor distance can be negative, so choose several nonnegative values for x. Use the function to generate ordered pairs.

Graph the ordered pairs. Connect the points with a line.

• y-intercept : 4500. This is the starting distance from the top (time = 0).

• x-intercept : 15. This the time when the tram reaches the peak (distance = 0).

Graphing Linear Equations by Using Intercepts

Use intercepts to graph the line described by each  equation.

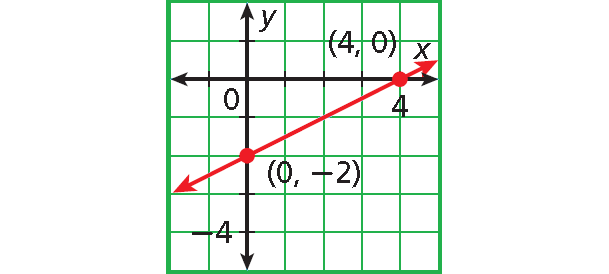
Example 4 :

2x - 4y  =  8

Solution :

Step 1 :

Find the intercepts.

Step 2 :

Plot (4, 0) and (0, -2).

Connect with a straight line.

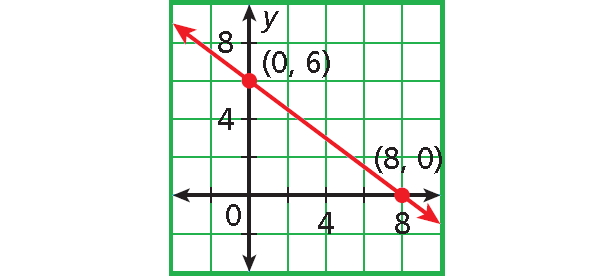
Example 5 :

2y  =  12 - 3x/2

Solution :

Step 1 :

Write the equation in standard form.

2y  =  12 - 3x/2

Multiply each side by 2. 

4y  =  24 - 3x

Add 3x to each side. 

3x + 4y  =  24

Step 2 :

Find the intercepts.

Step 3 :

Plot (8, 0) and (0, 6).

Connect with a straight line.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.