ADDING AND SUBTRACTING WITH SCIENTIFIC NOTATION WORKSHEET

Problem 1 :

Evaluate :

(1.328 x 10⁷) + (2.034 x 10⁵)

Problem 2 :

Evaluate :

(3.2 x 10^-3) - (8.02 x 10^-5)

Problem 3 : 

Simplify the expression given below. 

(0.723 x 10⁸) + (338.2 x 10⁵) - (6.1 x 10⁷)

Problem 4 : 

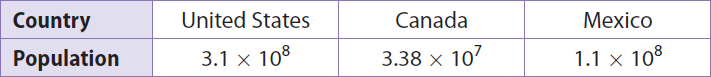
The table below shows the population of the three largest countries in North America in 2011. Find the total population of these countries.

Detailed Answer Key

Problem 1 :

Evaluate :

(1.328 x 10⁷) + (2.034 x 10⁵)

Give your answer in scientific notation. 

Solution :

(1.328 x 10⁷) + (2.034 x 10⁵)

In the given numbers, we don't have the same exponent for 10.

Adjust the exponents of 10 in the given numbers such that they have the same exponent.

It is easier to adjust the smaller exponent to equal the larger exponent. 

Then, 

=  (1.328 x 10⁷) + (0.02034 x 10⁷)

In the above numbers, we have the same exponent for 10.

So, factor 10⁷ out from the given numbers. 

=  (1.328 + 0.02034) x 10⁷

  =  1.34834 x 10⁷

The above number is in scientific notation.

Therefore, 

(1.328 x 10⁷) + (2.034 x 10⁵)  =  1.34834 x 10⁷

Problem 2 :

Evaluate :

(3.2 x 10^-3) - (8.02 x 10^-5)

Give your answer in scientific notation. 

Solution :

(3.2 x 10^-3) - (8.02 x 10^-5)

In the given numbers, we don't have the same exponent for 10.

Adjust the exponents of 10 in the given numbers such that they have the same exponent.

Then, 

=  (3.2 x 10^-3) - (0.0802 x 10² x 10^-5)

=  (3.2 x 10^-3) - (0.0802 x 10^2-5)

=  (3.2 x 10^-3) - (0.0802 x 10^-3)

In the above numbers, we have the same exponent for 10.

So, factor 10^-3 out from the given numbers. 

=  (3.2 - 0.0802) x 10^-3

=  3.1198 x 10^-3

Write the above number in scientific notation. 

=  3.1198 x 10^-3

Therefore, 

(3.2 x 10^-3) - (8.02 x 10^-5)  =  3.1198 x 10^-3

Problem 3 : 

Simplify the expression given below. 

(0.723 x 10⁸) + (338.2 x 10⁵) - (6.1 x 10⁷)

Solution :

Method 1 :

Step 1 : 

In the given numbers, the highest power of 10 is 8. 

So, write each number with 10 power 8.

0.723 x 10⁸  =  0.723 x 10⁸

338.2 x 10⁵  =  0.3382 x 10⁸

6.1 x 10⁷  =  0.61 x 10⁸

Step 2 : 

Simplify the multipliers.

0.723 + 0.3382 - 0.61  =  0.4512

Step 3 : 

Write the final answer in scientific notation :

0.4512 x 10⁸  =  4.512 x 10⁷

Method 2 :

Step 1 : 

First, write each number in standard notation.

0.723 x 10⁸  =  72,300,000

338.2 x 10⁵  =  33,820,000

6.1 x 10⁷  =  61,000,000

Step 2 : 

Simplify the numbers in standard notation.

72,300,000 + 33,820,000 - 61,000,000  =  45,120,000

Step 3 : 

Write the final answer in scientific notation :

45,120,000  =  4.512 x 10⁷

Problem 4 : 

The table below shows the population of the three largest countries in North America in 2011. Find the total population of these countries.

Solution :

Method 1 :

Step 1 : 

First, write each population with the same power of 10.

United States : 3.1 x 10⁸

Canada : 0.338 x 10⁸

Mexico : 1.1 x 10⁸

Step 2 : 

Add the multipliers for each population.

3.1 + 0.338 + 1.1  =  4.538

Step 3 : 

Write the final answer in scientific notation :

4.538 x 10⁸

Method 2 :

Step 1 : 

First, write each number in standard notation.

United States : 310,000,000

Canada : 33,800,000

Mexico : 110,000,000

Step 2 : 

Find the sum of the numbers in standard notation.

310,000,000 + 33,800,000 + 110,000,000  =  453,800,000

Step 3 : 

Write the final answer in scientific notation :

453,800,000  =  4.538 x 10⁸

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.