ALGEBRA PRACTICE QUESTIONS FOR GRADE 10

Question 1 :

Find the square root of 289x⁴ −612x³ + 970x² −684x + 361.

Solution :

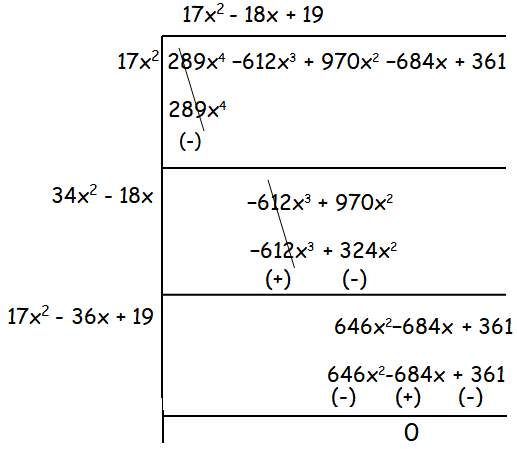
Question 2 :

Solve √(y + 1) + √(2y −5) = 3

Solution :

√(y + 1) + √(2y −5) = 3

√(y + 1)  = 3 - √(2y −5)

Taking squares on both sides

[√(y + 1)]²  =  [3 - √(2y −5)]²

(y + 1)  =  9 - 6√(2y −5) + (2y - 5)

y  + 1 - 2y + 5 - 9  =  - 6√(2y −5)

- y - 3  =  - 6√(2y −5)

[- (y + 3)]²  =  [- 6√(2y −5)]²

y² + 6y + 9  =  36(2y - 5)  

y² + 6y - 72y + 9 + 180  =  0

y² - 66y + 189  =  0

(y - 63)(y - 3)  =  0

y = 63 and y = 3

Question 3 :

A boat takes 1.6 hours longer to go 36 kms up a river than down the river. If the  speed of the water current is 4 km per hr, what is the speed of the boat in still water?

Solution :

Distance covered  =  36 kms

Let "x" be the speed of boat 

Let "y" be the speed of stream  =  4 km

Speed of down stream  =  x + y  =  x + 4

Speed of up stream  =  x - y  =  x - 4

Time taken  =  1.6 hours

Time = Distance / Speed

Time taken for upstream  =  36/(x - 4)  ---(1)

Time taken for downstream  =  36/(x + 4) ---(2)

(1) + (2)

36[1/(x - 4) - 1/(x + 4)]  =  1.6

x + 4 - x + 4/(x + 4)(x - 4)  =  1.6/36

8/(x² - 16)  =  16/360 

2880  =  16(x² - 16)

2880  =  16x²  -  256

2880 + 256  =  16x²

16x²  =  3136

x²  =  196

x  =  14

Hence the speed of the boat is 14 km/hr.

Question 4 :

Is it possible to design a rectangular park of perimeter 320 m and area 4800 m² ? If so find its length and breadth.

Solution :

Perimeter of the rectangular park  =  320 m

Area of park  =  4800

2(l + b)  =  320

l + b  =  160---(1)

l x b  =  4800

l = 4800/b

By applying the value of l in (1), we get

(4800/b) + b  =  160

(4800 + b²)/b  =  160

4800 + b²  =  160b

b² - 160b + 4800  =  0

(b - 120)(b - 40)  =  0

b  =  120 and b =  40

If b = 120, then l = 4800/120  =  40

If b = 40, then l = 4800/40  =  120

Hence the required measurements are 120 m and 40 m.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.