ALTERNATE EXTERIOR ANGLES WORKSHEET
Problem 1 :
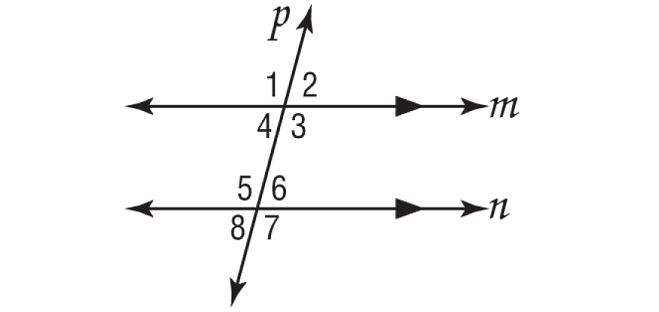
In the figure shown below, m∠8 = 75°. Find m∠1.
Problem 2 :
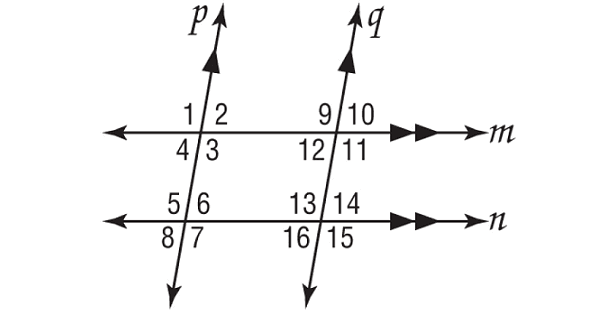
In the figure shown below, m∠2 = 78°. Find the measures of ∠8, ∠10 and ∠16.
Problem 3 :
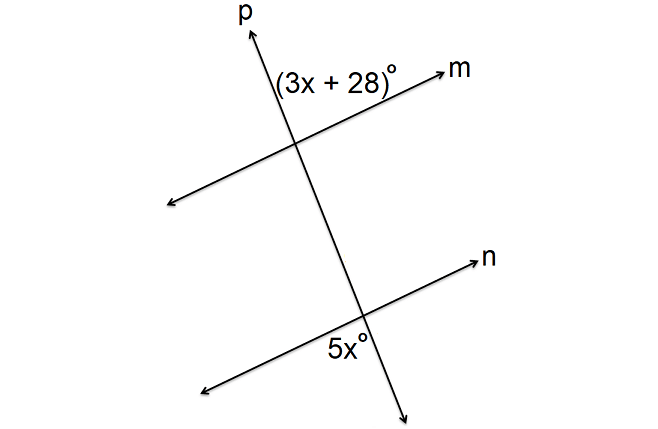
In the figure shown below, lines m and n are parallel and p is transversal. Find the value of x.
Problem 4 :
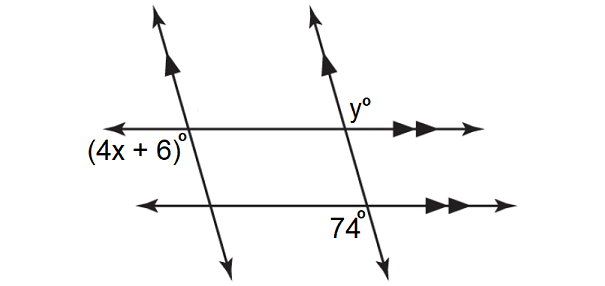
In the figure shown below, solve for x.

1. Answer :

In the figure above, lines m and n are parallel, ∠7 and ∠8 form a linear pair.
m∠7 + m∠8 = 180°
Substitute m∠8 = 75°.
m∠7 + 75° = 180°
Subtract 75° from each side.
m∠7 = 105°
∠1 and ∠7 are alternate exterior angles.
∠1 ≅ ∠7
m∠1 = m∠7
Substitute m∠7 = 105°.
m∠1 = 105°
2. Answer :

In the figure above, lines m and n are parallel, p and q are parallel.
∠2 and ∠8 are alternate exterior angles.
∠2 ≅ ∠8
m∠2 = m∠8
Substitute m∠2 = 78°.
78° = m∠8
∠8 and ∠16 are corresponding angles.
∠8 ≅ ∠16
m∠8 = m∠16
Substitute m∠8 = 78°.
78° = m∠16
∠10 and ∠16 are alternate interior angles.
∠10 ≅ ∠16
m∠10 = m∠16
Substitute m∠16 = 78°.
m∠10 = 78°
Therefore,
m∠8 = 78°
m∠10 = 78°
m∠16 = 78°
3. Answer :

In the figure above m and n are parallel and p is transversal. Angles 5x° and (3x + 28)° are alternate exterior angles and they are congruent.
By the definition of congruent angles,
5x° = (3x + 28)°
5x = 3x + 28
Subtract 3x from each side.
2x = 28
Divide each side by 2.
x = 14
4. Answer :

In the figure above, y° and 74° are alternate exterior angles and they are equal.
y° = 74°
(4x + 6)° and y° are alternate exterior angles and they are equal.
(4x + 6)° = y°
Substitute y° = 74°.
(4x + 6)° = 74°
4x + 6 = 74
Subtract 6 from each side.
4x = 68
Divide each side by 4.
x = 17
Kindly mail your feedback to v4formath@gmail.com
We always appreciate your feedback.
©All rights reserved. onlinemath4all.com
Recent Articles
-
Rational Root Theorem
Feb 06, 25 07:42 PM
Rational Root Theorem or Rational Zero Theorem - Concept - Examples -
How to Find Pythagorean Triplet from One Number
Feb 06, 25 06:10 PM
How to Find Pythagorean Triplet from One Number - Concept - Examples with step by step explanation -
AP Calculus AB Problems with Solutions (Part - 10)
Feb 06, 25 09:46 AM
AP Calculus AB Problems with Solutions (Part - 10)

