AREA AND PERIMETER

Square

A square is a four-sided polygon where the lengths of all the four sides will be equal and each vertex angle will be right angle or 90°  as shown below. 

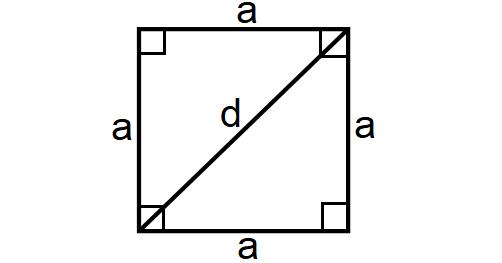
When the side length is given :

Area of a Square = a²

When the length of the diagonal is given :

Area of a Square = 1/2 ⋅ d²

Perimeter of a Square = 4a

Rectangle

A rectangle is a four-sided polygon where the lengths of opposite sides will be equal and each vertex angle will be 90° or right angle as shown below.

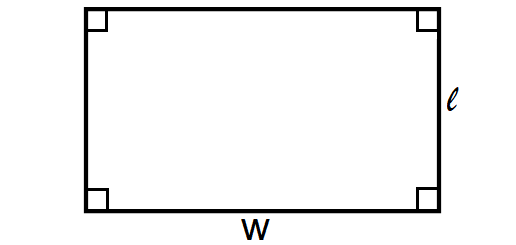
Area of a Rectangle = l ⋅ w

Perimeter of a Rectangle = 2(l + w)

Equilateral Triangle

An equilateral triangle is a three-sided polygon where the lengths of all the three sides will be equal and each vertex angle will be 60° as shown below.

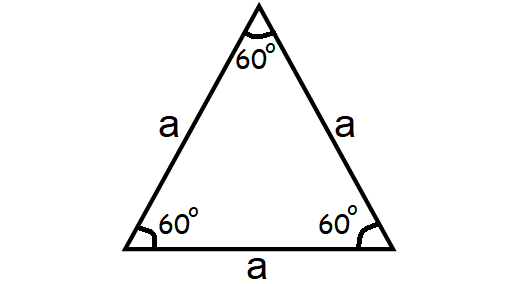
Area of an Equilateral Triangle = √3/4 ⋅ a²

Perimeter of an Equilateral Triangle = 3a

Scalene Triangle

An scalene triangle is a three-sided polygon where the all the three sides will have unequal lengths and the measures of all the three  angles will be different as shown below.

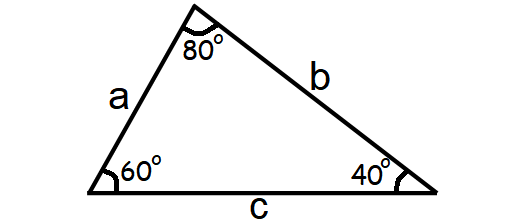
Area of a Scalene Triangle = √[s(s - a)(s - b)(s - c)]

where s = (a + b + c)/2

Perimeter of a Scalene Triangle = a + b + c

Quadrilateral

A quadrilateral is a four-sided polygon as shown below.

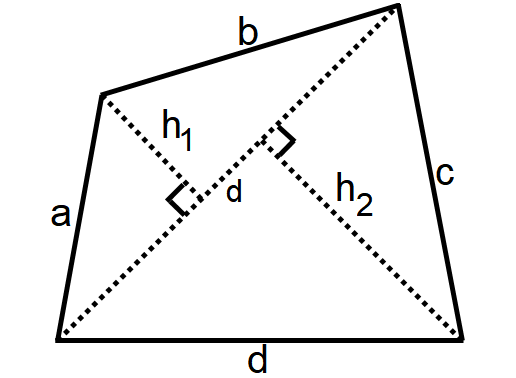
Area of a Quadrilateral = 1/2 ⋅ d ⋅ (h₁ + h₂)

Perimeter of a Quadrilateral = a + b + c + d

Parallelogram

A parallelogram is a four-sided polygon where the opposite sides will be equal and parallel as shown below.

Area of a Parallelogram = b ⋅ h

Perimeter of a Parallelogram = 2(a + b)

Rhombus

A rhombus is a four-sided polygon where the lengths of all the four sides will be equal and opposite sides will be parallel as shown below.

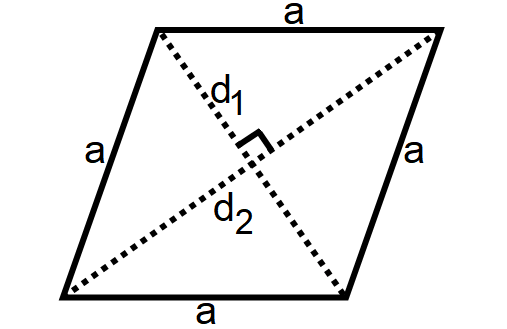
Area of a Rhombus = 1/2 ⋅ d₁ ⋅ d₂

Perimeter of a Rhombus = 4a

Trapezoid

A trapezoid is a four-sided polygon which has a pair of opposite sides parallel as shown below. 

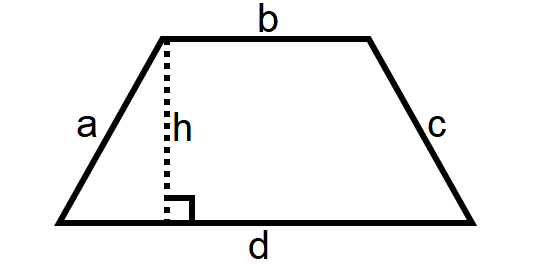
Area of a Trapezoid = 1/2 ⋅ h ⋅ (a + b)

Perimeter of a Trapezoid = a + b + c + d

Circle

A circle is a round plane figure whose boundary (the circumference) consists of points equidistant from a fixed point (the center). The distance between the center and any point on the circumference is called radius (r). 

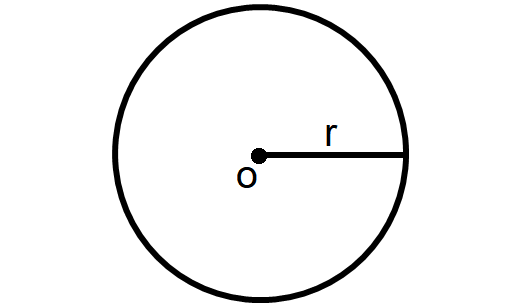
Area of a Circle = πr²

Perimeter of a Circle = 2πr

Semicircle

A semicircle is a half of a circle or of its circumference as shown below.

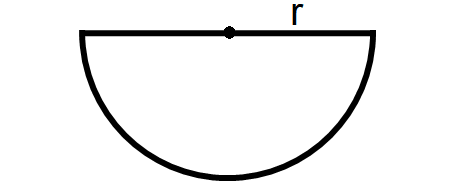
Area of a Semicircle = πr²/2

Perimeter of a Semicircle = πr + 2r

Quadrant

A quadrant is a one-fourth of a circle. 

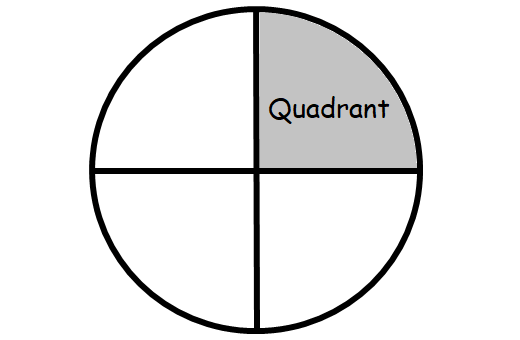
Area of a Quadrant = πr²/4

Perimeter of a Quadrant = πr/2 + 2r

Sector of a Circle

A sector of a circle is a part of the interior of the circle enclosed by an arc and two radii. 

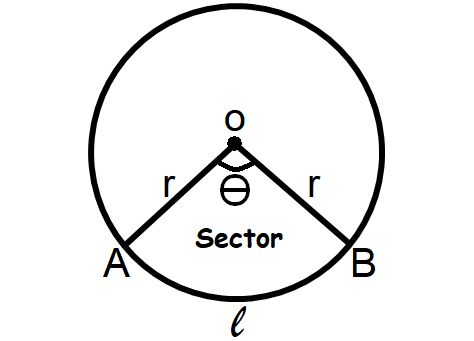
Area of a Sector = θ/360° ⋅ πr²

Length of the Arc AB = θ/360° ⋅ 2πr

Perimeter a Sector = (θ/360° ⋅ 2πr) + 2r

Regular Polygon

A regular polygon is a  polygon that is equiangular (all interior angles are equal in measure) and equilateral (all sides have the same length).

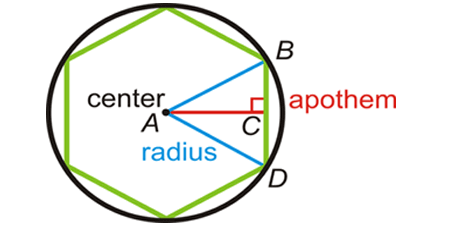
Area = 1/2 ⋅ (Apothem x Perimeter) 

Perimeter = No. of sides ⋅ Length of each side

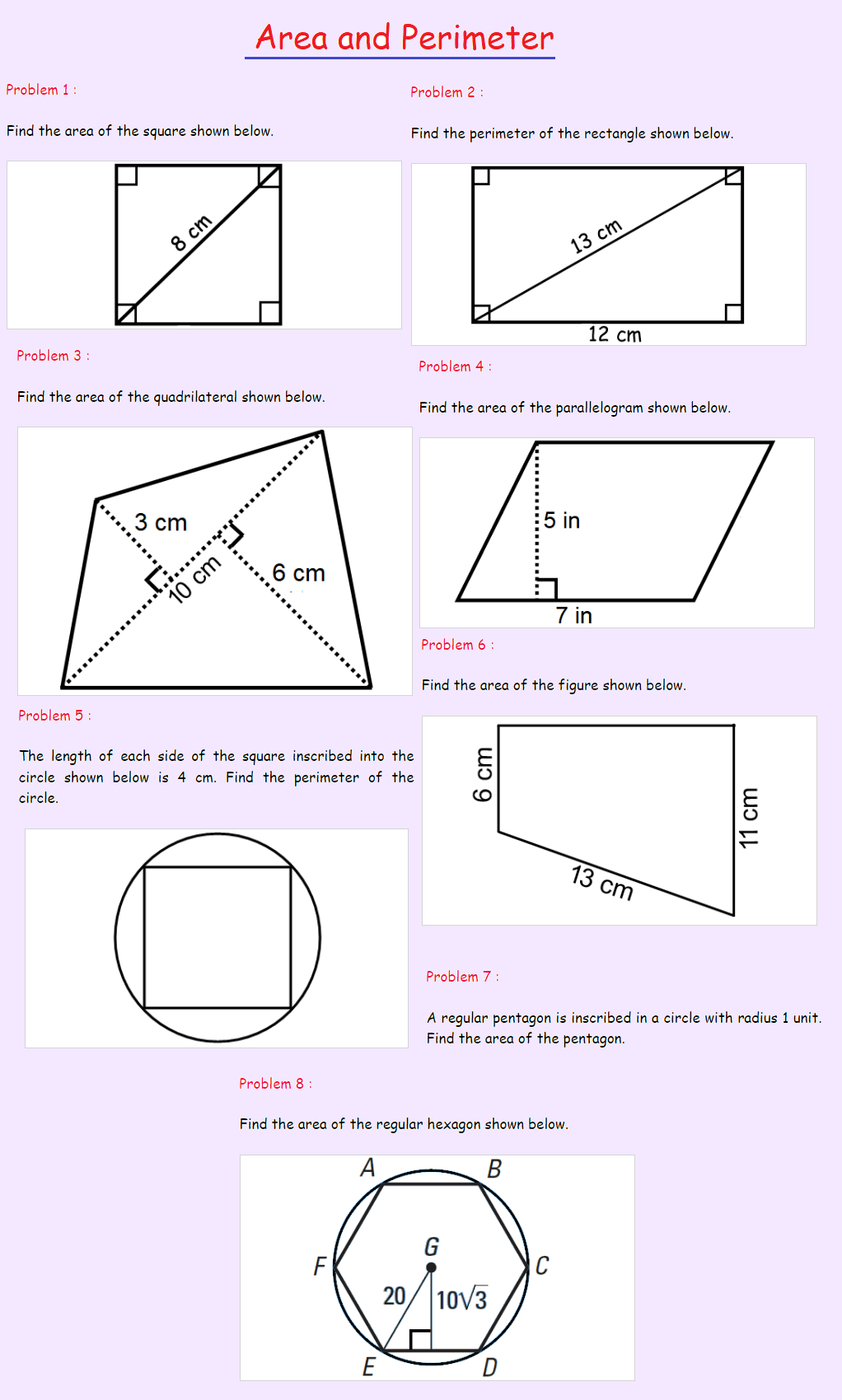
Solved Problems

Problem 1 :

If the length of each side of a square is 5 cm, find its perimeter.

Solution :

Perimeter of the square = 4 x length of each side

= 4 x 5

= 20 cm

Problem 2 :

If the length and width of a rectangle are 4.2 in. and 7 in. respectively. Find its area.

Solution :

Area of the rectangle = length x width

= 4.2 x 7

= 29.4 in.²

Problem 3 :

The length of a rectangle is 3 more than twice the width. If the perimeter of the rectangle is 36 cm, find the length and width of the rectangle.

Solution :

Let x be the width of the rectangle.

Then, length of the rectangle :

= 2x + 3

Perimeter of the rectangle = 36 cm

2(length + width) = 36

2(2x + 3 + x) = 36

2(3x + 3) = 36

Divide both sides by 2.

3x + 3 = 18

Subtract 3 from both sides.

3x = 15

Divide both sides by 3.

x = 5

2x + 3 = 2(5) + 3

2x + 3 = 10 + 3

2x + 3 = 13

Length and width of the rectangle are 13 cm and 5 cm respectively.

Problem 4 :

Find the area of a circle whose radius is 7 cm. Use π = 3.14 and round your answer to the nearest tenth.

Solution :

Area of the circle = πr²

= 3.14 x 7²

= 3.14 x 49

= 153.9 cm²

Problem 5 :

If the radius of a circle is 6.5 in., find its circumference. Use π = 3.14 and round your answer to the nearest tenth.

Solution :

Circumference of the circle = 2πr

= 2 x 3.14 x 6.5

= 40.82 in.

Click here to get detailed answers for the above questions.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.