AREA OF SHADED REGION WORD PROBLEMS WORKSHEET

Problem 1 :

A cylindrical tank has radius 1.5 m. What is the circumference of its base ?

Solution

Problem 2 :

A circular pond has diameter 8 m and needs to be fenced for the protection of children.

a) What length of fencing is required?

b) Fencing comes in 1 m lengths. How many lengths are needed?

c) What is the total cost of the fencing if each length costs $25.00?

Solution

Problem 3 :

A car wheel has a radius of 35 cm.

a)  What is the circumference of the wheel?

b)  If the wheel rotates 100 000 times, how far does the car travel?

Solution

Problem 4 :

A trundle wheel is used for measuring distances. The circumference of the wheel is exactly 1 m. Each time the wheel rotates through one complete turn a click sound is heard and a counter adds a meter to the total. What is the radius of this wheel?

Solution

Problem 5 :

A circular garden plot has circumference 12.65 m. Find its radius in meters, correct to the nearest centimetre.

Solution

Problem 6 :

An irrigation sprinkler sprays water over a field. The radius of the spray is 12.6 m. What area of the field is being watered?

Solution

Problem 7 :

The rope connecting a goat to a pole is 8 m long. What area of grass can the goat eat?

Solution

Problem 8 :

A circular hoop has a radius of 40 cm. Find the length of tubing needed to make the hoop.

Solution

Problem 9 :

6 identical metal discs are stamped out of an 18 cm by 12 cm sheet of copper as illustrated. What percentage of the copper is wasted

Solution

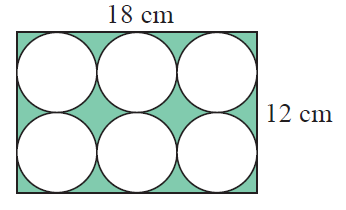
Problem 10 :

A table-top is shaped as illustrated. A cloth to protect the table-top from stains and heat is cut exactly the same size as the table-top. It is made from fabric 1.6 m wide and costs $18.40 per metre of length.

a) What length of fabric must be purchased?

b) Calculate the cost of the fabric.

c) Find the area of the cloth.

d) Calculate the amount of fabric that is wasted.

Solution

Answers :

(1)  9.42 m²

(2)  (a)  25.12  (b)  26  (c)  $650

(3)  (a)  220 cm  (b)   220 km

(4)  159 mm.

(5)  r  =  2.01 m

(6)  498.50 m²

(7)  201 m²

(8)  251.2 cm.

(9)  21.5%

(10)   (a)  4 m  (b)  $73.60  (c)  5.85 m²   (d)  0.55 m²

Problem 1 :

The diagram below shows the rectangular pen in which Gomer confines his wolverines and badgers. As a special treat, Gomer is going to cover the badgers' area of the pen with Astroturf. Assuming that Astroturf costs $1.25 per square foot, how much with this project cost?

A. $1500    B. $9000    C. $4500    D. $3000

Solution

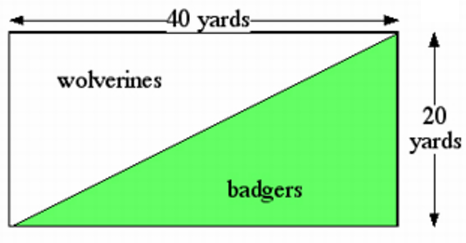
Problem 2 :

The diagram below shows the plan for a new parking lot at the South westdale Mall. It is estimated that such construction costs $12 per square yard. What will be the total cost for the parking lot?

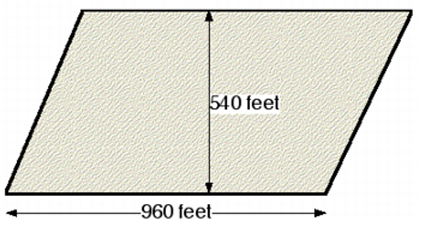
A. $2,073,600         B. $691,200

C. $6,220,800          D. $518,400

Solution

Problem 3 :

The diagram below shows a circular pizza whose diameter is 8 inches, situated in a square box whose side length is 10 inches. How much of the box is "empty?"

Solution

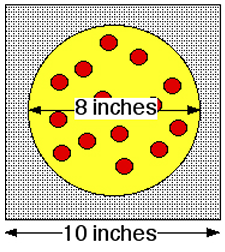
Problem 4 :

The diagram below shows one exterior wall of a house. The wall has a door opening that measures 3 feet by 7 feet, and two window openings that each measure 6 feet by 4 feet. The wall, but not the openings, will be covered with siding material that costs $1.50 per square foot. Find the total cost of the siding material.

Solution

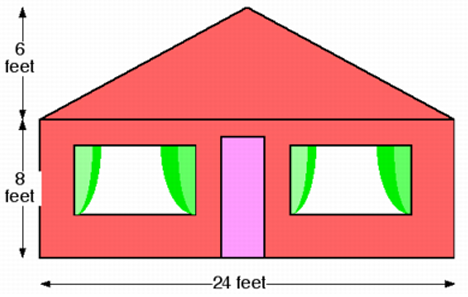
Problem 5 :

Which is larger: a square cake pan that measures 8 inches on each side, or a circular cake pan with a diameter of 9 inches?

Solution

Problem 6 :

The diagram below shows one exterior wall of a house. The wall has a door that measures 3 ft. by 7 feet, and three windows which each measure 4 ft. by 4 ft. The wall (but not the doors or windows) will be covered with siding material. How much siding material is required?

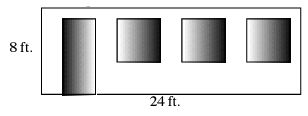
A. 192 sq. ft.   B. 155 sq. ft.   C. 123 sq. ft.    D. 261 sq. ft.

Solution

Problem 7 :

The figure below shows the plan for the a new county park. How many acres will the park occupy? (Use the estimate: 1 acre = 40,000 square feet.)

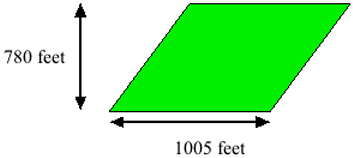
A. 6.53 acres        B. 391950 acres

C. 176.38 acres     D. 19.60 acres

Solution

Problem 8 :

Suppose that it costs $480 to build a rectangular wooden deck that measures 6 feet by 8 feet. Assuming that the cost of such an object depends upon its size (area), how much would it cost to build a similar deck measuring 18 feet by 24 feet?

A. $640     B. $1440     C. $1,920      D. $4,320

Solution

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.