BINOMIAL EXPANSION PRACTICE WORKSHEET

Binomial expansion for (x + a)ⁿ is,

nc₀xⁿa⁰ + nc₁x^n-1a¹ + nc₂x^n-2a² + .........+ nc_nx^n-na⁰⁽

If X is a set containing n elements, then we know that ⁿC_r is the number of subsets of X having exactly r elements. So by adding ⁿC_r for r = 0, 1, 2, . . . , n we get the number of subsets of X. So by using the above identity we see that a set of n elements has 2ⁿ subsets.

(1)  Expand (i) [2x² − (3/x)]³        Solution

(ii)  (2x² − 3√1 − x²)⁴  +  (2x² + 3√1 − x²)⁴  Solution 

(2)  Compute (i)  102⁴   (ii)  99⁴   (iii)  9⁷    Solution

(3) Using binomial theorem, indicate which of the following two number is larger: (1.01)^1000000, 10000.

Solution

(4)  Find the coefficient of x¹⁵ in  (x² + (1/x³))¹⁰  Solution

(5)  Find the coefficient of x⁶ and the coefficient of x² in  (x² - (1/x³))⁶   Solution 

(6)  Find the coefficient of x⁴ in the expansion of (1 + x³)⁵⁰(x² + 1/x)⁵.   Solution

(7)  Find the constant term of (2x³ - (1/3x²))⁵  Solution

(8)  Find the last two digits of the number 3⁶⁰⁰    Solution

(9)  If n is a positive integer, show that, 9ⁿ⁺¹ − 8n − 9 is always divisible by 64.   Solution

(10)  If n is an odd positive integer, prove that the coefficients of the middle terms in the expansion of (x + y)ⁿ are equal.              Solution

(11)  If n is a positive integer and r is a non negative integer, prove that the coefficients of x^r and x^n−r in the expansion of (1 + x)ⁿ are equal             Solution

(12)  If a and b are distinct integers, prove that a − b is a factor of aⁿ − bⁿ, whenever n is a positive integer. [Hint: write aⁿ = (a − b + b)ⁿ and expand]    Solution

(13)  In the binomial expansion of (a + b)ⁿ, the coefficients of the 4^th and 13^th terms are equal to each other, find n.      Solution

(14)  If the binomial coefficients of three consecutive terms in the expansion of (a + x)ⁿ are in the ratio 1 : 7 : 42, then find n.              Solution

(15)  In the binomial coefficients of (1 + x)ⁿ, the coefficients of the 5th, 6th and 7th terms are in AP. Find all values of n.    Solution

(16)  

Solution

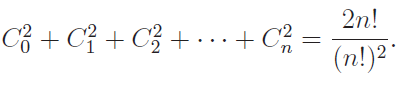
Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.