DIRECT VARIATION WORKSHEET

Problem 1-3 : Tell whether each equation represents a direct variation. If so, identify the constant of variation.

Problem 1 :

y  =  4x

Problem 2 :

-2x + 3y  =  0

Problem 3 :

3x + 2y  =  6

Problem 4-7 : Tell whether each relationship is a direct variation. Explain.

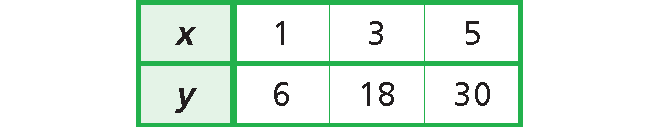
Problem 4 :

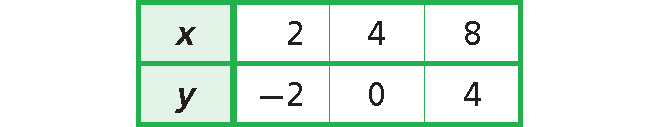
Problem 5 :

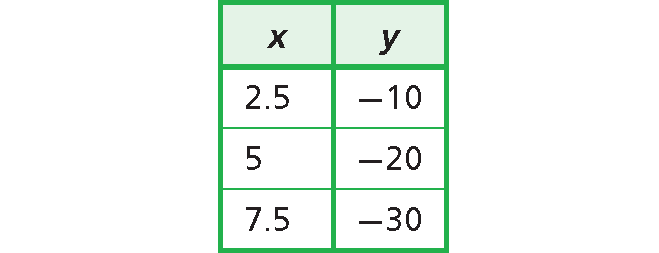
Problem 6 :

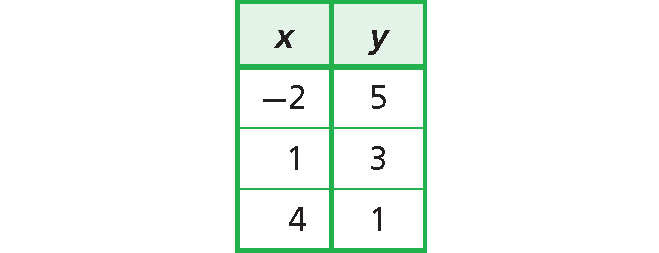
Problem 7 :

Problem 8 :

The value of y varies directly with x, and y = 8 when x = 2. Find y when x = 5.

Problem 9 :

The value of y varies directly with x, and y = 4.5 when x = 0.5. Find y when x = 10.

Problem 10 :

The three-toed sloth is an extremely slow animal. On the ground, it travels at a speed of about 6 feet per minute. Write a direct variation equation for the distance y a sloth will travel in x minutes. Then graph.

1. Answer : 

This equation represents a direct variation, because it is in the form y = kx. The constant of variation is 4.

2. Answer : 

-2x + 3y  =  0

Solve the equation for y.

Because -2x is added to 3y, add 2x to each side.

3y  =  2x

Because y is multiplied by 3, divide each side by 3.

3y/3  =  2x/3

y  =  (2/3)x

This equation represents a direct variation, because it can be written in the form y = kx. The constant of variation is 2/3.

3. Answer : 

3x + 2y  =  6

Solve the equation for y.

Because 3x is added to 2y, subtract 3x from each side. 

2y  =  -3x + 6

Because y is multiplied by 2, divide each side by 2.

2y/2  =  (-3x + 6)/2

y  =  -3x/2 + 6/2

y  =  -3x/2 + 3

This equation does not represent a direct variation, because it cannot be written in the form y = kx.

4. Answer : 

Write an equation that represents the relationship given in the table above. 

y  =  6x

Each y-value is 6 times the corresponding x-value.

This is a direct variation.

Because the equation y = 6x is in the form of y = kx, where k = 6.

5. Answer : 

Write an equation that represents the relationship given in the table above. 

y  =  x - 4

Each y-value is 4 less than the corresponding x-value.

This is a not direct variation. 

Because the equation y = x - 1 is not in the form of y = kx.

6. Answer : 

Write an equation that represents the relationship given in the table above. 

y  =  -4x

Each y-value is -4 times the corresponding x-value.

This is a direct variation. 

Because the equation y = -4x is not in the form of y = kx, where k = -4. 

7. Answer : 

From the table, we have the following ordered pairs.

(-2, 5), (1, 3) and (4, 1)

Plotting the above points in xy-plane, we get a straight line with slope -2/3 and y-intercept 11/3

So, the equation is

y  =  -2x/3 + 11/3

This is not a direct variation. 

Because the above equation is not in the form of y = kx. 

8. Answer :

It is given that y varies directly with x. 

Write the equation for a direct variation.

y  =  kx

Substitute 8 for y and 2 for x. 

8  =  k(2)

8  =  2k

Divide each side by 2.

8/2  =  2k/2

4  =  k

The equation is

y  =  4x

Find y, when x = 5.

y  =  4(5)

y  =  20

9. Answer :

It is given that y varies directly with x. 

Write the equation for a direct variation.

y  =  kx

Substitute 4.5 for y and 0.5 for x. 

4.5  =  k(0.5)

4.5  =  0.5k

Divide each side by 0.5.

4.5/0.5  =  0.5k/0.5

9  =  k

The equation is

y  =  9x

Find y, when x = 10.

y  =  9(10)

y  =  90

10. Answer : 

Step 1 :

Write a direct variation equation.

Step 2 : 

Choose values of x and generate ordered pairs.

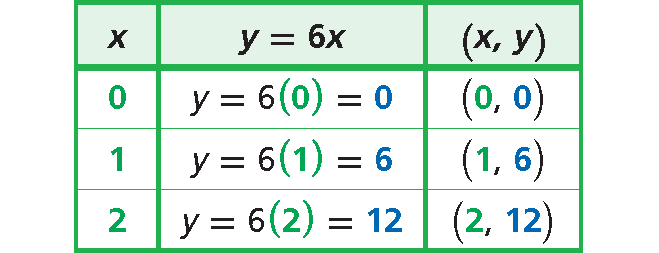
Step 3 : 

Graph the points and connect. 

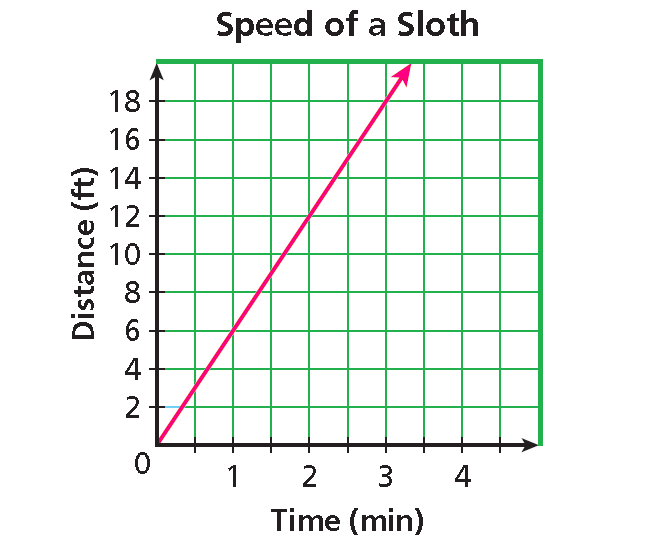
Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.