EXAMPLE PROBLEMS ON SURFACE AREA WITH COMBINED SOLIDS

Example 1 :

A play top is in the form of a hemisphere surmounted on a cone. The diameter of the hemisphere is 3.6 cm. The total height of the play top is 4.2 cm. Find its total surface area.

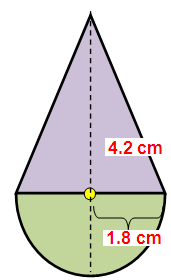
Solution :

Let r and h are radius and height of the cone.

diameter of the hemisphere  =  3.6 cm

radius of hemisphere (r)  =  3.6/2  =  1.8 cm

total height of the play top  =  4.2cm

radius of hemisphere + height of cone = 4.2

1.8 + h  =  4.2

h  =  4.2-1.8

h  =  2.4 cm

Now we are going to find slant height of cone

l²  =  r²+h²

=  (1.8)²+ (2.4)²

=  3.24 + 5.76

l²  =  9

L  =  √(3 ⋅ 3)

L  =  3 cm

To find the total surface area of the play top, we have to find the sum of curved surface area of hemisphere and curved surface area of cone.

Total surface area of play top = C.S.A of hemisphere + C.S.A of cone

=  2Πr²+Πrl

=  Πr(2r+l)

=  Π ⋅ (1.8) [2(1.8) + 3] 

=  Π ⋅ (1.8) (3.6+3) 

=  Π (1.8) (6.6)

=  11.88 Π cm²

Total surface area of the play top = 11.88 Π cm².

Example 2 :

A solid is in the shape of a cylinder surmounted on a hemisphere. If the diameter and the total height of the solid are 21 cm,25.5 cm respectively, then find its volume.

Solution :

Let r and h are radius and height of the cylinder.

diameter of the hemisphere  =  21 cm

radius of hemisphere (r)  =  (21)/2  =  10.5 cm

Total height of the solid shape  =  25.5 cm

radius of hemisphere + height of the cylinder  =  25.5

10.5 + h = 25.5

h  =  25.5-10.5

h  =  15 cm

To find the volume of the solid shape we have to find the sum of volume of hemisphere and volume of cylinder.

Volume of solid toy  =  Volume of hemisphere + Volume of cylinder 

=  (2/3) Πr³ + Π r²h

=   Πr²[(2/3) r + h]

=  (22/7) x (10.5)² [(2/3) 10.5 + 15] 

=  (22/7) ⋅ 10.5 ⋅ 10.5 (2(3.5)+15)

=  22 ⋅ 1.5 ⋅ 10.5 ⋅ (7+15)

=  7623 cm³

Volume of solid shape  =  7623 cm³.

Example 3 :

A capsule is in the shape of a cylinder with two hemispheres stuck to each of its ends. If the length of the entire capsule is 14 mm and diameter of the capsule is 5 mm, find its surface area.

Solution :

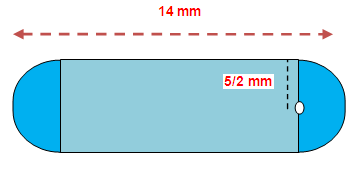
diameter of the hemisphere  =  5 mm

radius of hemisphere (r)  =  5/2  =  2.5 mm

total height of the solid  =  14 mm

2(radius of hemisphere)+height of cylinder  =  14

2 (5/2)+h  =  14

5+h  =  14

h  =  14-5

h  =  9 mm

Now we have to find total surface area of capsule. For that we have to find the sum of surface areas of two hemispheres and one cylinder.

Total surface area of capsule

=  2(CSA of hemisphere) + CSA of cylinder

=  2(2 Π r²)+2Πrh

=  2Πr(2r+h)

=  2⋅ (22/7)⋅ (2.5) [2(2.5)+9] 

=  (22/7) ⋅ 5⋅ (5+9)

=  (110/7) (14)

=  220 mm²

Total surface area of capsule = 220 mm².

Example 4 :

A tent is in the shape of right circular cylinder surmounted by a cone. The total height and diameter of the base are 13.5 m and 28 m. If the height of the cylindrical portion is 3 m, find the total surface area of the tent.

Solution :

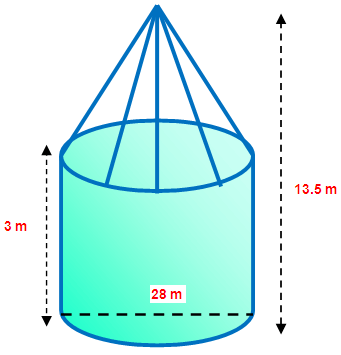
The total height of the tent  =  13.5 m

The diameter of the base of the tent  =  28 m

radius of the tent  =  14 m

Height of cylindrical portion  =  3 m

Total height of the tent  =  13.5 m

Height of cylinder + Height of cone  =  13.5

3+h  =  13.5

h  =  13.5-3

h  =  10.5 m

height of cylindrical portion is 10.5 m

l²  =  r²+h²

=  14²+10.5²  

=  196+110.25

l  =  √306.25

l  =  17.5

Total surface area of tent  =  CSA of cylinder + CSA area of cone

=  2Πrh + Πrl

=  Πr(2h+l)

=  (22/7) ⋅ 14 [2(3)+17.5]

=  22 ⋅ 2(6+17.5)

=  44 (23.5)

=  1034 cm³

Example 5 :

Using clay, a student made a right circular cone of height 48 cm and base radius 12 cm. Another student reshapes it in the form of a sphere. Find the radius of the sphere.

Solution :

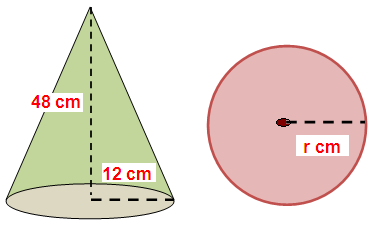
Height of the right circular cone (h)  =  48 cm

Radius of right circular cone (r)  =  12 cm

Volume of right circular cone  =  Volume of sphere

(1/3) Πr²h  =  (4/3) Πr³

(1/3) r²h  =  (4/3) r³

(1/3) (12)²(48)  =  (4/3) r³

(1/3) ⋅ 12 ⋅ 12 ⋅ 48  =  (4/3) r³

r³  =  (1/3) ⋅ 12 ⋅ 12 ⋅ 48 ⋅ (3/4)

r³  =  3 ⋅ 12 ⋅ 48 

r  =  ∛ (3⋅3⋅4⋅4⋅4⋅3)

r  =  12 cm

Radius of sphere is 12 cm.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.