FIND THE OTHER ROOTS OF THE POLYNOMIAL EQUATION OF DEGREE 6

Question 1 :

Find all zeros of the polynomial x⁶ − 3x⁵ − 5x⁴ + 22x³ − 39x² − 39x + 135, if it is known that 1 + 2i and √3 are two of its zeros.

Solution :

The four roots are 1 + 2i, 1 - 2i, √3 and -√3.

Quadratic equation whose roots are 1 + 2i and 1 - 2i.

Sum of roots  =  2

Product of roots  =  1 + 4  =  5

x² - 2x + 5

Sum of roots   =  0

Product of roots  =  -3

(x² - 3) is a factor.

(x² - 2x + 5) (x² - 3)  =  x⁴ - 3x² - 2x³ + 6x + 5x² - 15

  =  x⁴ - 2x³ + 2x² + 6x - 15

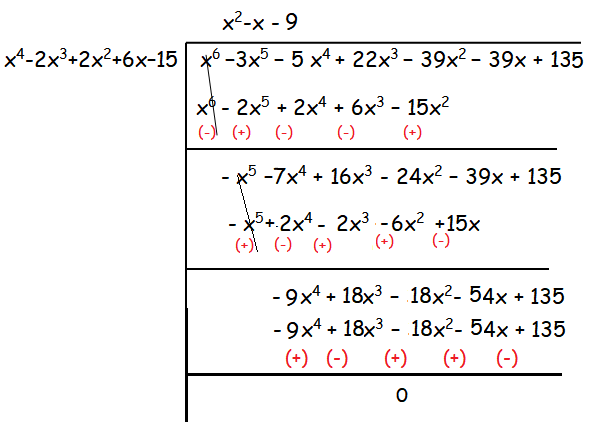
x² - x - 9  =  0

x = (-b ± √b² - 4ac)/2a

x = (1 ± √1 + 36)/2

x = (1 ± √37)/2

Hence the zeroes are 1 + 2i, 1 - 2i, √3, -√3, (1 ± √37)/2.

Question 2 :

Solve the cubic equation

(i) 2x³ − 9x² +10x = 3

Solution :

2x³ − 9x² +10x - 3  =  0

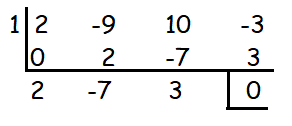
(x -1) is a factor

  =  2x² - 7x + 3  

  =  2x² - 1x - 6x + 3  

  =  x(2x - 1) - 3(2x - 1)

  =  (x - 3) (2x - 1)

(x - 1) (x - 3) (2x - 1)  =  0

x - 1  =  0, x - 3  =  0,  2x - 1  =  0

x  =  1, x  =  3, x  =  1/2

Hence the solutions are 1, 3 and 1/2.

(ii)  8x³ − 2x² − 7x + 3 = 0.

Solution :

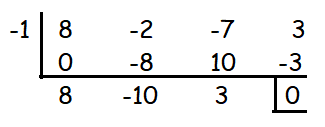
(x + 1) is a factor.

The other factors are 8x² - 10x + 3

  =  8x² - 12x + 2x + 3

  =  4x(2x - 3) -1(2x - 3)

  =  (4x - 1) (2x - 3)

By equating them to zero, we get 

x + 1  =  0, 4x - 1  =  0,  2x - 3  =  0

x  =  -1, x  =  1/4,  x  =  3/2

Hence the solutions are -1, 1/4 and 3/2.

Question 3 :

Solve the equation x⁴ −14x² + 45 = 0

Solution :

Let x²  =  t

t² − 14t + 45 = 0

t² − 9t - 5t + 45 = 0

t(t - 9) - 5(t - 9)  =  0

(t - 5) (t - 9)  =  0

t  =  5 and t  =  9

x²  =  5, x²  =  9

x  =  ±√5, x = ±3

Hence the solutions are √5, -√5, 3 and -3.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.