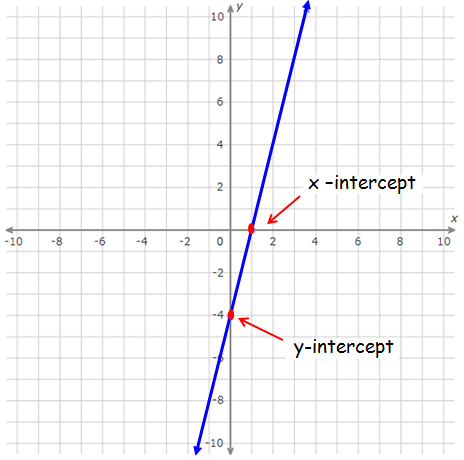
FIND X AND Y INTERCEPTS

X-intercept :

The x-intercept is where a line crosses the x-axis. To find the x-intercept, we have to put y = 0 and solve the value for x.

Y-intercept :

The y-intercept is the point where the line crosses the y-axis. To find the y-intercept, we have to put x = 0 and solve the value for y.

Example 1 :

Find the x and y intercepts of the following equation.

2x + 3y  =  12

Solution :

X-intercept :

To find x-intercept, we have to put y  =  0

2x + 3y  =  12

2x + 3(0)  =  12 ==> 2x + 0 = 12 ==> 2x  =  12

Divide by 2 on both sides, we get

x  =  6

Y-intercept :

To find y-intercept, we have to put x  =  0

2(0) + 3y  =  12 ==> 3y  =  12

Divide by 3 on both sides, we get

y  =  4

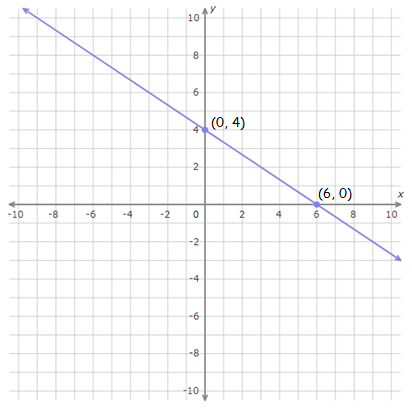
Hence x and y intercepts are 6 and 4 respectively.

So, we have to plot the points (6, 0) and (0, 4) and draw the line which connects the above points.

Example 2 :

Find the x and y intercepts of the following equation.

5x + y - 10  =  0

Solution :

X-intercept :

To find x-intercept, we have to put y  =  0

5x - y - 10  =  0

5x - 0 - 10  =  0 ==> 5x  =  10

Divide by 5 on both sides, we get

x  =  2

Y-intercept :

To find y-intercept, we have to put x  =  0

5(0) - y - 10 ==> -y - 10  =  0

Add 10 on both sides 

-y  =  10 ==> y  =  -10

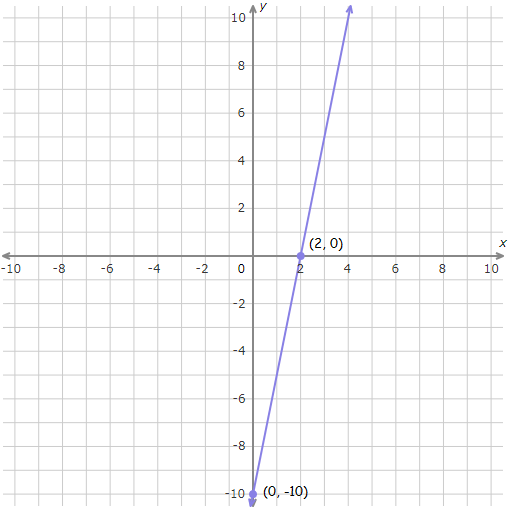
Hence x and y intercepts are 2 and -10 respectively.

So, we have to plot the points (2, 0) and (0, -10) and draw  the line which connects the above points.

Example 3 :

Find the x and y intercepts of the following equation.

y  =  4x - 4

Solution :

X-intercept :

To find x-intercept, we have to put y  =  0

y  =  4x - 4

4x - 4  =  0 ==> 4x  =  4

Divide by 4 on both sides, we get

x  =  1

Y-intercept :

To find y-intercept, we have to put x  =  0

y  =  4(0) - 4 ==> y  =  -4 

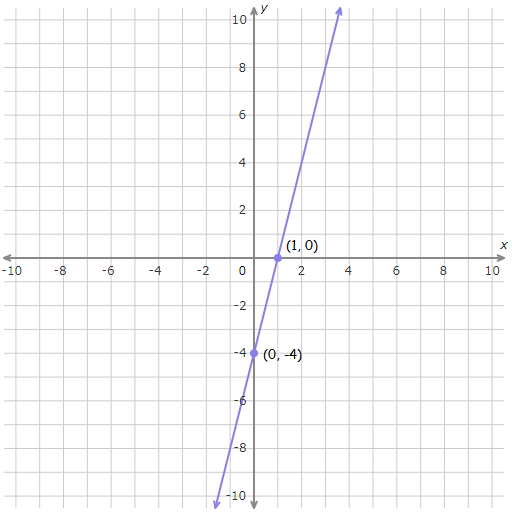
Hence x and y intercepts are 1 and -4 respectively.

So we have to plot the points (1, 0) and (0, -4) and draw the line which connects the above points.

Example 4 :

Find the x and y intercepts of the following equation.

y  =  -x/2 - 2

Solution :

X-intercept :

x-intercept, put y  =  0

0  =  (-x/2) - 2

Add 2 on both sides

2  =  -x/2

Multiply 2 on both sides

4  =  -x ==> x  = -4

Y-intercept :

y-intercept, put x  =  0

y  =  (-0/2) - 2

y  =  -2

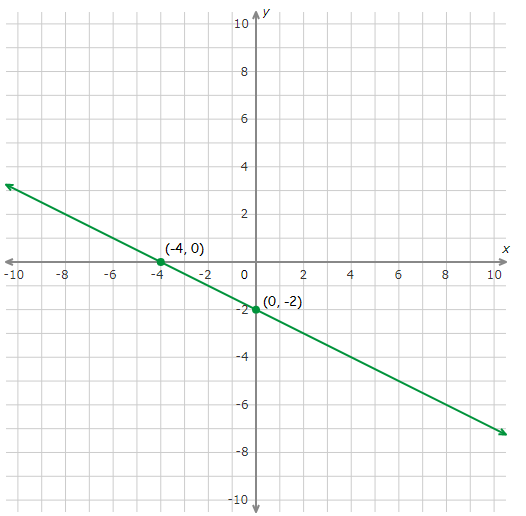
Hence x and y-intercepts are -4 and -2

So we have to plot the points (-4, 0) and (0, -2) and draw the line which connects the above points.

Example 5 :

Find the x intercepts of the following parabola.

y  =  x² - 3 x

Solution :

X-intercept :

x-intercept, put y = 0

0  =  x² - 3 x

x² - 3 x  =  0

x (x - 3)  =  0

 x  =  0        x - 3  =  0 

                    x  =  3

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.