FINDING THE PERIMETER AND AREA OF A RECTANGLE

Perimeter :

Perimeter is a path that surrounds a rectangle. The term may be used either for the path or its length it can be thought of as the length of the outline of the rectangle.

Area :

Area of a rectangle is defined as the space occupied by the rectangle shaped object on a flat surface. The area of a shape can be measured by comparing the shape to squares of a fixed size.

Formulas for Perimeter and Area of a Rectangle

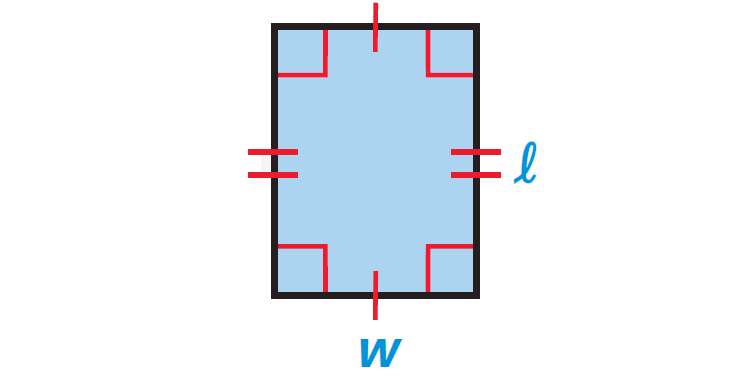
length = l and width = w

Perimeter = 2l + 2w

Area = lw

The measurements of perimeter use units such as centimeters, meters, kilometers, inches, feet, yards, and miles. The measurements of area use units such as square centimeters (cm²), square meters(m²), and so on.

Example 1 :

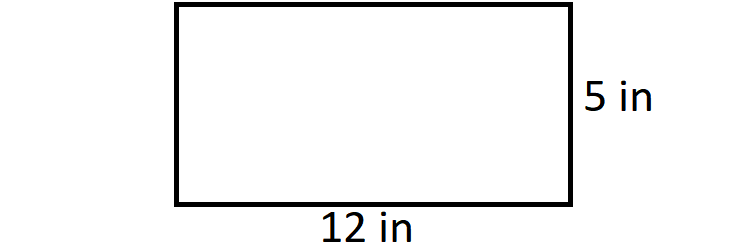
Find the perimeter and area of a rectangle of length 12 inches and width 5 inches.

Solution :

Draw a rectangle and label the length and width.

So, the perimeter is 34 inches and the area is 60 square inches.

Example 2 :

The area of a rectangle is 56 square inches. If the length is 8 inches, find the width of the rectangle.

Solution :

Given : Area of the rectangle = 56 sq. in.

lw = 56

Substitute l = 8.

8w = 56

Divide both sides by 8.

w = 7 in

Example 3 :

The perimeter of a rectangle is 30 cm. The length is 3 more than twice the width. Find the length and width of the rectangle.

Solution :

Step 1 :

Let w  =  x.

Then, l = 2x + 3.

Step 2 :

Given : Perimeter of the rectangle = 30 cm.

2l + 2w = 30

Step 3 :

Substitute w = x and l = 2x + 3.

2(2x + 3) + 2x = 30

4x + 6 + 2x = 30

6x + 6 = 30

Subtract 6 from both sides.

6x = 24

Divide both sides by 6.

x = 4

Width = x = 4.

Length = 2x + 3 = 2(4) + 3 = 11.

So, the length is 11 cm and width is 4 cm.

Example 4 :

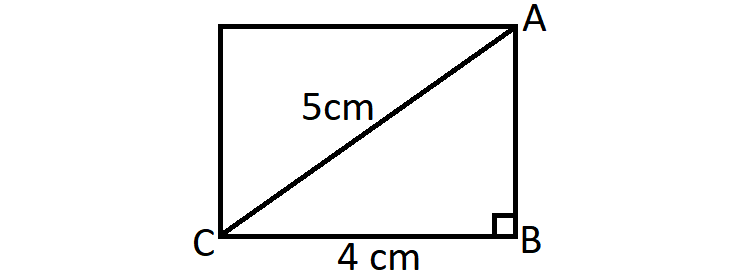
The diagonal of a rectangle is 5 cm and one of its sides is 4 cm. Find its area.

Solution :

Step 1 :

Let us assume that one of the sides given is length.

Then, l = 4 cm.

Step 2 :

Draw a rectangle and label the diagonal and length.

Step 3 :

To find area of a rectangle, we need the measures of length and width. We know the length and it is 4 cm. We have to find the width.

Step 4 :

In the rectangle above, let us consider the right triangle ABC and apply Pythagorean theorem.

AB² + BC²  =  AC²

Substitute BC = 4 and AC = 6.

w² + 4² = 5²

w² + 16 = 25

Subtract 16 from both the sides.

w² = 9

w² = 3²

Remove the exponent 2 on both sides.

w = 3 cm

Step 5 :

Area of the rectangle = lw

Substitute l = 4 and w = 3.

= 4 ⋅ 3

= 12 cm² 

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.