GRAPH AND SOLVE QUADRATIC INEQUALITIES WORKSHEET
Problem 1 :
Graph and solve for x :
x² - 7x + 6 > 0
Problem 2 :
Graph and solve for x :
-x² + 3x - 2 > 0
Problem 3 :
Graph and solve for x :
4x² - 25 ≥ 0
Problem 4 :
Graph and solve for x :
2x² − 12x + 50 ≤ 0

Solutions
Problem 1 :
Graph and solve for x :
x² - 7x + 6 > 0
Solution :
x² - 7x + 6 > 0
(x - 1)(x - 6) > 0
On equating the factors to zero, we see that x = 1, x = 6 are the roots of the quadratic equation. Plotting these roots on real line and marking positive and negative alternatively from the right most part we obtain the corresponding number line as
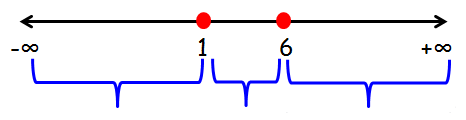
If we plot these points on the number line, we will get intervals (-∞, 1) (1, 6) (6, ∞).
|
From (-∞, 1) let us take -1 |
(-1 − 1) (-1 − 6) > 0 -2(-7) > 0 14 > 0 True |
|
From (1, 6) let us take 4 |
(4 − 1) (4 − 6) > 0 3(-2) > 0 -6 > 0 False |
|
From (6, ∞) let us take 7 |
(7 − 1) (7 − 6) > 0 6(1) > 0 6 > 0 True |
Hence, the solution set is
(− ∞, 1) ∪ (6, ∞)
Problem 2 :
Graph and solve for x :
-x² + 3x - 2 > 0
Solution :
-x² + 3x - 2 > 0
Multiplying by negative sign on both sides
x²- 3x + 2 < 0
(x − 1) (x − 2) < 0
x - 1 = 0 x - 2 = 0
x = 1 and x = 2
On equating the factors to zero, we see that x = 1, x = 2 are the roots of the quadratic equation. Plotting these roots on real line and marking positive and negative alternatively from the right most part we obtain the corresponding number line as
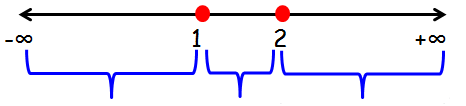
If we plot these points on the number line, we will get intervals (-∞, 1) (1, 2) (2, ∞).
|
From (-∞, 1) let us take -1 |
(x − 1) (x − 2) < 0 (-1 − 1) (-1 − 2) < 0 (-2)(-3) > 0 6 < 0 False |
|
From (1, 2) let us take 1.5 |
(x − 1) (x − 2) < 0 (1.5 − 1) (1.5 − 2) < 0 (-0.5)(-0.5) > 0 0.25 < 0 True |
|
From (2, ∞) let us take 7 |
(7 − 1) (7 − 6) > 0 6(1) > 0 6 > 0 False |
Hence, the solution set is
(1, 2)
Problem 3 :
Graph and solve for x :
4x² - 25 ≥ 0
Solution :
4x² - 25 ≥ 0
(2x)² - 5² ≥ 0
(2x - 5) (2x + 5) ≥ 0
2x - 5 ≥ 0 (or) 2x + 5 ≥ 0
x = 5/2 (or) x = -5/2
On equating the factors to zero, we see that x = 5/2, x = -5/2 are the roots of the quadratic equation. Plotting these roots on real line and marking positive and negative alternatively from the right most part we obtain the corresponding number line as
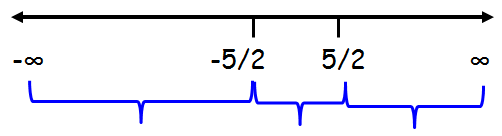
If we plot these points on the number line, we will get intervals (-∞, -5/2) (-5/2, 5/2) (5/2, ∞).Graph solutions to quadratic inequalities
|
From (-∞, -5/2) let us take -3 |
(2x - 5) (2x + 5) ≥ 0 (-6 − 5) (-6 + 5) < 0 (-11)(-1) > 0 11 > 0 True |
|
From (-5/2, 5/2) let us take 0 |
(2x - 5) (2x + 5) ≥ 0 (0− 5) (0 + 5) < 0 (-5)(5) > 0 -25 > 0 False |
|
From (5/2, ∞) let us take 4 |
(2x - 5) (2x + 5) ≥ 0 (8 − 5) (8 + 5) < 0 (3)(13) > 0 39 > 0 True |
Hence, the solution set is
(-∞, -5/2) U (5/2, ∞)
Problem 4 :
Graph and solve for x :
2x² − 12x + 50 ≤ 0
Solution :
2x² − 12x + 50 ≤ 0
2(x²− 6x + 25) ≤ 0
x² − 6x + 25 ≤ 0
(x²− 6x + 9) + 25 − 9 ≤ 0
(x − 3)² + 16 ≤ 0
This is not true for any real value of x.
So, the given quadratic inequality has no solution.
Kindly mail your feedback to v4formath@gmail.com
We always appreciate your feedback.
©All rights reserved. onlinemath4all.com
Recent Articles
-
AP Calculus AB Problems with Solutions (Part - 10)
Feb 11, 25 11:15 AM
AP Calculus AB Problems with Solutions (Part - 10) -
AP Calculus AB Problems with Solutions (Part - 11)
Feb 11, 25 11:09 AM
AP Calculus AB Problems with Solutions (Part - 11) -
AP Calculus AB Problems with Solutions (Part - 9)
Feb 10, 25 06:02 PM
AP Calculus AB Problems with Solutions (Part - 9)