HOW TO FIND DOMAIN AND RANGE OF A MAPPING DIAGRAM

Example 1 :

Examine whether the following mapping diagram represents function or not, find its domain, range and co-domain.

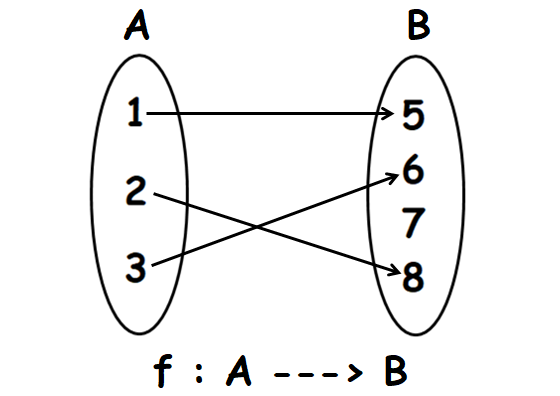
Solution :

Every element of A is associated with exactly one element in B. So, it is function.

Domain  =  {1, 2, 3}

Range  =  {5, 6, 8}

Co-domain  =  {5, 6, 7, 8} 

Example 2 :

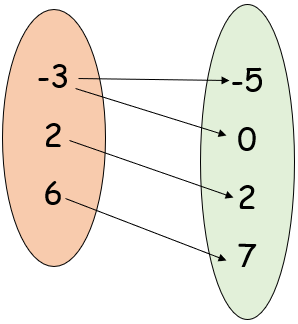
Solution :

By observing the mapping, it is clear it is not a function. Because -3 is having  more than one image.

Domain  =  {-3, 2, 6}

Range  =  {-5, 0, 2, 7}

Co domain  =  {-5, 0, 2, 7}

Example 3 :

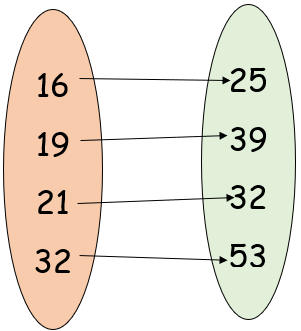
Solution :

By observing the mapping, it is a function. 

Domain  =  {16, 19, 21, 22}

Range  =  {25, 39, 32, 53}

Co domain  =  {25, 39, 32, 53}

Example 4 :

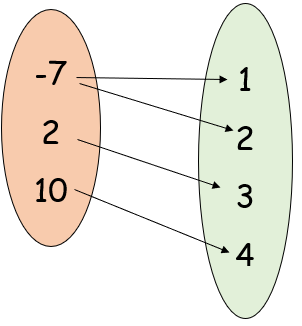
Solution :

By observing the mapping, it is not a function. Because -7 is having more than one image.

Domain  =  {-7, 2, 10}

Range  =  {1, 2, 3, 4}

Co domain  =  {1, 2, 3, 4}

Write the ordered pairs associated with each mapping diagram. Then state if the relation is a function.

Example 5 :

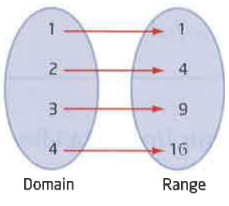
Solution :

The ordered pairs which is shown in the above mapping diagram,

(1, 1) (2, 4) (3, 9) and (4, 16)

This relation represents a function.

Example 6 :

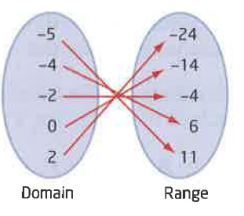
Solution :

The ordered pairs which is shown in the above mapping diagram,

(-5, 11) (-4, 6) (-2, -4) (0, -14) (2, -24)

This relation represents a function.

Example 7 :

If (4x + 2, 2y – 3) = (4, 3) find the values of x, y?

Solution :

(4x + 2, 2y – 3) = (4, 3)

Comparing the corresponding terms, we get

So, the values of x and y are 1/2 and 3 respectively.

Show each set of data in a mapping diagram. Then state if the relation is a function. 

Example 8 :

{(-5, 6) (-4, 9) (-3, 1) (-5, -6) (1, -2) (3, 8) and (8, 8)}

Solution :

By representing the set of ordered pairs in arrow diagram, we get

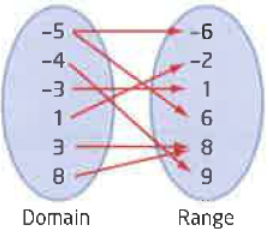
This relation is not a function.

Write the Domain and Range for the following Relations?

Example 9 :

R₁ = {(6, 2); (6, 5); (3, 5); (0, 8); (7, 3)}

Solution :

Domain = {0, 3, 6, 7}

Range = {2, 3, 5, 8}

Example 10 :

 R₂ = {(x, 2); (y, 3); (z, 2); (u, 6)}

Solution :

Domain = {x, y, z, u}

Range = {2, 3, 6}

Example 11 :

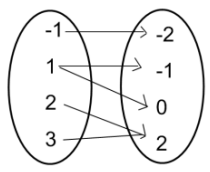
a) Domain 

b) Range

c) Find f(3)

Solution :

a) Domain = {-1, 1, 2, 3}

b) Range = {-2, -1, 0, 2}

1 is associated with more than one term, then it is not a function.

c) f(3) = 2

Example 12 :

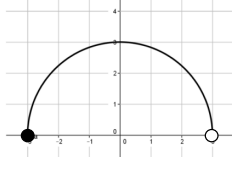
a) Domain 

b) Range

c) Find f(0)

Solution :

a) Domain = -3 ≤ x < 3

b) Range = 0 ≤ y ≤ 3

Using vertical line test, the vertical line is intersecting the graph at one point maximum. Then this relationship represents the function.

c) f(0) = 3

Determine the independent and dependent variable for each function.

Example 13 :

The air pressure inside a tire increases with the temperature.

Solution :

Temperature is the independent variable and air pressure is the dependent variable.

Example 14 :

The Spanish classes are having a fiesta lunch. Each student that attends is to bring a Spanish side dish or dessert. The more students that attend, the more food there will be

Solution :

• Number if students attend is independent variable 
• number of food items is dependent variable.

Example 15 :

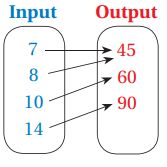
The table shows the number of songs played by four bands at a festival and the amount of time each band played.

Use the table to draw a mapping diagram. Let the number of songs played be the inputs and the times onstage be the outputs.

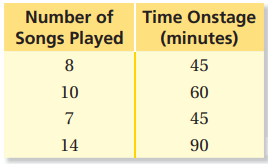
Solution :

Let the number of songs played be the inputs and the times onstage be the outputs.

Inputs: 7, 8, 10, 14

Outputs: 45, 60, 90

Example 16 :

Describe the pattern in the mapping diagram. Copy and complete the diagram.

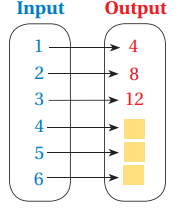
Solution :

By observing the inputs and outputs, every input is multiplied by 4.

When,

• input = 1, output = 4
• input = 2, output = 8
• input = 3, output = 12
  
• input = 4, output = 16
  
• input = 5, output = 20
  
• input = 6, output = 24

Example 17 :

A store sells previously viewed movies. The table shows the cost of buying 1, 2, 3, or 4 movies.

a. Use the table to draw a mapping diagram.

b. Describe the pattern. How does the cost per movie change as you buy more movies?

Solution :

a) Mapping diagram :

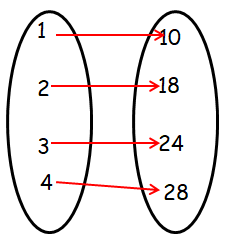
b) When number of tickets = 1, the amount paid = $10

number of tickets = 2, the amount paid = $18

number of tickets = 3, the amount paid = $24

number of tickets = 4, the amount paid = $28

• Difference between 18 and 10 is 8
• Difference between 24 and 18 is 6
• Difference between 28 and 24 is 4

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.