HOW TO FIND THE ORDER OF PRODUCT OF TWO MATRICES

How to Find the Order of Product of Two Matrices ?

Here we are going to see how to find the order of product of two matrices.

Question 1 :

Find the order of the product matrix AB if

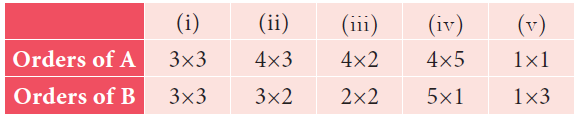
Solution :

(i)  Order of A is 3 x 3, order of B is 3 x 3.

The order of the matrix AB is 3 x 3.

(ii)  Order of A is 4 x 3, order of B is 3 x 2.

The order of the matrix AB is 4 x 2.

(iii)  Order of A is 4 x 2, order of B is 2 x 2.

The order of the matrix AB is 4 x 2.

(iv)  Order of A is 4 x 5, order of B is 5 x 1.

The order of the matrix AB is 4 x 1.

(v)  Order of A is 1 x 1, order of B is 1 x 3.

The order of the matrix AB is 1 x 3.

Question 2 :

If A is of order p x q and B is of order q x r what is the order of AB and BA?

Solution :

(p x q) (q x r)  =  p x r

The order of matrix AB is p x r.

(q x r) (p x q)   =  p x r

The product of matrices B and A is not possible.

Hence it is not defined.

Question 3 :

A has ‘a’ rows and ‘a + 3 ’ columns. B has ‘b’ rows and ‘17–b’ columns, and if both products AB and BA exist, find a, b?

Solution :

Number of rows of A = a 

Number of columns of A = a + 3

Number of rows of B = b

Number of columns of A = 17 - b

Since the product of matrices A and B is possible,

[a x (a + 3)] [b x (17 - b)]  

a + 3  =  b

a - b  =  -3  -----(1)

Since the product of matrices B and A is possible,

[b x (17 - b)]  [a x (a + 3)] 

17 - b  =  a

a + b  =  17 ----(2)

(1) + (2)

2a  =  14

a  =  7

By applying the value of a in (1), we get 

7 - b = -3

-b  =  -3 - 7

-b  =  -10

b = 10

After having gone through the stuff given above, we hope that the students would have understood, "How to Find the Order of Product of Two Matrices". 

Apart from the stuff given in this section "How to Find the Order of Product of Two Matrices", if you need any other stuff in math, please use our google custom search here.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.