HOW TO FIND THE TRISECTION POINTS OF A LINE

Here we are going to see how to find the trisection points of the line segment joining the given points.

Trisection points means the points  which exactly divides the line segment into three equal parts.

Let us look into some example problems to understand the above concept.

Example 1 :

Find the points of trisection of the line segment joining (4,- 1) and (-2,- 3).

Solution :

Let A(4,-1) and B(-2,-3) be the given points.

Let P(x,y) and Q(a,b) be the points of trisection of AB so that AP = PQ = QB

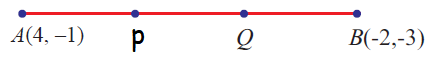
Hence P divides AB internally in the ratio 1 : 2 and Q divides AB internally in the ratio 2 : 1

By the section formula, the required points are

AP = 1

PQ = 1

QB = 1

Section formula internally = (lx₂ + mx₁)/(l + m) , (ly₂ + my₁)/(l + m)

P divides the line segment in the ratio 1:2

l = 1      m = 2    

A(4,-1) and B(-2,-3)

    =  [(1(-2) + 2(4)]/(1+2) , [(1(-3) + 2(-1)]/(1+2)

    =  (-2+8)/3 , (-3-2)/3

   =  6/3 , -5/3

  =  P (2 , -5/3)

Q divides the line segment in the ratio 2:1

l = 2      m = 1    

    =  [(2(-2) + 1(4)]/(2+1) , [(2(-3) + 1(-1)]/(2+1)

    =  (-4+4)/3 , (-6-1)/3

   =  0/3 , -7/3

  =  Q (0 , -7/3)

Example 2 :

Find the points of trisection of the line segment joining the points A (2 , -2) and B (-7 , 4).

Solution :

Let P and Q are the points of the trisection of the line segment joining the points A and B

Here AP = PQ = QB   

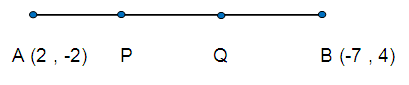
 AP = 1

PQ = 1

QB = 1

Section formula internally = (Lx₂ + mx₁)/(L + m) , (Ly₂ + my₁)/(L + m)

P divides the line segment in the ratio 1:2

L = 1      m = 2    

  =  [(1(-7)) + 2(2)]/(1+2) , [1(4) + (2(-2)]/(1+2)

  =  (-7 + 4)/3 , (4 - 4)/3

  =  -3/3 , 0/3

  =  P (-1 , 0)

Q divides the line segment in the ratio 2:1

L = 2      m = 1    

   = [(2(-7)) + 1(2)]/(2+1) , [2(4) + 1(-2)]/(2+1)

   = (-14 + 2)/3 , (8 - 2)/3

   =  -12/3 , 6/3

   =  Q (-4 , 2)

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.