LINE PASSING THROUGH LINE OF INTERSECTION OF THE GIVEN LINES

Question 1 :

Find the equation of a straight line joining the point of intersection of 3x + y + 2 = 0 and x − 2y − 4 = 0 to the point of intersection of 7x − 3y = −12 and 2y = x + 3

Solution :

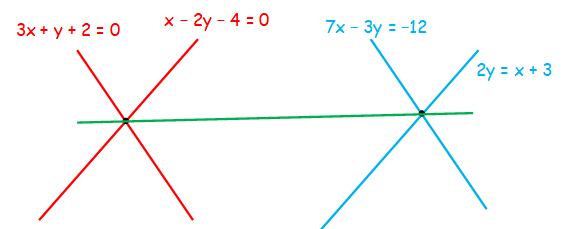
3x + y + 2 = 0 -----(1)

x − 2y − 4 = 0 -----(2)

2(1) + (2)

6x + 2y + 4  =  0

  x - 2y - 4   =  0

------------------------

7x  =  0

x  =  0/7

x  =  0

By applying the value of x in (1), we get 

3(0) + y + 2  =  0

y  =  -2

Point of intersection of the first two lines is (0, -2)

 7x − 3y = −12 -------(3) 

 2y = x + 3

 x - 2y  =  -3 -------(4)  

2(3) - 3(4)

14x - 6y  =  -24

3x - 6y  =  -9

(-)    (+)   (+)

--------------------

                                     11x  =   -15

x  =  -15/11

By applying x = -15/11 in (4), we get 

(-15/11) - 2y  =  -3

-2y  =  -3 + (15/11)

-2y  =  (-33 + 15)/11

-2y  =  (-33 + 15)/11

-2y  =  -18/11

y = 9/11

Point of intersection of other set of lines is (-15/11, 9/11).

Now, we have to find the equation o the line passing through the points (0, -2) and (-15/11, 9/11).

(y - y₁)/(y₂ - y₁)  =  (x - x₁)/(x₂ - x₁)

(y + 2)/((9/11) + 2)  =  (x - 0)/(-15/11 - 0)

(y + 2)/(31/11)  =  (x - 0)/(-15/11)

-15(y + 2)  =  31(x)

-15y - 30  =  31x

31x + 15y + 30  =  0

Question 2 :

Find the equation of a straight line through the point of intersection of the lines 8x + 3y = 18, 4x + 5y = 9 and bisecting the line segment joining the points (5,–4) and (–7,6).

Solution :

8x + 3y = 18 ----(1)

4x + 5y = 9  ----(2)

5(1) - 3(2)

40x + 15y = 90

12x + 15y  =  27

(-)     (-)     (-)

--------------------

28x  =  63

x  =  63/28

By applying the value of x in (1), we get 

8(63/28) + 3y  =  18

3y  =  18 - (126/7)

3y  =  (126-126)/7

y  =  0 

Point of intersection of the given lines is (63/28, 0). 

(5,–4) and (–7,6)

Midpoint  =  (5 - 7)/2, (-4 + 6)/2

   =  -2/2, 2/2

  =  (-1, 1)

Equation of the line passing through the points (-1, 1) and (63/28, 0)

(y - y₁)/(y₂ - y₁)  =  (x - x₁)/(x₂ - x₁)

(y - 1)/(0 - 1)  =  (x + 1)/((63/28) + 1)

(y - 1)/(- 1)  =  (x + 1)/(91/28)

91(y - 1)  =  -28(x + 1)

91y - 91  =  -28x - 28

28x + 91y - 91 + 28  =  0

28x + 91y - 63  =  0

Dividing the entire equation by 7, we get

4x + 13y - 9  =  0

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.