MODELING MIXED NUMBER DIVISION

For some real-world problems, we may need to divide a mixed number by fraction or mixed number or whole numbers. 

Let us see how division of mixed numbers can be modeled through some examples.

Example 1 : 

If 1/4 cup of rice is used to make each sushi roll, how many sushi rolls can be made using 2 1/2 cups of rice ?

Solution : 

To find the number of sushi rolls that can be made, we need to determine how many fourths are in 2 1/2 . 

Since 1/4 cup of rice is used to make each sushi roll, in the diagram given below, 2 1/2 is divided into fourths.

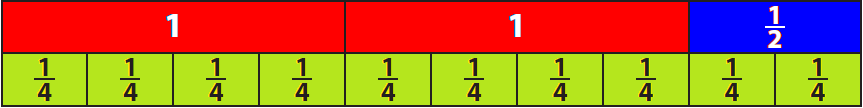
In 1 cup, we have four 1/4 cups of rice. 

But, we have 2 1/2 cups of rice.

So, we have to count the number of 1/4 cups in 2 1/2 cups in the above diagram. 

And there are ten 1/4 cups in 1 cup of rice. 

Hence, we can make 10 sushi rolls from 2 1/2 cups of rice.

Example 2 : 

We have 1 1/4 pounds of cheese. And we are going to pack this 1 1/4 pounds of cheese in two cans where each can has the capacity of 5/8 pound. In how many cans can we fill 1 1/4 pounds of cheese ?

Solution : 

To find the number of cans required, let us use the diagram given below. 

The diagram below represents 2 pounds and it is divided into quarters and into eighths.

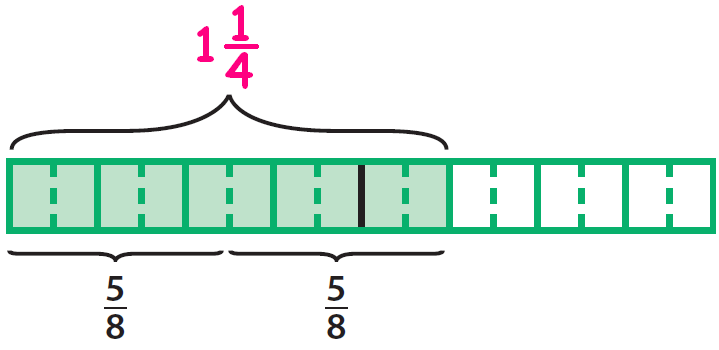
We have to fill 1 1/4 pounds of cheese in two cans where each can has the capacity of 5/8 pound.

So, we have to count the number of 5/8 pounds in 1 1/4 pounds. 

And there are two 5/8 pounds in 1 1/4 pounds of cheese.  

Hence, we can fill 1 1/4 pounds of cheese in two cans. 

Using Reciprocal to Divide Mixed Numbers - Steps

Step 1 :

When we divide a mixed number by fraction or another mixed number or whole number, first we have to convert the mixed numbers into fractions. 

Step 2 :

Change the division sign as multiplication.

Step 3 :

Take reciprocal of the second number.

Step 3 :

Multiply the two numbers.

Example 3 : 

Divide  3 2/5  by  6/7 

Solution : 

Using the method explained above, we have 

 3 2/5 ÷ 6/7  =  17/5 ÷ 7/6

3 2/5 ÷ 6/7  =  (17/5) x (6/7)

3 2/5 ÷ 6/7  =  (17x6) / (5x7)

3 2/5 ÷ 6/7  =  102/35

3 2/5 ÷ 6/7  =  2 32/35

Example 4 :

Divide  2 3/5  by  3

Solution : 

Using the method explained above, we have 

2 3/5 ÷ 3  =  13/5 ÷ 3

2 3/5 ÷ 3  =  (13/5) x (1/3)

2 3/5 ÷ 3  =  (13x1) x (5x3)

2 3/5 ÷ 3  =  13 / 15

Example 5 :

Divide  1 1/2  by  2 3/5

Solution : 

Using the method explained above, we have 

1 1/2 ÷ 2 3/5  =  3/2 ÷ 13/5

1 1/2 ÷ 2 3/5  =  3/2 x 5/13

1 1/2 ÷ 2 3/5  =  (3x5) /  (2x13)

1 1/2 ÷ 2 3/5  =  15/26

Example 6 :

One pizza can be made in 1/2 hour. How many pizzas can be made in 2 1/2 hours ?

Solution : 

Time taken to make one pizza  =  1/2 hour  

No. of pizzas made in 2 1/2 hours  =  2 1/2 ÷ 1/2

No. of pizzas made in 2 1/2 hours  =  5/2 ÷ 1/2

No. of pizzas made in 2 1/2 hours  =  5/2 x 2/1

No. of pizzas made in 2 1/2 hours  =  (5x2) / (2x1)

No. of pizzas made in 2 1/2 hours  =  5

Example 7 :

David eats 1 1/8 pizzas and divides into two equal parts for his two kids. What is the part of the pizza will each kid receive ?

Solution : 

1 1/8 pizzas is divided in to two equal parts for his two kids. 

So, amount of part pizza received by each kid is 

=  1 1/8 ÷ 2

=  9/8 ÷ 2

=  9/8 x 1/2

=  (9x1) / (8x2)

=  9/16

Hence, the amount of pizza received by each kid is 9/16.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.