MULTIPLYING DECIMALS by 10 100 and 1000

Problem 1 :

Multiply 3.723 by 10.

Solution :

We multiply by 3.723 by 10. So, we have to move the decimal point one digit to the right. 

Then, 

3.723 x 10 = 37.23

Multiplying 3.723 by 10, we get 37.23.

Problem 2 :

Multiply 3.723 by 100.

Solution :

We multiply by 3.723 by 100. So, we have to move the decimal point two digits to the right. 

Then, 

3.723 x 100 = 372.3

Multiplying 3.723 by 100, we get 372.3.

Problem 3 :

Multiply 3.723 by 1000.

Solution :

We multiply by 3.723 by 1000. So, we have to move the decimal point three digits to the right. 

Then, 

3.723 x 1000 = 3723

Multiplying 3.723 by 1000, we get 3723.

Problem 4 :

Multiply 5.628 by 10000.

Solution :

We multiply by 5.628 by 10000. So, we have to move the decimal point four digits to the right. But, in 5.628, there are only three digits after the decimal point. Add one zero at the end to get four digits after the decimal point.  

Then, 

5.628 x 10000 = 5.6280 x 10000

= 56280

Multiplying 5.628 by 10000, we get 56280.

Problem 5 :

Multiply 0.05 by 10000.

Solution :

We multiply by 0.05 by 10000. So, we have to move the decimal point four digits to the right. But, in 0.05, there are only two digits after the decimal point. Add two zeros at the end to get four digits after the decimal point.  

Then, 

0.05 x 10000 = 0.0500 x 10000

= 500

Multiplying 0.05 by 10000, we get 500.

Problem 6 :

Multiply 8.2567 by 100.

Solution :

We multiply by 8.2567 by 100. So, we have to move the decimal point four digits to the right.

Then, 

8.2567 x 100 = 825.67

Multiplying 8.2567 by 100, we get 825.67.

Problem 7 :

The price of a fruit is $3.52 per lb. Find the cost of 10 lbs. of fruits. 

Solution :

Given : Cost of 1 lb. of fruit = $3.52.

Then, the cost of 10 lbs. of fruits is

= 10 x 3.52

We multiply by 3.52 by 10. So, we have to move the decimal point one digit to the right.

= 35.2

So, the cost of 10 lbs. of fruits is $35.2

Problem 8 :

A person walks regularly in the morning. If the speed of his walking is 43.26 ft. per minute, Find the distance covered by him in  100 minutes. 

Solution :

Given : Speed is 43.26 ft. per minute.

Formula to find distance :

Distance = Speed x Time

To know the distance covered in 100 minutes, we have to substitute time = 100 and speed = 43.26.

Distance = 43.26 x 100 

We multiply by 43.26 by 100. So, we have to move the decimal point two digits to the right.

Distance = 4326 ft.

Problem 9 :

The speed of a car is 54.72 miles per hour. At the same rate, find the distance covered by the car in 10 minutes.

Solution :

Given : Speed of the car is 54.72 miles per hour.

That is, distance covered by the car in one hour is 54.72 miles.

1 hour ----> 54.72 miles

60 minutes ----> 54.72 miles

1 minute ----> (54.72/60) miles

1 minute ----> 0.912 miles

Distance covered by the car in one minute is 0.912 miles.

Distance covered by the car in 10 minutes :

= 0.912 x 10

We multiply by 0.912 by 10. So, we have to move the decimal point one digit to the right.

= 9.12 miles

Problem 10 :

One-thousandth of a certain number is 0.003. What is the number?

Solution :

Given : One-thousandth of a certain number is 0.003

Let a be the number.

Then, from the given information, we get

(1/1000) x a = 0.003

Multiplying both sides by 1000, we get

a = 0.003 x 1000

We multiply by 0.003 by 1000. So, we have to move the decimal point three digits to the right.

a = 3

So, the the number is 3.

Practice Questions

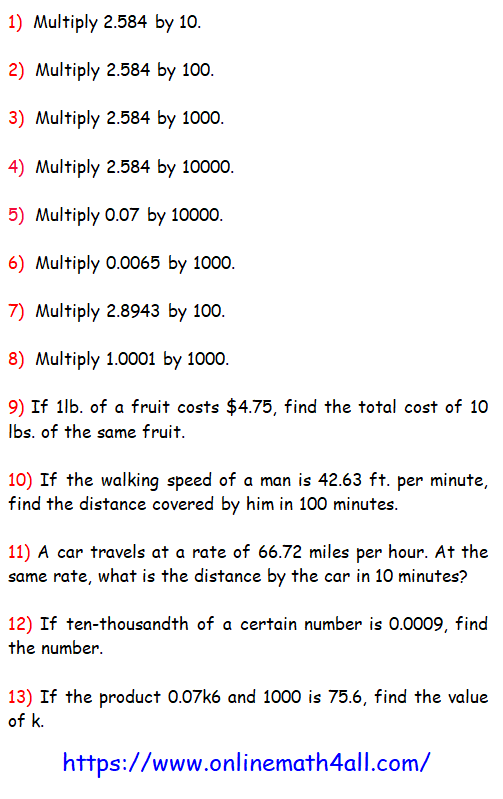
Click here to get detailed answers for the above questions.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.