NEGATIVE EXPONENTS
Consider the following exponential form.
a-b
Here the base is 'a' and the exponent is '-b'.
To evaluate a-b, first we have to change the negative sign of the exponent as positive shown below.
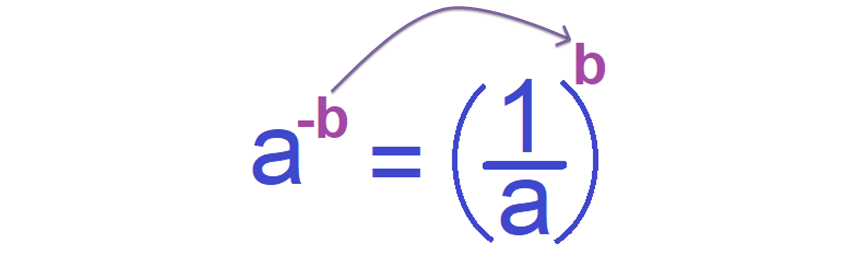
Consider the following exponential form where the base is a fraction.
(a/b)-m
Here, the negative exponent can be changed positive as shown below.
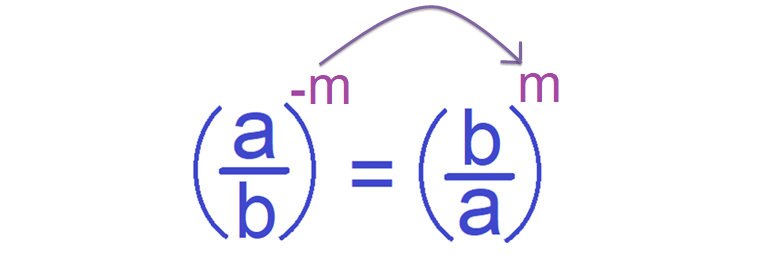
From the above two examples, it is clear that if there is a negative exponent for a base, the negative exponent can be changed as positive exponent by taking the reciprocal to the base.
Solved Problems
Problem 1 :
Evaluate :
8-2
Solution :
= 8-2
= (1/8)2
Distribute the exponent to numerator and denominator.
= 12 / 82
= 1/64
Problem 2 :
Evaluate :
(3/2)-3
Solution :
= (3/2)-3
= (2/3)3
Distribute the exponent to numerator and denominator.
= 23 / 33
= 8/27
Problem 3 :
Evaluate :
(-5/4)-2
Solution :
= (-5/4)-2
= (-4/5)2
Since the exponent is even, the negative sign inside the parentheses will become positive.
= (4/5)2
Distribute the exponent to numerator and denominator.
= 42 / 52
= 16/25
Problem 4 :
Evaluate :
(-7/6)-3
Solution :
= (-6/7)-3
= (-6/7)3
Since the exponent is odd, the negative sign inside the parentheses will remain same.
Distribute the exponent to numerator and denominator.
= - 63 / 73
= - 216/343
Problem 5 :
Evaluate :
(5-2) ⋅ (3-4)
Solution :
= (5-2) ⋅ (3-4)
= (1/5)2 ⋅ (1/3)4
= (1/25) ⋅ (1/81)
= 1/2025
Problem 6 :
Evaluate :
3-4/ 2-3
Solution :
= 3-4/ 2-3
= (1/3)4/ (1/2)3
= (1/81) / (1/8)
= (1/81) ⋅ (8/1)
= 8/81
Problem 7 :
It is given that a-1/3 = 4/3. Find the value of a.
Solution :
a-1/3 = 4/3
a = (4/3)-3/1
a = (4/3)-3
a = (3/4)3
Distribute the exponent to numerator and denominator.
a = 33 / 43
a = 27/64
Problem 8 :
It is given that (a/b)-x = (b/a)3. Find the value of x.
Solution :
(a/b)-x = (b/a)3
(b/a)x = (b/a)3
x = 3
Kindly mail your feedback to v4formath@gmail.com
We always appreciate your feedback.
©All rights reserved. onlinemath4all.com
Recent Articles
-
10 Hard SAT Math Questions (Part - 30)
Oct 17, 25 07:27 PM
10 Hard SAT Math Questions (Part - 30) -
10 Hard SAT Math Questions (Part - 29)
Oct 16, 25 06:04 AM
10 Hard SAT Math Questions (Part - 29) -
10 Hard SAT Math Questions (Part - 28)
Oct 14, 25 10:57 AM
10 Hard SAT Math Questions (Part - 28)
