OPERATIONS WITH NEGATIVE NUMBERS

Addition

In this section, we are going to see how to do addition when working with negative numbers. It can be done using a number line.

To add a positive number :

Move to the right on a number line

To add a negative number :

Move to the left on a number line

Example 1 :

Evaluate : -3 + 8.

Solution :

First number is -3 ----> start at -3.

A positive number 8 is added ----> move 8 units to the right of -3.

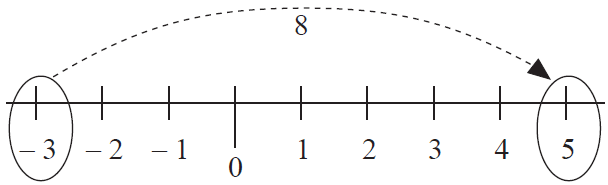
So, -3 + 8 = 5.

Example 2 :

Evaluate : 4 + (-2).

Solution :

First number is 4 ----> start at 4.

A negative number -2 is added ----> move 2 units to the left of 4.

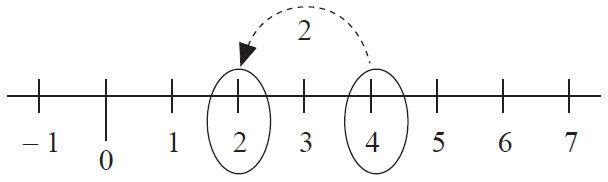
So, 4 + (-2) = 2.

Example 3 :

Evaluate : -8 + 5.

Solution :

First number is -8 ----> start at -8.

A positive number 5 is added ----> move 5 units to the right of -8.

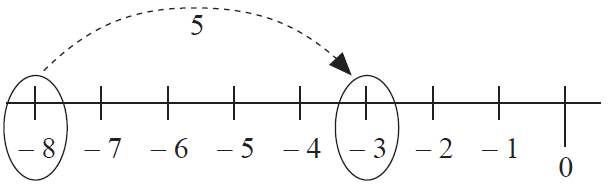
So, -8 + 5 = -3.

Example 4 :

Evaluate : -1 + (-3).

Solution :

First number is -1 ----> start at -1.

A negative number -3 is added ----> move 3 units to the left of -1.

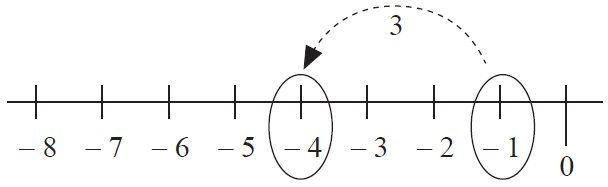
So, -1 + (-3) = -4.

Subtraction

In this section, we are going to see how to do subtraction when working with negative numbers. It can be done using a number line.

To subtract a positive number :

Move to the left on a number line

To subtract a negative number :

Move to the right on a number line

Example 5 :

Evaluate : 5 - 7.

Solution :

First number is 5 ----> start at 5.

A positive number 7 is subtracted ----> move 7 units to the left of 5.

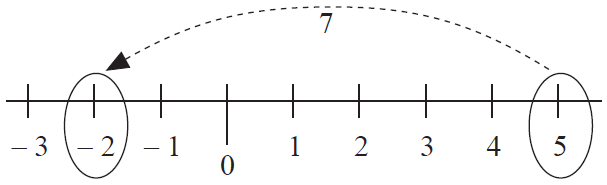
So, 5 - 7 = -2.

Example 6 :

Evaluate : 4 - (-2).

Solution :

First number is 4 ----> start at 4.

A negative number -2 is subtracted ----> move 2 units to the right of 4.

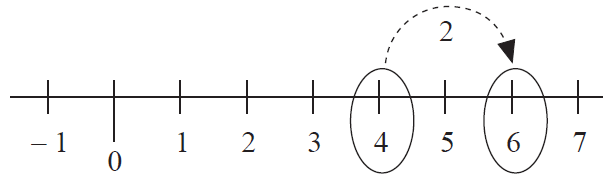
So, 4 - (-2) = 6.

Example 7 :

Evaluate : -2 - 3.

Solution :

First number is -2 ----> start at -2.

A positive number 3 is subtracted ----> move 3 units to the left of -2.

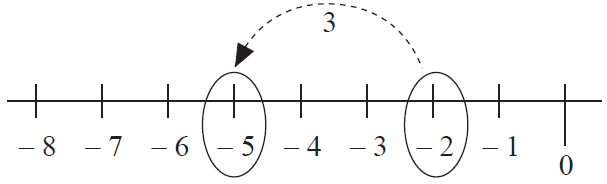
So, -2 - 3 = -5.

Example 8 :

Evaluate : -5 - (-3).

Solution :

First number is -5 ----> start at -5.

A negative number -3 is subtracted ----> move 3 units to the right of -5.

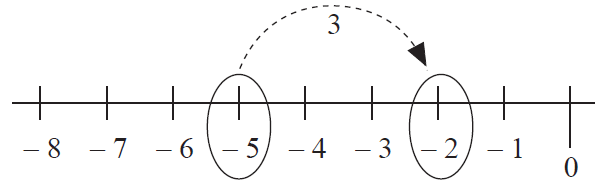
So, -5 - (-3) = -2.

Multiplication

In this section, we are going to see how to do multiplication when working with negative numbers. It can be done using a number line.

We can multiply the numbers as usual and decide the sign of the answer as shown below.

Positive x Negative = Negative

Negative x Positive = Negative

Negative x Negative = Positive

Example 9 :

Evaluate : 5 x (-7).

Solution :

5 times 7 is equal to 35.

Since a positive number is multiplied by a negative number, the answer is negative.

So, 5 x (-7) = -35.

Example 10 :

Evaluate : (-4) x 9.

Solution :

4 times 9 is equal to 36.

Since a negative number is multiplied by a positive number, the answer is negative.

So, (-4) x 9 = -36.

Example 11 :

Evaluate : (-3) x (-6).

Solution :

3 times 6 is equal to 18.

Since a negative number is multiplied by a negative number, the answer is positive.

So, (-3) x (-6) = 18.

Division

In this section, we are going to see how to do division when working with negative numbers. It can be done using a number line.

We can divide the numbers as usual and decide the sign of the answer as shown below.

Positive ÷ Negative = Negative

Negative ÷ Positive = Negative

Negative ÷ Negative = Positive

Example 12 :

Evaluate : 15 ÷ (-3).

Solution :

15 divided by 3 is equal to 5.

Since a positive number is divided by a negative number, the answer is negative.

So, 15 ÷ (-3) = -5.

Example 13 :

Evaluate : (-20) ÷ 5.

Solution :

20 divided by 5 is equal to 4.

Since a negative number is divided by a positive number, the answer is negative.

So, (-20) ÷ 5 = -4.

Example 14 :

Evaluate : (-28) ÷ (-4).

Solution :

28 divided by 4 is equal to 7.

Since a negative number is divided by a negative number, the answer is positive.

So, (-28) ÷ (-4) = 7.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.

OPERATIONS WITH NEGATIVE NUMBERS

Addition

Subtraction

Multiplication

Division

Recent Articles

Multi Step Algebra Word Problems

Solving Multi Step Word Problems Worksheet

Solving Multi Step Word Problems