POSITIVE AND NEGATIVE RATIONAL NUMBERS

Rational numbers may be classified as positive and negative rational numbers.

If both the numerator and the denominator of the fraction representing a rational number are of the same sign, then the rational number is positive.

For example, numbers like

If either the numerator or the denominator of the fraction representing a rational number is negative, then the rational number is negative.

For example, numbers like

All rational numbers can be represented as points on a number line.

Positive rational numbers are greater than 0. They are located to the right of 0 on a number line.

Negative rational numbers are less than 0. They are located to the left of 0 on a number line.

You can find the opposites of rational numbers that are not integers the same way you found the opposites of integers.

Two rational numbers are opposites if they are the same distance from 0 but on different sides of 0.

It has been clearly illustrated in the picture given below. 

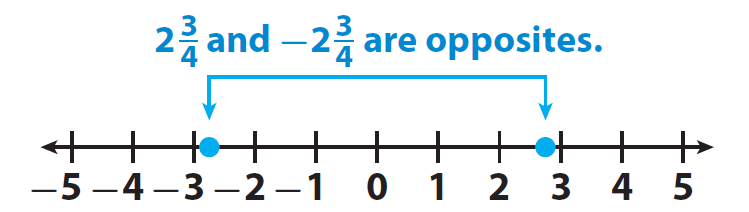
Zero is a rational number which is neither positive nor negative.

Note that

Example 1 :

Until May 15, 2007, the New York Stock Exchange priced the value of a share of stock in eighths, such as $27⅛ or at $41¾. The change in value of a share of stock from day to day was also represented in eighths as a positive or negative number.

The table shows the change in value of a stock over two days.

Graph the change in stock value for Wednesday and its opposite on a number line.

Solution :

Step 1 :

Graph the change in stock value for Wednesday on the number line.

The change in value for Wednesday is -4¼. Graph a point 4¼ units below 0.

Step 2 : 

Graph the opposite of -4¼.

The opposite of -4¼ is the same distance from 0 but on the other side of 0.

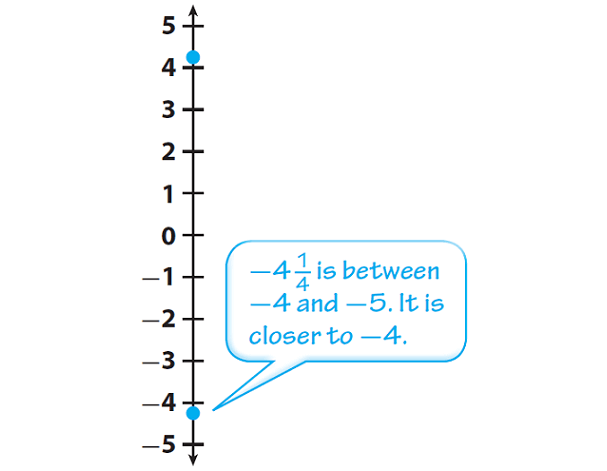
The opposite of -4¼ is 4¼.

The opposite of the change in stock value for Wednesday is 4¼.

Example 2 :

Five friends completed a triathlon that included a 3-mile run, a 12-mile bike ride, and a ½-mile swim. To compare their running times they created a table that shows the difference between each person’s time and the average time, with negative numbers representing times less than the average.

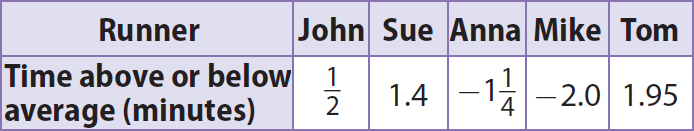
Use a number line to order the numbers from greatest to least.

Solution :

Step 1 : 

Write the fractions as equivalent decimals.

½ = 0.5 and -1¼ = -1.25

Step 2 : 

Use the number line to write the decimals in order.

From the above number line, we have the numbers in order from greatest to least are

1.95, 1.4, ½, -1¼, -2.0

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.