PRACTICE PROBLEMS IN VECTOR WITH SOLUTION

(1)  Verify whether the following ratios are direction cosines of some vector or not.

(i) 1/5 , 3/5 , 4/5

(ii) 1/√2, 1/2 , 1/2

(iii) 4/3, 0, 3/4            Solution

(2)  Find the direction cosines of a vector whose direction ratios are

(i) 1 , 2 , 3 (ii) 3 , - 1 , 3 (iii) 0 , 0 , 7         Solution

(3)  Find the direction cosines and direction ratios of the following vectors.

(i)  3i vector - 4j vector + 8k vector

(ii)  3i vector + j vector + k vector

(iii)  j vector

(iv)  5i vector - 3j vector - 48k vector

(v)  3i vector + 4j vector - 3k vector

(vi)  i vector - k vector       Solution

(4)  A triangle is formed by joining the points (1, 0, 0), (0, 1, 0) and (0, 0, 1). Find the direction cosines of the medians.     Solution

(5)  If 1/2, 1/√2, a are the direction cosines of some vector, then find a.     Solution

(6)  If (a , a + b , a + b + c) is one set of direction ratios of the line joining (1, 0, 0) and (0, 1, 0), then find a set of values of a, b, c.    Solution

(7)  Show that the vectors 2i − j + k, 3i − 4j − 4k, i − 3j − 5k form a right angled triangle.     Solution

(8)  Find the value of λ for which the vectors a = 3i + 2j + 9k and b = i + λj + 3k are parallel.    Solution

(9)  Show that the following vectors are coplanar

(i) i− 2 j + 3k, − 2i + 3j − 4k, − j + 2k

(ii) 5i + 6 j + 7k,  7i −8 j + 9k,  3i + 20j + 5k    Solution

(10)  Show that the points whose position vectors 4i + 5j + k, − j − k, 3i + 9j + 4k and −4i + 4j + 4k are coplanar.    Solution

(11)  If

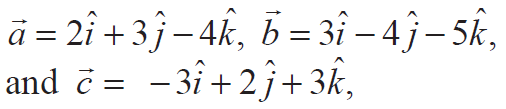
find the magnitude and direction cosines of

(i) a vector + b vector + c vector

(ii)  3a vector - 2b vector + 5c vector     Solution

(12)  The position vectors of the vertices of a triangle are i+2j +3k; 3i − 4j + 5k and − 2i+ 3j − 7k . Find the perimeter of the triangle (Given in vectors)    Solution

(13)  Find the unit vector parallel to 3a − 2b + 4c if a = 3i − j − 4k, b = −2i + 4j − 3k, and c = i + 2 j − k   Solution

(14)  The position vectors a vector, b vector, c vector of three points satisfy the relation 2a vector - 7b vector + 5c vector. Are these points collinear?     Solution

(15)  The position vectors of the points P, Q, R, S are i + j + k, 2i+ 5j, 3i + 2j − 3k, and i − 6j − k respectively. Prove that the line PQ and RS are parallel    Solution

(16)  Find the value or values of m for which m (i + j + k) is a unit vector.     Solution

(17)  Show that the points A (1, 1, 1), B(1, 2, 3) and C(2, - 1, 1) are vertices of an isosceles triangle    Solution

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.