PRACTICE PROBLEMS ON COMPLEMENT OF A SET

(1)  If U  =  {x : 0 ≤ x ≤ 10, x ∈ W} and A = {x : x is a multiple of 3}. Find A'.

(2)  If U is the set of all natural numbers and A' is the set of all composite numbers, then what is A?

(3)  If  U  =   {a, b, c, d, e, f, g, h},  A  =  {a, b, c, d} and B = { b, d, f, g}, find

(i) A∪B     (ii) (A∪B)'      (iii)  A∩B     (iv) (A∩B)'

(4)  If    U  =    { x :  1 ≤ x ≤ 10, x∈ℕ},  A = {1, 3, 5, 7, 9} and B = {2, 3, 5, 9, 10}, find

(i) A'    (ii) B'    (iii) A'∪B'     (iv) A'∩B'  

(5)  If U  =  {0, 1, 2, 3, 4, 5, 6, 7} and E = {2, 3, 5, 7}, list the set E' and illustrate E and E' on a Venn diagram.  

Hence find :

(a)  n(E)     (b) n(E')     (c) n(U)

(6)  Consider U  =  {x | x ≤ 12, x∊ Z⁺}

A  =  {2, 7, 9, 10, 11} and B  =  {1, 2, 9, 11, 12}.

a) Show these sets on a Venn diagram.

b) List the elements of :

(i) A n B (ii) A U B  (iii) B' (iv) A'

(7)  Simplify:

(a)  AUA' for any set A ∈ U.

(b) A n A' for any set A ∈ U.

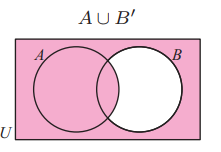
(8)  If A and B are two non-disjoint sets, shade the region of a Venn diagram representing:

(a) A'  (b) A' n B  (c) A U B' (d) A' n B'

(9)  Write down the members of :

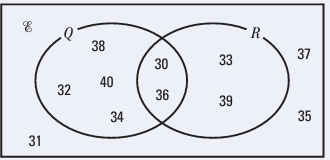
a) the universal set     b) set Q       c) set R'

d)  Q n R       e)  Q U R

(1) Solution :

First let us write the given sets.

U  =  {0, 1, 2, 3, 4, 5, 6, 7, 8, 9, 10}

A  =  {3, 6, 9}

So, A' is the set of all elements in U which are not in A.

A'  =   {0, 1, 2, 4, 5, 7, 8, 10}

(2)  Solution :

Let U  =  {1, 2, 3, 4, 5, .................}

Set U is containing the set of natural numbers, including prime and composite numbers.

A' is containing composite numbers, then the set A will contain set of prime numbers.

(3)  Solution :

U  =  {a, b, c, d, e, f, g, h},  A =  {a, b, c, d} and B = { b, d, f, g}

(i) A∪B  =  {a, b, c, d} ∪ { b, d, f, g}

 A∪B  =  {a, b, c, d, f, g}

(ii)  Write the elements from U (universal set) by excluding the elements from AUB.

(A∪B)'  =  {e, h}

(iii)  Write the common elements of the sets A and B.

A∩B  =  {b, d}

(iv)  Write the elements from U (universal set) by excluding the elements from AnB.

 (A∩B)'  =  {a, c, e, f, g, h}

(4)  Solution :

U = { x : 1 ≤ x ≤ 10, x∈ℕ},  A = {1, 3, 5, 7, 9} and B = {2, 3, 5, 9, 10}

First we will write the given sets A and B.

U  =  {1, 2, 3, 4, 5, 6, 7, 8, 9, 10}

A  =  {1, 3, 5, 7, 9}

B  =  {2, 3, 5, 9, 10}

(i) A'  =   {2, 4, 6, 8, 10}

(ii) B'  =  {1, 4, 6, 7, 8}

(iii) A'∪B'  =  {2, 4, 6, 8, 10} ∪ {1, 4, 6, 7, 8}

A'∪B'  =  {1, 2, 4, 6, 7, 8, 10}

(iv) A'∩B'  =  {2, 4, 6, 8, 10} ∩  {1, 4, 6, 7, 8}

A'∩B'  =  {4, 6, 8}

(5)  Solution :

U  =  {0, 1, 2, 3, 4, 5, 6, 7} and E  =  {2, 3, 5, 7}

E'  =  {0, 1, 4, 6}

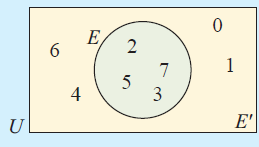
(a)  n(E)  =  4 (Number of elements in the set E)

(b)  n(E')  =  4  (number of elements other than E)

(c)  n(U)  =  8  (Number of elements in all sets)

(6)  Solution :

U  =  {x | x ≤ 12, x∊ Z⁺}

Z is a set of integers.

U  =  {1, 2, 3, 4, 5, 6, 7, 8, 9, 10, 11, 12}, A = {2, 7, 9, 10, 11} and B  =  {1, 2, 9, 11, 12}.

(a) 

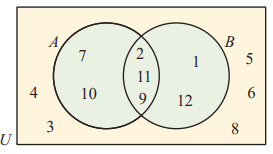
(i) A n B  =  {2, 9, 11}

(ii) A U B  =  {1, 2, 7, 9, 10, 12}

(iii) B'  =  {3, 4, 5, 6, 7, 8, 10}

(iv) A'  =  {1, 3, 4, 5, 6, 8, 12}

(7)  Solution :

(a)  AUA' for any set A ∈ U.

Let U  =  {1, 2, 3}  A  =  {1}

So, A'  =  {2, 3}

AUA'  =  {1, 2, 3}  =  U

(b) A n A' for any set A ∈ U.

A n A'  =   {1} n {2, 3}

A n A'  =  Null set

(8)  Solution :

(a) A'  

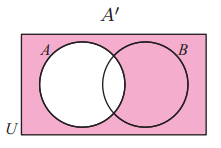
(b) A'nB 

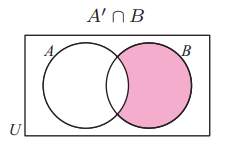
(c) AUB' 

(d) A'nB'

(9)  Solution :

a) the universal set

{31, 32, 33, 34, 35, 36, 37, 38, 39, 40}

b) set Q = {30, 32, 34, 36, 38, 40} 

c) set R'= { 31, 32, 34, 35, 37, 38, 40}

d)  Q n R = {30, 36}

e)  Q U R = {30, 32, 33, 34, 36, 39, 38, 40}

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.