PRACTICE PROBLEMS ON MEAN MEDIAN MODE

Problem 1 :

Find the (i) mean  (ii) median  (iii) mode for each of the following data sets :

a)  12, 17, 20, 24, 25, 30, 40

b)  8, 8, 8, 10, 11, 11, 12, 12, 16, 20, 20, 24

c)  7.9, 8.5, 9.1, 9.2, 9.9, 10.0, 11.1, 11.2, 11.2, 12.6, 12.9

d)  427, 423, 415, 405, 445, 433, 442, 415, 435, 448, 429, 427, 403, 430, 446, 440, 425, 424, 419, 428, 441

Solution

Problem 2 :

Consider the following two data sets :

Data set A : 5, 6, 6, 7, 7, 7, 8, 8, 9, 10, 12

Data set B : 5, 6, 6, 7, 7, 7, 8, 8, 9, 10, 20

a)  Find the mean for both Data set A and Data set B.

b)  Find the median of both Data set A and Data set B.

c)  Explain why the mean of Data set A is less than the mean of Data set B.

d)  Explain why the median of Data set A is the same as the median of Data set B

Solution

Problem 3 :

The table given shows the result when 3 coins were tossed simultaneously 40 times. The number of heads appearing was recorded.

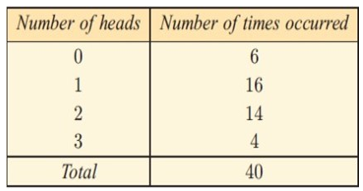
Calculate the :   a)  mean     b)  median     c)  mode

Solution

Problem 4 :

The following frequency table records the number of text messages sent in a day by 50 fifteen-years-olds

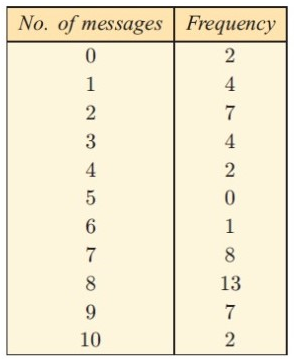
a)  For this data, find the : (i) mean   (ii)  median   (iii)  mode

b)  construct a column graph for the data and show the position of the measures of centre (mean, median and mode) on the horizontal axis.

c)  Describe the distribution of the data.

d)  why is the mean smaller than the median for this data ?

e)  which measure of centre would be the most suitable for this data set ?

Solution

Problem 5 :

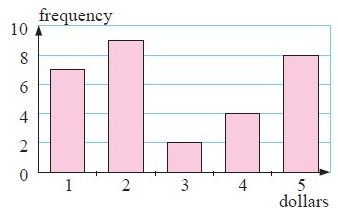
The frequency column graph alongside gives the value of donations for an overseas aid organisation, collected in a particular street.

a)  construct the frequency table from the graph.

b)  Determine the total number of donations.

c)  For the donations find the :  (i)  mean   (ii)  median   (iii)  mode

d) which of the measures of central tendency can be found easily from the graph only ?

Solution

Problem 6 :

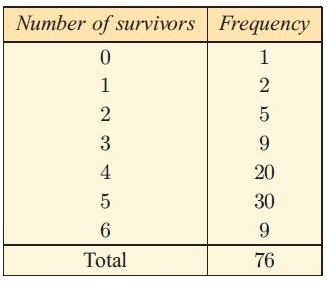
Hui breeds ducks. The number of ducklings surviving for each pair after one month is recorded in the table.

a)  Calculate the : (i)  mean   (ii)  median   (iii) mode

b)  Is the data skewed ?

c)  How does the skewness of the data affect the measures of the middle of the distribution ?

Solution

Problem 7 :

Find the mean of the data in the pictograph below.

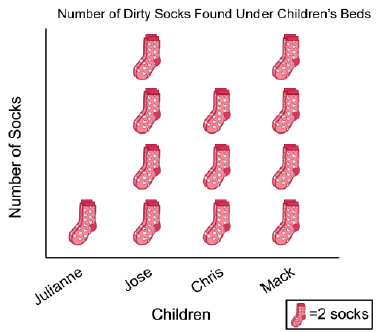
A. The mean is 13.        B. The mean is 6 

C. The mean is 2           D. The mean is 26.

Solution

Problem 8 :

The following table shows the distances of different places from Rachel’s house. Calculate the mean distance.

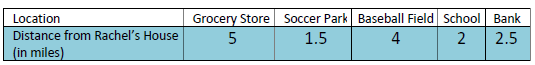
A. The mean is 2.5 miles.    B. The mean is 4 miles.

C. The mean is 15 miles.      D. The mean is 3 miles.

Solution

Problem 9 :

Find the mean, median and mode for the following frequency table:

Solution

Problem 10 :

Find the mean, median and mode for the following frequency table:

Solution

Problem 11 :

For this column graph on shoe sizes, determine the 

(i) Mode

(ii) Median shoe size

Solution

Problem 12 :

The mean of 12 scores is 8.8. What is the sum of the scores?

Solution

Problem 13 :

While on camping holiday, Daffyd drove on average 325 km per day for a period of 7 days. How far Daffyd  drive in total while on holiday ?

Solution

Problem 14 :

Find a, given that 10, a, 15, 20, a, a, 17, 7 and 15 have a mean 12.

Solution

Answers 

(2)

(c)  the mean of A is less than the mean of B.

(d)  median is the same.

(3)  (a)  Mean  =  1.4    (b)  median  =  1  (c)   mode  =  1

(4)  

(a)  (i)  Mean  =  5.74  (ii)   median  =  7  (iii)  mode  =  8

(b) 

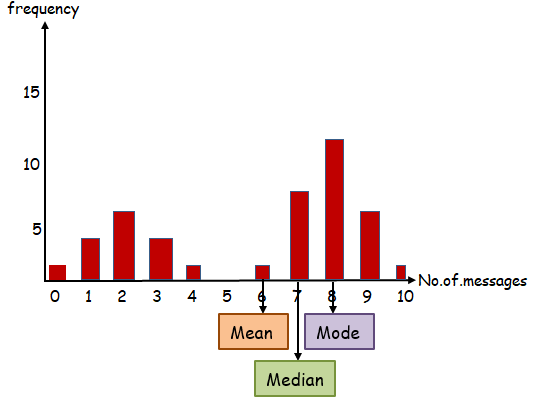
(c)   bimodal data.

(d)

The mean takes into account the full range of numbers of text messages and is affected by extreme values. Also, the value which is lower than the median is well below it.

(e)  The median

(5)  

(a)

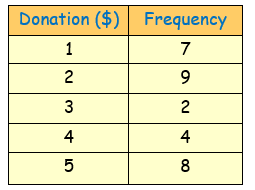
(b)  ∑f  =  30

(c)  (i)  Mean  =  $2.9  (ii)  median  =  $2  (iii)  mode  =  $2

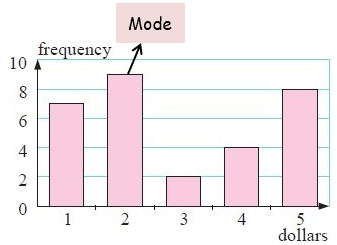
(6)  

(a)  (i)  Mean  =  4.25   (ii)  median  =  5   (iii)  mode  =  5

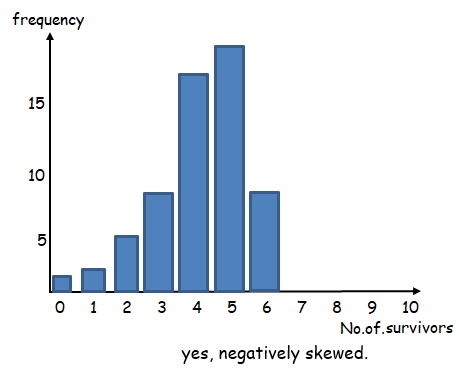
c)  By observing the graph, the mean is less than the median and mode.

7) mean = 2

8) mean = 3

9)  

Mean  =  28

Mode  =  25 and

Mode  =  30.

10)

Mean  =  25

Mode  =  25

Mode  =  23

11)

i) Mode = 8

ii)  Median = 8.5

12) 105.6

13)  2275

14)  a = 8

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.