PROBLEMS ON LAGRANGES MEAN VALUE THEOREM

Let f (x) be continuous in a closed interval [a, b] and differentiable in the open interval (a, b) (where f (a), f (b) are not necessarily equal). Then there exist at least one point c ∈ (a, b) such that,

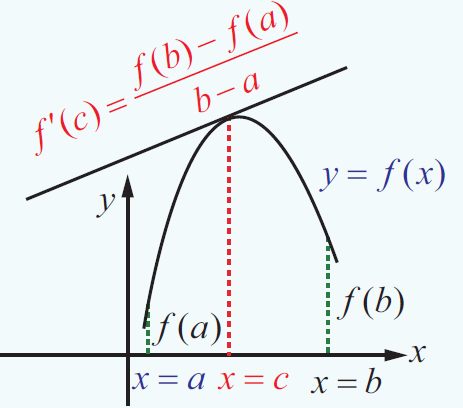
A geometrical meaning of the Lagrange’s mean value theorem is that the instantaneous rate of change at some interior point is equal to the average rate of change over the entire interval.

Problem 1 :

A race car driver is racing at 20th km. If his speed never exceeds 150 km/hr, what is the maximum distance he can cover in the next two hours.

Solution :

Here he is travelling in the interval [0, 2].

f'(x)  =  150 km/hr

f(a)  =  20, f(b)  =  ?

f'(x)  =  [f(b) - f(a)]/(b-a)

150  =  [f(b) - 20]/(2-0)

150(2)  =  f(b) - 20

300+20  =  f(b)

f(b)  =  320

So, he can cover the maximum distance of 320 km/hr.

Problem 2 :

Suppose that for a function f (x), 

f'(x) ≤ 1 for all 1 ≤ x ≤ 4. Show that f(4) − f(1) ≤ 3 .

Solution :

Given :

f'(x) ≤ 1

f'(x)  =  [f(b) - f(a)]/(b-a)

[f(4)-f(1)]/(4-1) ≤ 1

[f(4)-f(1)]/3 ≤ 1

[f(4)-f(1)] ≤ 3

Problem 3 :

Does there exist a differentiable function f(x) such that f(0) = −1, f(2) = 4 and f'(x) ≤ 2 for all x . Justify your answer.

Solution :

f'(x)  =  [f(b) - f(a)]/(b-a)

Given :

f'(x)  ≤ 2

=  [4-(-1)] / (2-0)

=  5/2

=  2.5

f'(x)  =  2.5, it is not possible, because it is not in the interval [0, 2].

Problem 4 :

Show that there lies a point on the curve

f(x)  =  x(x+3)e^π/2, -3 ≤  x ≤ 0,

where the tangent is parallel to the x-axis.

Solution :

Since the tangent is parallel to x-axis, its slope must be 0.

f'(x)  =  e^π/2[x(1) + (x+3)(1)]

f'(x)  =  e^π/2[2x+3]

e^π/2[2x+3]  =  0

2x+3  =  0

2x  =  -3

x  =  -3/2,  ∊ [-3, 0]

Problem 5 :

Using mean value theorem prove that for a > 0, b > 0,

|e^-a-e^-b| < |a-b|

Solution :

Let f(x)  =  e^-x

f'(x)  =  e^-x

f(a)  =  e^-a

f(b)  =  e^-b

f'(x)  =  [f(b) - f(a)] / (b-a)

|[e^-b - e^-a] / (b-a) | ≤ |e^-c| ≤ 1

|[e^-b - e^-a]| ≤ |b-a|

Problem 6 :

Skater Sully is riding a skateboard back and forth on a street that runs north/south. The twice differentiable function S model's Sully's position on a street, measured by how many meters north he is from his starting point at time t, measured in seconds from the start of his ride. The table below gives values of S(t) at selected times t.

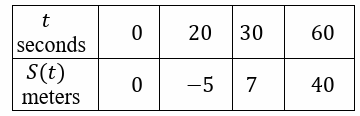
a) For 0 ≤ t ≤ 20must be there a time when Sully is 2 meters south of his starting point ? Justify your answer.

a) For 30 ≤ t ≤ 60 must be there a time t when Sully velocity 1.1 meters per pound  is 2 meters south of his starting point ? Justify your answer.

Solution :

a)  From the table S(0) = 0 and s(20) = -5

According to the IVT, there is a value 𝒄 such that 𝑺(𝒄) = -𝟐 and 𝟎 ≤ 𝒄 ≤ 𝟐0.

b) s(t) is differentiable and from the table,

s(30) = 7 and s(60) = 40

= s(60) - s(30) / (60 - 30)

= (40 - 7)/30

= 33/30

= 1.1

Yes accordingly to the mean value theorem, there must be a value of c where 30 ≤ 𝒄 ≤ 60 and s'(c) = 1.1

Problem 7 :

A particle is moving along the 𝑥-axis. The twice-differentiable function 𝑠 models the particles distance from the origin, measured in centimeters, at time 𝑡, measured in seconds.

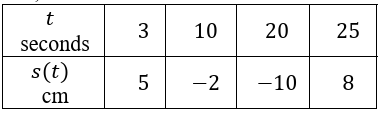
a. For 20 ≤ 𝑡 ≤ 25, must there be a time 𝑡 when the particle is at the origin? Justify your answer.

b. For 3 ≤ 𝑡 ≤ 10, must there be a time 𝑡 when the particle’s velocity is 1 cm per second? Justify your answer.

Solution :

a) s(t) is continuous and s(20) = -10 and s(25) = 8

According to the mean value theorem is a value c such that s(c) = 0 and 20  ≤ 𝑡 ≤ 25

b) s(t) is differentiable and from the table

s(10) = -2 and s(3) = 5

= s(10) - s(3) / (10 - 3)

= (-2 - 5) / 7

= -7/7

= -1

Yes, accordingly mean value theorem, there must be a value c where  3 ≤ 𝑡 ≤ 10 and s('c) = -1

Problem 8 :

A hot air balloon is launched into the air with a human pilot. The twice-differentiable function ℎ models the balloon’s height, measured in feet, at time 𝑡, measured in minutes. The table below gives values of ℎ𝑡 at selected times 𝑡.

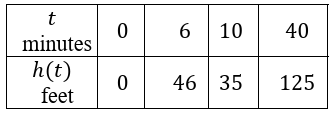
a)  For 6 ≤ 𝑡 ≤ 10, must there be a time 𝑡 when the balloon is 50 feet in the air? Justify your answer.

b)   For 10 𝑡 40, must there be a time 𝑡 when the balloon’s velocity is 3 feet per second? Justify your answer.

Solution :

a)  𝒉(𝟔) = 𝟒𝟔 and 𝒉(𝟏𝟎) = 𝟑𝟓. No, the IVT does not guarantee a height of 50

b) 𝒉(𝒕) is differentiable and from the table,

s(10) = 35 and s(40) = 125

= s(40) - s(10) / (40 - 10)

= (125 - 35) / 30

= 90 / 30

= 3

Yes. According to the MVT, there must be a value 𝒄 where 𝟏𝟎  ≤ 𝒄  ≤ 𝟒𝟎 and 𝒉'(𝒄) = 𝟑

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.