SETS AND SUBSETS OF RATIONAL NUMBERS

By understanding which sets are subsets of types of numbers, we can verify whether statements about the relationships between sets are true or false.

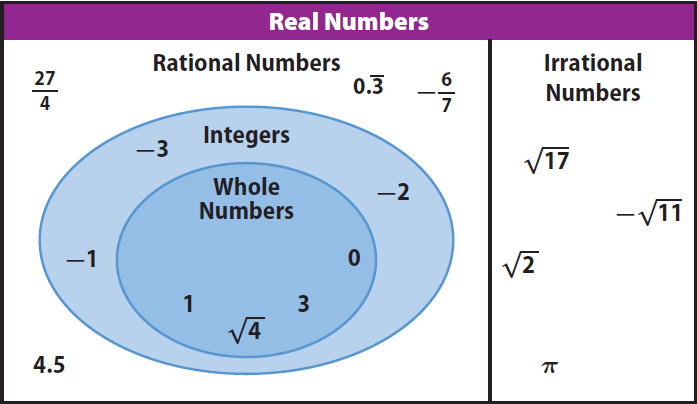
The picture given below clearly illustrates the subsets of real numbers.

Solved Questions

Question 1 : 

Tell whether the given statement is true or false. Explain your choice.

"All integers are rational numbers"

Answer :

True

Every integer is included in the set of rational numbers. Integers are a subset of the set of rational numbers.

Question 2 : 

Tell whether the given statement is true or false. Explain your choice.

"No rational numbers are whole numbers"

Answer :

False

A whole number can be written as a fraction with a denominator of 1, so every whole number is included in the set of rational numbers. The whole numbers are a subset of the rational numbers.

Question 3 : 

Tell whether the given statement is true or false. Explain your choice.

"All rational numbers are integers"

Answer :

False

Every integer is a rational number, but not every rational number is an integer. 

For example, rational numbers such as 3/5 and -5/2 are not integers. 

Question 4 : 

Tell whether the given statement is true or false. Explain your choice.

"All integers are whole numbers"

Answer :

False

Every whole number is an integer, but it is not true that every integer is a whole number.

Integers such as -1 and -6 are not whole numbers.

Question 5 : 

Tell whether the given statement is true or false. Explain your choice.

"All whole numbers are rational numbers"

Answer :

True

Whole numbers are a subset of the set of rational numbers and can be written as a ratio of the whole number to 1.

Question 6 : 

Tell whether the given statement is true or false. Explain your choice.

"Some integers are irrational numbers"

Answer :

False

All integers are rational numbers.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.