SOLVING SYSTEMS OF INEQUALITIES WITH ONE VARIABLE

Example 1 :

Solve the following system of linear inequalities 

3x - 6 ≥ 0,  4x - 10 ≤ 6

Solution :

Solving the equations separately

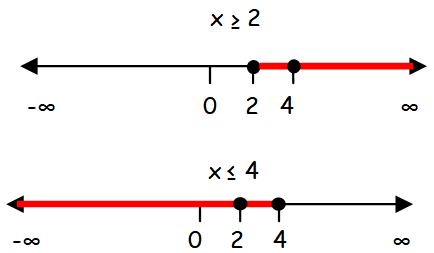
The solution set of first given inequality is [2,  ∞).

The solution set of second given inequality is (-∞, 4]

The intersection of these solution sets is the set [2, 4].

Example 2 :

Solve the following system of linear inequalities 

(5x/4) + (3x/8)  >  39/8

(2x - 1)/12 - (x - 1)/3 < (3x + 1)/4

Solution :

Solving the first given inequality 

(5x/4) + (3x/8)  >  39/8

(10x + 3x)/8 > 39/8

(13x/8)  > 39/8

Multiplying by 8 through out the equations

13x > 39

Divide by 13, we get

x > 39/13

x > 3

Solution set of the first given inequality is (3, ∞)

Solving the second given inequality :

(2x - 1)/12 - (x - 1)/3 < (3x + 1)/4

[(2x - 1) - 4(x - 1)]/12 < (3x + 1)/4

[(2x - 1 - 4x + 4)]/12 < (3x + 1)/4

(-2x + 3)/12 < (3x + 1)/4

Multiply 12 on both sides

(-2x + 3) < 3(3x + 1)

-2x + 3 < 9x + 3

Subtract 9x on both sides

-2x - 9x + 3 < 3

Subtract 3 on both sides

-11x  < 0

Divide by -1 on both sides

x > 0

The solution set of the second inequality is (0, ∞)

The intersection of the two solutions sets is (3, ∞).

So, the solution of given inequalities is (3, ∞).

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.