SOLVING SYSTEMS OF LINEAR AND QUADRATIC EQUATIONS

Example 1 :

Solve :

y = x² + 3x - 5

y = x + 3

Solution :

y = x² + 3x - 5 ----(1)

y = x + 3 ----(2)

By equating (1) and (2), we get 

x + 3 = x² + 3x - 5

x² + 3x - x - 3 - 5 = 0

x² + 2x - 8 = 0

(x + 4)(x - 2) = 0

x + 4 = 0  or  x - 2 = 0

x = -4  or  x = 2

To get corresponding y values, we can apply each x values one by one either in first or second equation. 

If x = -4, then y = -4 + 3 = -1.

If x = 2, then y = 2 + 3 = 5.

So, the given parabola and straight line will intersect at the points (-4, -1) and (2, 5). 

By observing the graph given below, we can understand what is point of intersection.

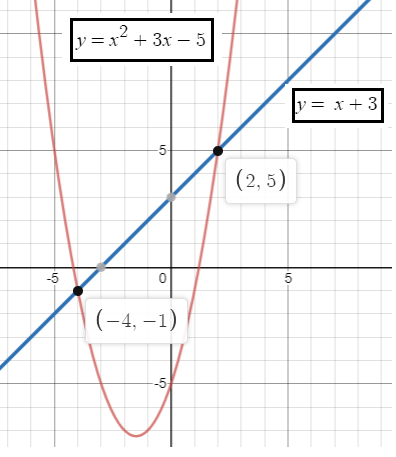
Example 2 :

Solve :

y = x² - 4x + 6

y = x + 2

Solution :

y = x² - 4x + 6 ----(1)

y = x + 2 ----(2)

By equating (1) and (2), we get 

x + 2 = x² - 4x + 6

x² - 4x - x + 6 - 2 = 0

x² - 5x + 4 = 0

(x - 1)(x - 4) = 0

x - 1 = 0   or  x - 4 = 0

x = 1  or  x = 4

To get corresponding y values, we can apply each x values one by one either in first or second equation. 

If x = 1, then y =1 + 2 = 3.

If x = 4, then y = 4 + 2 = 6.

So, the given parabola and straight line will intersect at the points (1, 3) and (4, 6).

Example 3 :

Solve :

y = x² - 10x + 14

y = 7x - 16

Solution :

y = x² - 10x + 14 ----(1)

y = 7x - 16 -----(2)

By equating (1) and (2), we get

x² - 10x + 14 = 7x - 16 

x² - 10x - 7x + 14 + 16 = 0

x² - 17x + 30 = 0

(x - 12)(x - 5) = 0

x - 12 = 0 and x - 5 = 0

x = 12 and x = 5

To get corresponding y values, we can apply each x values one by one either in first or second equation.

So, the given parabola and straight line will intersect at the points (12, 68) and (5, 19). 

Example 4 :

Solve :

y = x² - 24

y = x - 12

Solution :

y = x² - 24 ----(1)

y = x - 12 ----(2)

By equating (1) and (2), we get 

x - 12 = x² - 24

x² - x - 24 + 12 = 0

x² - x - 12 = 0

(x - 4)(x + 3) = 0

x - 4 = 0  or  x + 3 = 0

x = 4  or  x = -3

To get corresponding y values, we can apply each x values one by one either in first or second equation.

So, the given parabola and straight line will intersect at the points (4, -8) and (-3, -15). 

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.