SOLVING TWO-STEP AND MULTI-STEP INEQUALITIES
Inequalities that contain more than one operation require more than one step to solve. Use inverse operations to undo the operations in the inequality one at a time.
Solving Two-Step Inequalities
Solve each inequality and graph the solutions :
Example 1 :
170 + 4x ≤ 510
Solution :
170 + 4x ≤ 510
Because 170 is added to 4x, subtract 160 from each side to undo the addition.
(170 + 4x) - 170 ≤ 510 - 170
170 + 4x - 170 ≤ 340
4x ≤ 340
Because x is multiplied by 4, divide each side by 4 to undo the multiplication.
4x/4 ≤ 340/4
x ≤ 85
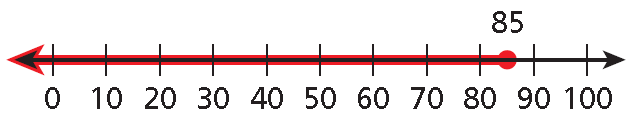
Example 2 :
8 - 2y ≤ 22
Solution :
8 - 2y ≤ 22
Because 8 is added to -2y, subtract 8 from each side to undo the addition.
(8 - 2y) - 8 ≤ 22 - 8
8 - 2y - 8 ≤ 14
-2y ≤ 14
Because y is multiplied by -2, divide each side by -2 to undo the multiplication and change ≤ to ≥.
-2y/(-2) ≥ 14/(-2)
y ≥ -7
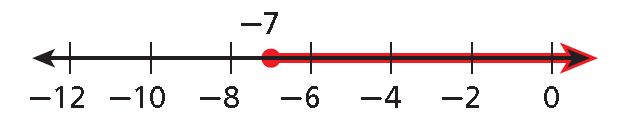
Solving Multi-Step Inequalities
Solve each inequality and graph the solutions :
Example 3 :
(7x + 2)/5 < 8.8
Solution :
(7x + 2)/5 < 8.8
Because (7x + 2) is divided by 5, multiply each side by 5 to undo the division.
5[(7x + 2)/5] < 5(8.8)
7x + 2 < 44
Because 2 is added to 7x, subtract 2 from each side to undo the addition.
(7x + 2) - 2 < 44 - 2
7x + 2 - 2 < 44 - 2
7x < 42
Because x is multiplied by 7, divide each side by 7 to undo the multiplication.
7x/7 < 42/7
x < 6
x < 6

Example 4 :
(1 -3y)/2 ≤ -2.5
Solution :
(1 -3y)/2 ≤ -2.5
Because (1 - 3y) is divided by 2, multiply each side by 2 to undo the division.
2[(1 - 3y)/2] ≤ 2(-2.5)
1 - 3y ≤ -5
Because 1 is added to -3y, subtract 1 from each side to undo the addition.
(1 -3y) - 1 ≤ -5 - 1
1 - 3y - 1 ≤ -6
-3y ≤ -6
Because y is multiplied by -3, divide each side by -3 and change ≤ to ≥.
-3y/(-3) ≥ -6/(-3)
y ≥ 2
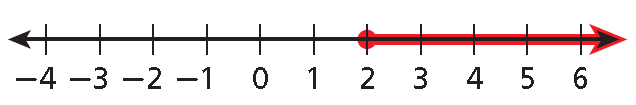
To solve more complicated inequalities, you may first need to simplify the expressions on one or both sides by using the order of operations, combining like terms, or using the Distributive Property.
Simplifying Before Solving Inequalities
Solve each inequality and graph the solutions :
Example 5 :
-5 + (-7) < -5x - 2
Solution :
-5 + (-7) < -5x - 2
Combine like terms.
-12 < -5x - 2
Because 2 is subtracted from -5x, add 2 to each side to undo the subtraction.
-12 + 2 < (-5x - 2) + 2
-10 < -5x - 2 + 2
-10 < -5x
Because x is multiplied by -5, divide each side by -5 to undo the multiplication and change < to >.
-10/(-5) > -5x/(-5)
2 > x
x < 2
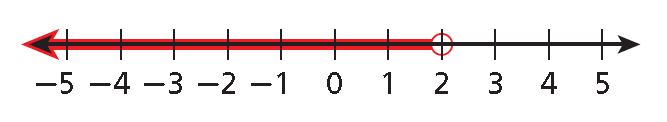
Example 6 :
-3(3 - y) < 16
Solution :
-3(3 - y) < 16
Distribute -3 on the left side.
-9 + 3y < 16
Because -9 is added to 3y, add 9 to each side to undo the addition.
9 + (-9 + 3y) < 9 + 16
9 - 9 + 3y < 25
3y < 25
Because y is multiplied by 3, divide each side by 3 to undo the multiplication.
3y/3 < 25/3
y < 8⅓
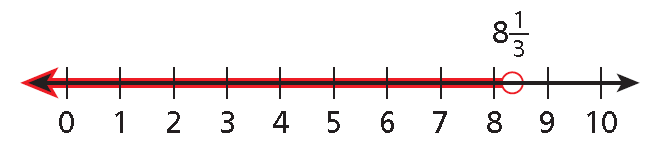
Example 7 :
4m/5 + 1/2 < 3/5
Solution :
4m/5 + 1/2 < 3/5
In the fractions above, we find the denominators 2 and 5.
Least common multiple of the denominators (2, 5) = 10.
Multiply each side by the least common multiple, 10 to get rid of the fractions.
10(4m/5 + 1/2) < 10(3/5)
10(4m/5) + 10(1/2) < 30/5
40m/5 + 10/2 < 6
8m + 5 < 6
Because 5 is added to 8m, subtract 5 to each side to undo the addition.
(8m + 5) - 5 < 6 - 5
8m + 5 - 5 < 1
8m < 1
Because x is multiplied by 8, divide each side by 8 to undo the multiplication.
8m/8 < 1/8
m < 1/8
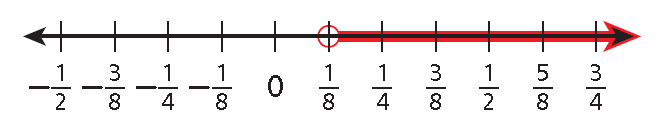
Gardening Application
Example 8 :
To win the blue ribbon for the Heaviest Pumpkin Crop at the county fair, the average weight of David's two pumpkins must be greater than 829 lb. One of his pumpkins weighs 897 lb. What is the least number of pounds the second pumpkin could weigh in order for David to win the blue ribbon?
Solution :
Let y represent the weight of the second pumpkin. The average weight of the pumpkins is the sum of each weight divided by 2.
(897 plus y) divided by 2 must be greater than 829
(897 + y)/2 > 829
Because (897 + y) is divided by 2, multiply each side by 2 to undo the division.
2[(897 + y)/2] > 2(829)
897 + y > 1658
Because 897 is added to y, subtract 897 from each side to undo the addition.
(897 + y) - 897 > 1658 - 897
897 + y - 897 > 761
y > 761
The second pumpkin must weigh more than 761 pounds.
Check :
|
Check the endpoint, 761 (897 + y)/2 = 829 (897 + 761)/2 = 829 1658/2 = 829 829 = 829 ✓ |
Check a number > 761 (897 + y)/2 > 829 (897 + 765)/2 > 829 1662/2 > 829 831 > 829 ✓ |
Kindly mail your feedback to v4formath@gmail.com
We always appreciate your feedback.
©All rights reserved. onlinemath4all.com
Recent Articles
-
AP Calculus AB Problems with Solutions (Part - 10)
Feb 06, 25 09:46 AM
AP Calculus AB Problems with Solutions (Part - 10) -
AP Calculus AB Problems with Solutions (Part - 8)
Feb 05, 25 12:22 PM
AP Calculus AB Problems with Solutions (Part - 8) -
AP Calculus AB Problems with Solutions (Part - 9)
Feb 05, 25 12:15 PM
AP Calculus AB Problems with Solutions (Part - 9)
