SYMMETRY PROPERTY OF CONGRUENCE

When two shapes or figures have the same shape and size, we use the term congruence and they shapes or figures are said to be congruent (≅).

In geometry, the symmetry property of congruence states that if shape 1 is congruent to shape 2, then shape 2 is also congruent to shape 2.

Similarly, if line segment AB is congruent to line segment CD, then CD is also congruent to AB.

An also, if ∠A is congruent to ∠B, then ∠B is also congruent to ∠A.

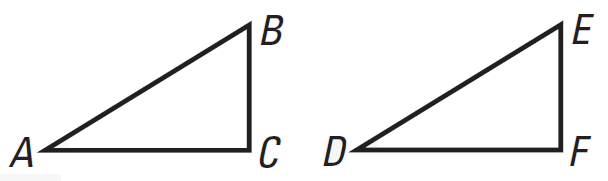
In the diagram above,

if ΔABC ≅ ΔDEF, then

ΔDEF ≅ ΔABC

Example :

In the diagram given below, triangle ABD is congruent to triangle BCD. Is triangle BCD congruent to triangle ABC ? Explain your reasoning.

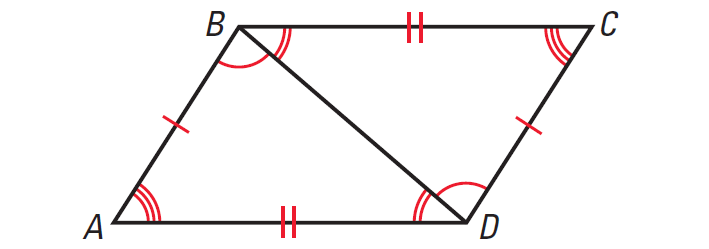
Solution :

Yes, triangle BCD is congruent to triangle ABC.

By Symmetry Property of Congruent Triangles,

if ΔABD ≅ ΔBCD, then

ΔBCD ≅ ΔABD.

Thus, triangle BCD is congruent to triangle ABC

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.

Recent Articles