TRIGONOMETRIC IDENTITIES EXAMPLES WITH SOLUTIONS

Abbreviations used :

L.H.S -----> Left hand side

R.H.S -----> Right hand side

Example 1 :

Prove :

tanθ/(1 - cotθ) + cotθ/(1 - tanθ)  =  1 + secθ cosecθ

Solution :

L.H.S : 

=  tanθ/(1 - cotθ) + cotθ/(1 - tanθ)

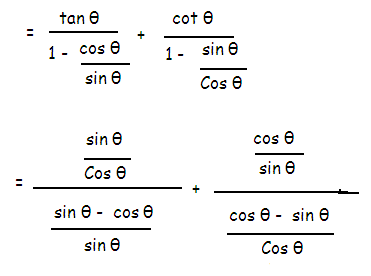
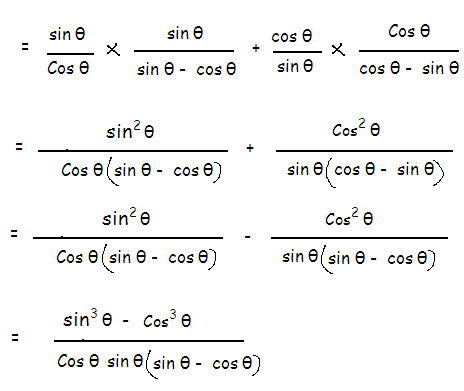
  a³ - b³  =  (a - b)(a² + ab + b²)  

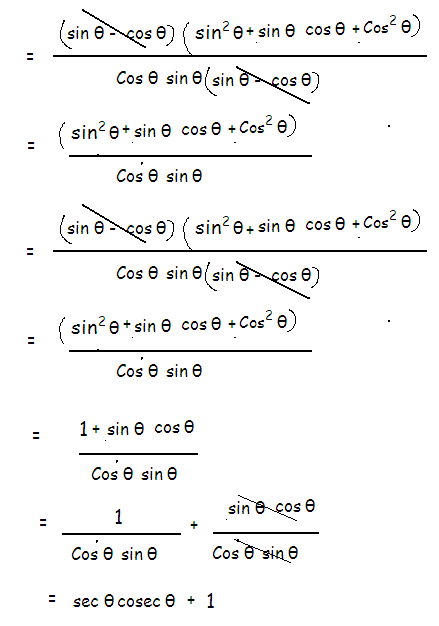
  =  1 + cosecθsecθ

=  R.H.S               

Example 2 :

Prove :

sin(90 - θ)/(1 - tanθ) + cos(90 - θ)/(1 - cotθ)

=  cosθ + sin θ

Solution :

L.H.S :

=  sin(90 - θ)/(1 - tanθ) + cos(90 - θ)/(1 - cotθ)

  =  cosθ/(1 - tanθ) + sinθ/(1 - cotθ)

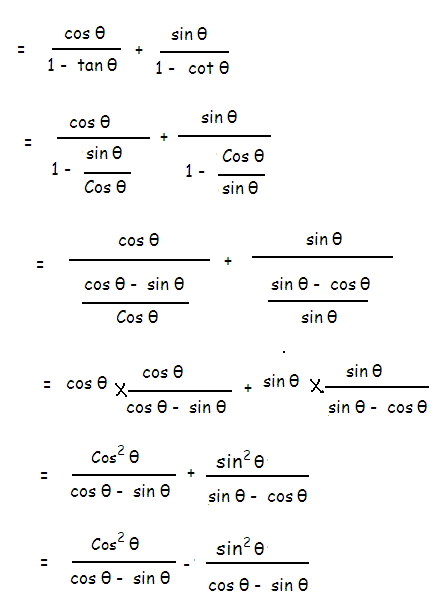
a² - b²  =  (a + b)(a - b)

  =  (cosθ + sinθ)(cosθ - sinθ)/(cosθ - sinθ)

  =  (cosθ + sinθ)

=  R.H.S

Example 3 :

Prove :

[tan(90 - θ)/(cosecθ + 1)] + [(cosecθ + 1)/cotθ)]

=  2 sec θ

Solution :

L.H.S :

  =  [tan(90 - θ)/(cosecθ + 1)] + [(cosecθ + 1)/cotθ)]

we can write tan(90 - θ) as cotθ.

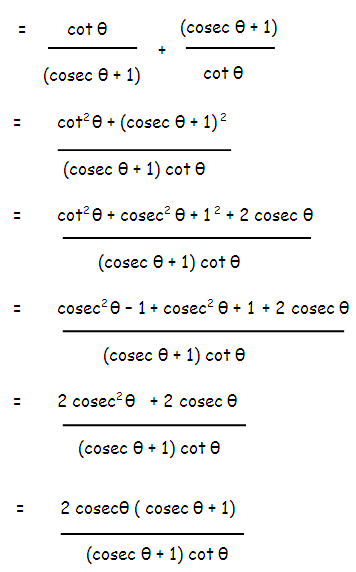
  =  (2/sinθ) / [cosθ/sinθ]

  =  (2/sinθ) x (sinθ/cosθ)

=  2/cosθ

  =  2secθ

=  R.H.S

Example 4 :

Prove :

(cotθ + cosecθ - 1)/(cotθ - cosecθ + 1)

=  cosecθ + cotθ 

Solution :

L.H.S :

=  (cotθ + cosecθ - 1)/(cotθ - cosecθ + 1)

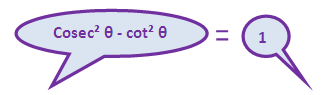
  =  R.H.S

Example 5 :

Prove :

(1 + cotθ - cosecθ)(1 + tanθ + secθ)  =  2

Solution :

L.H.S :

  =  2sinθcosθ/sinθcosθ

  =  2

=  R.H.S

Example 6 :

Prove :

(sinθ - cosθ + 1)/(sinθ + cosθ - 1)  =  1/(secθ - tanθ)

Solution :

L.H.S :

  =  (sinθ - cosθ + 1)/(sinθ + cosθ - 1)

Dividing everything by cos θ.

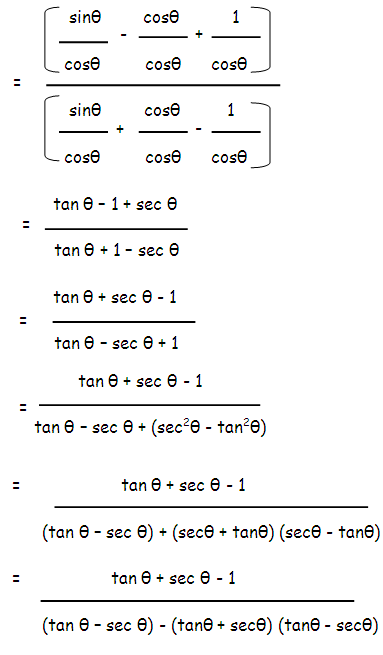
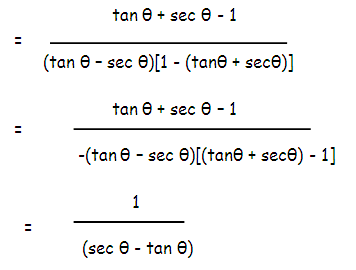
=  R.H.S

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.