USING THE EXTERIOR ANGLE THEOREM

This theorem is Proposition 1.16 in Euclid's Elements, which states that the measure of an exterior angle of a triangle is greater than either of the measures of the remote interior angles. This is a fundamental result in absolute geometry, because its proof does not depend upon the parallel postulate. 

Exterior Angle Theorem

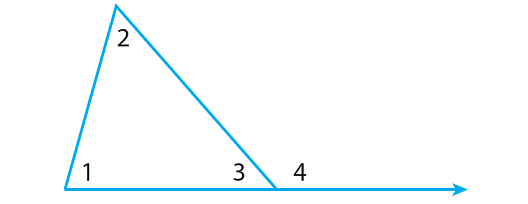
In the above diagram, 

• m∠1, m∠2, and m∠3 are interior angles.
• m∠4 is an exterior angle.
  
• m∠1 and m∠2 are remote interior angles to m∠4.

The theorem states that the measure of an exterior  angle is equal to the sum of its  remote interior angles.

That is, 

m∠1 + m∠2 = m∠4

Solved Problems

Problem 1 :

Find m∠W and m∠X in the triangle given below.

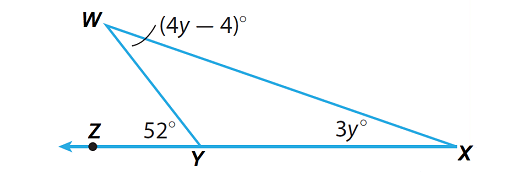
Solution :

Step 1 :

Write the Exterior Angle Theorem as it applies to this triangle.

m∠W + m∠X = m∠WYZ

Step 2 :

Substitute the given angle measures.

(4y - 4)° + 3y° = 52°

Step 3 :

Solve the equation for y.

(4y - 4)° + 3y° = 52°

4y - 4 + 3y = 52

Combine the like terms. 

7y - 4 = 52

Add 4 to both sides.

7y - 4 + 4 = 52 + 4

Simplify.

7y = 56

Divide both sides by 7. 

7y/7 = 56/7

y = 8

Step 4 :

Use the value of y to find m∠W and m∠X.

So, m∠W = 28° and m∠X = 24°.

Problem 2 :

Find m∠A and m∠B in the triangle given below.

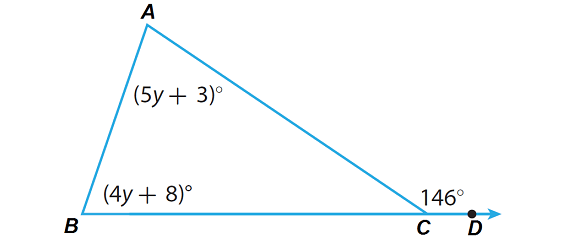
Solution :

Step 1 :

Write the Exterior Angle Theorem as it applies to this triangle.

m∠A + m∠B = m∠C

Step 2 :

Substitute the given angle measures.

(5y + 3)° + (4y + 8)° = 146°

Step 3 :

Solve the equation for y.

(5y + 3)° + (4y + 8)° = 146°

5y + 3 + 4y + 8 = 146

Combine the like terms. 

9y + 11 = 146

Subtract 11 from both sides.

9y + 11 - 11 = 146 - 11

Simplify.

9y = 135

Divide both sides by 9. 

9y/9 = 135/9

y = 15

Step 4 :

Use the value of y to find m∠A and m∠B.

So, m∠A = 78° and m∠B = 68°.

Problem 3 :

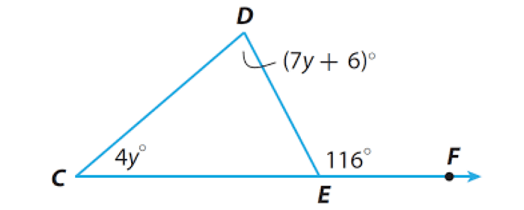
Find m∠C and m∠D in the triangle given below.

Solution :

Step 1 :

Write the Exterior Angle Theorem as it applies to this triangle.

m∠C + m∠D = m∠E

Step 2 :

Substitute the given angle measures.

4y° + (7y + 6)° = 116°

Step 3 :

Solve the equation for y.

4y° + (7y + 6)° = 116°

4y + 7y + 6 = 116

Combine the like terms.

11y + 6 = 116

Subtract 6 from both sides.

11y + 6 - 6 = 116 - 6

Simplify.

11y = 110

Divide both sides by 11. 

11y/11 = 110/11

y = 10

Step 4 :

Use the value of y to find m∠C and m∠D.

So, m∠C = 40° and m∠D = 76°.

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.