WORKSHEET ON SECTION FORMULA

1. Find the point which divides the line segment joining the points (3 , 5) and (8 , 10) internally in the ratio 2 : 3.

2. In what ratio does the point P(-2, 3) divide the line segment joining the points A(-3, 5) and B (4, -9) internally?

3. Find the points of trisection of the line segment joining the points (4, -1) and (-2, -3).

4. Find the coordinates of the point which divides the line segment joining (3, 4) and (–6, 2) in the ratio 3 : 2 externally.

5. Find the points which divide the line segment joining the points (-4, 0) and (0, 6) into four equal parts.

6. Three consecutive vertices of parallelogram are (-2, -1), (1, 0) and (4, 3). Find the fourth vertex.

1. Answer :

Let A(3, 5) and B(8, 10) be the given points.

Let the point P(x, y) divide the line AB internally in the ratio 2 : 3.

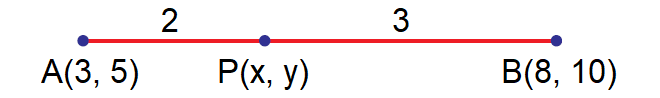
By section formula,

= (mx₂ + nx₁)/(m + n), (my₂ + ny₁)/(m + n)

= [2(8) + 3(3)]/(2 + 3), [2(10) + 3(5)]/(2 + 3)

= (16 + 9)/5, (20 + 15)/5

= 25/5, 35/5

= (5, 7)

So, the required point which divides the line segment is (5, 7).

2. Answer :

Given points are A(-3, 5) and B(4, -9).

Let P (-2, 3) divide AB internally in the ratio l : m.

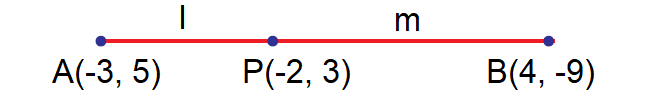
By the section formula,

= (lx₂ + mx₁)/(l + m), (ly₂ + my₁)/(l + m)

= (l(4) + m(-3))/(l + m), (l(-9) + m(5))/(l + m) = (-2, 3)

(4l - 3m)/(l + m), (-9l + 5m)/(l + m) = (-2, 3)

Equating the values of x and y, we get

(4l - 3m)/(l + m) = -2

4l - 3m = -2(l + m)

4l - 3m = -2l - 2m

4l + 2l = -2m + 3m

6l = 1m

l/m = 1/6

l : m = 1 : 6

So, the required ratio is 1 : 6.

3. Answer :

Let A(4, -1) and B(-2, -3) be the given points.

Let P(x, y) and Q(a, b) be the points of trisection of AB so that

AP = PQ = QB

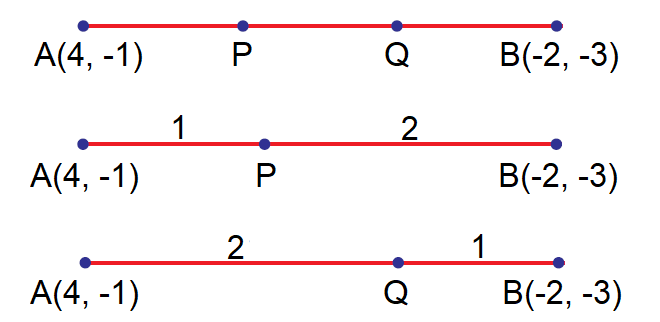
Hence P divides AB internally in the ratio 1 : 2 and Q divides AB internally in the ratio 2 : 1.

Finding the point P :

By the section formula,

= (lx₂ + mx₁)/(l + m), (ly₂ + my₁)/(l + m)

= (1(-2) + 2(4))/(1 + 2), (1(-3) + 2(-1))/(1 + 2)

= (-2 + 8)/3, (-3 - 2)/3

= 6/3, -5/3

= (2, -5/3)

So, the point P is (2, -5/3).

Finding the point Q :

= (2(-2) + 1(4))/(2 + 1), (2(-3) + 1(-1))/(2 + 1)

= (-4 + 4)/3, (-6 - 1)/3

= 0/3, -7/3

= (0, -7/3)

So, the point P is (0, -7/3).

So, the point Q is (0, -7/3).

Note that Q is the midpoint of PB and P is the midpoint of AQ.

4. Answer :

Let A(3, 4) and B(-6, 2) be the given points.

Let the point P(x, y) divide the line AB externally in the ratio 3 : 2.

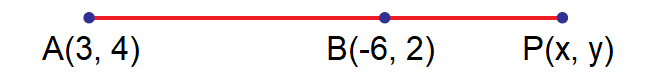
By the section formula,

= (lx₂ - mx₁)/(l - m), (ly₂ - my₁)/(l + m)

= (3(-6) - 2(3))/(3 - 2), (3(2) - 2(4))/(3 - 2)

= (-18 - 6)/1, (6 - 8)/1

= (-24, -2)

5. Answer :

Let A(-4, 0) and B(0, 6) be the given points.

Let P, Q and R be the three points which divide the line AB into four equal parts.

P divides the line segment in the ratio 1 : 3.

By section formula, point P :

= (lx₂ + mx₁)/(l + m), (ly₂ + my₁)/(l + m)

= [1(0) + 3(-4)]/(1 + 3), [1(6) + 3(0)]/(1 + 3)

= (0 - 12)/4, (6 + 0)/4

= (-12/4, 6/4)

= (-3, 3/2)

Q divides the line segment in the ratio 2 : 2.

By section formula, point Q :

= (lx₂ + mx₁)/(l + m), (ly₂ + my₁)/(l + m)

= [2(0) + 2(-4)]/(2 + 2), [2(6) + 2(0)]/(2 + 2)

= (0 - 8)/4, (12 + 0)/4

= (-8/4, 12/4)

= (-2, 3)

By section formula, point R :

= (lx₂ + mx₁)/(l + m), (ly₂ + my₁)/(l + m)

= [3(0) + 1(-4)]/(3 + 1), [3(6) + 1(0)]/(3 + 1)

= (0 - 4)/4, (18 + 0)/4

= (-4/4, 18/4)

= (-1, 9/2)

6. Answer :

Three consecutive vertices of parallelogram are (-2, -1), (1, 0) and (4, 3). Find the fourth vertex.

In parallelogram, the diaognals will bisect each other. Let the vertices of parallelogram be A(-2, -1) B (1, 0) and C (4, 3).

= (lx₂ + mx₁)/(l + m), (ly₂ + my₁)/(l + m)

Let D be the required point (a, b). AC is the diagonal in which E is the point of intersection of the diagonals AC and BD.

Finding the point E :

= (1(4) + 1(-2))/(1 + 1), (1(3) + 1(-1))/(1 + 1)

= (4 - 2)/2, (3 - 1)/2

= (2/2, 2/2)

= (1, 1) -------(1)

Finding the vertex D :

= (1(a) + 1(1))/(1 + 1), (1(b) + 1(0))/(1 + 1)

= (a + 1)/2, (b + 0)/2

= (a + 1/2, b/2) -------(2)

(1) = (2)

So, the fourth vertex is (1, 2).

Kindly mail your feedback to v4formath@gmail.com

We always appreciate your feedback.